Macaulay Duration

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
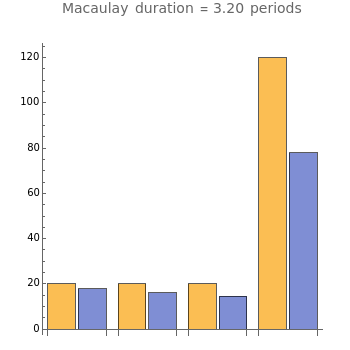
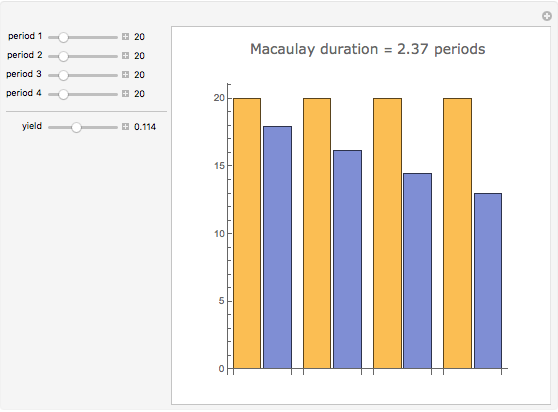
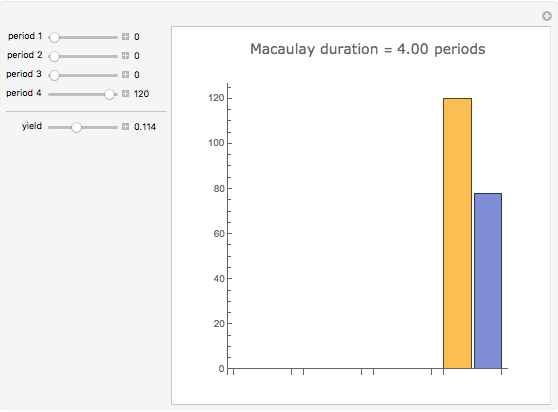
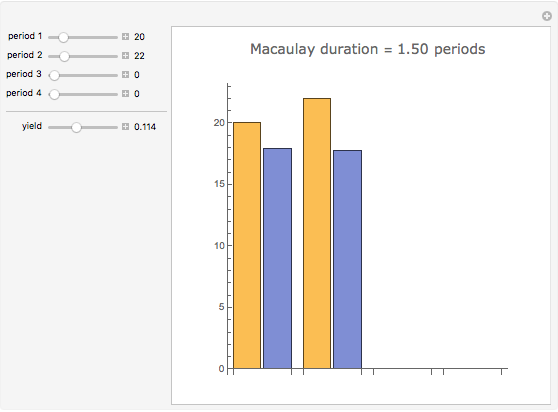
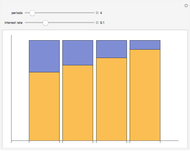
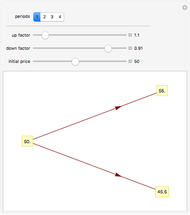
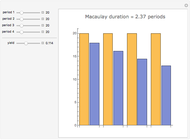
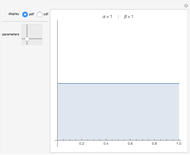
Macaulay duration is a weighted average of the time periods in which cash flows from a security are received. The weight attached to each period is the present value of the cash flow received in that period divided by the present value of the security. If the security pays a single cash flow at maturity, then the duration is equal to the maturity. Otherwise, the duration is less than maturity. The blue bars, controlled by the sliders, represent the raw cash flows, and the purple bars represent their present values. The duration shrinks as the bulk of the cash flows is shifted to the earlier periods.
Contributed by: Fiona Maclachlan (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
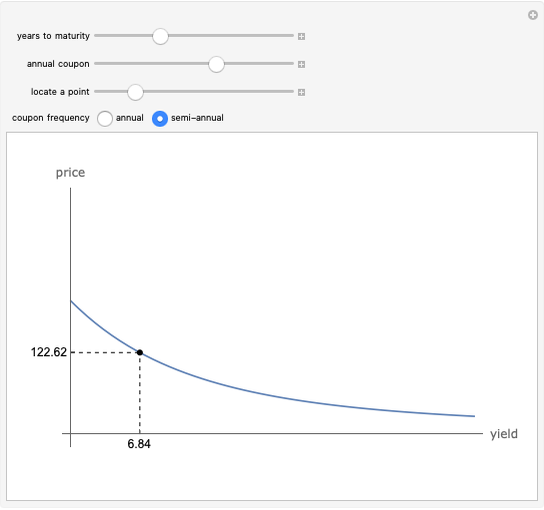
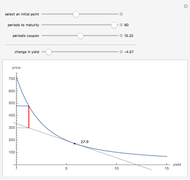
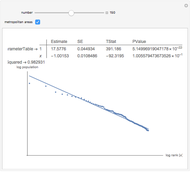
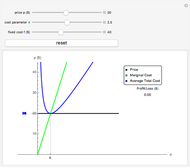
Duration is associated with the slope of the price-yield curve. The absolute value of slope at any point on the price-yield curve is the Macaulay duration times the price of the security, divided by one plus the periodic yield. Modified duration is defined as the Macaulay duration divided by one plus the periodic yield; it used to approximate the percentage change in the price of a security associated with a small change in yield. Dollar duration is modified duration times the price.
Permanent Citation
"Macaulay Duration"
http://demonstrations.wolfram.com/MacaulayDuration/
Wolfram Demonstrations Project
Published: March 7 2011