Normal Modes of a Beaded String

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
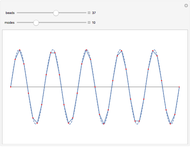
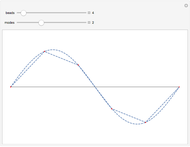
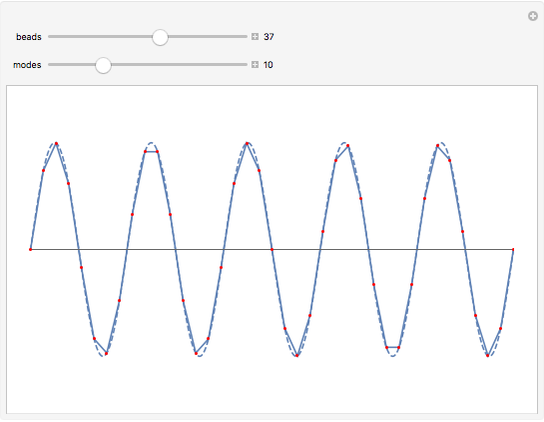
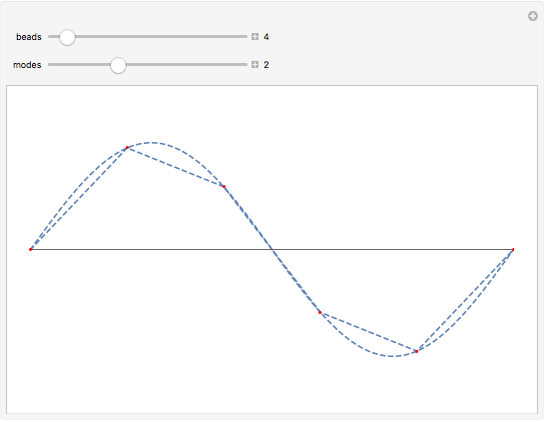
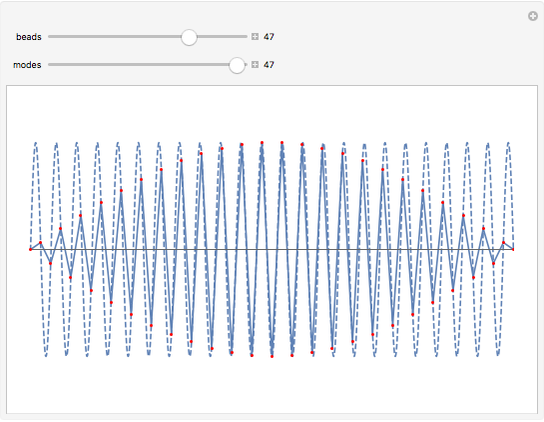
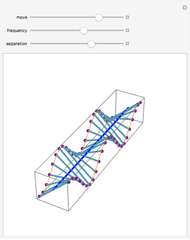
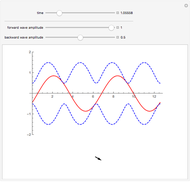
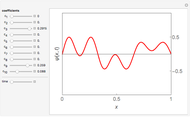
This Demonstration shows the transverse normal modes of oscillation for a string with equally spaced beads. It is assumed that the string consists of a series of massless idealized rectilinear segments that obey Hooke's law with fixed ends. If the string has  beads, then it has
beads, then it has  modes of vibration. The number of times that the string passes through the equilibrium axis in the
modes of vibration. The number of times that the string passes through the equilibrium axis in the  mode is equal to
mode is equal to  , with maximum value
, with maximum value  . When the number of beads is large, the system approaches the behavior of a continuous string.
. When the number of beads is large, the system approaches the behavior of a continuous string.
Contributed by: Enrique Zeleny (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation