Pólya's Urn

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
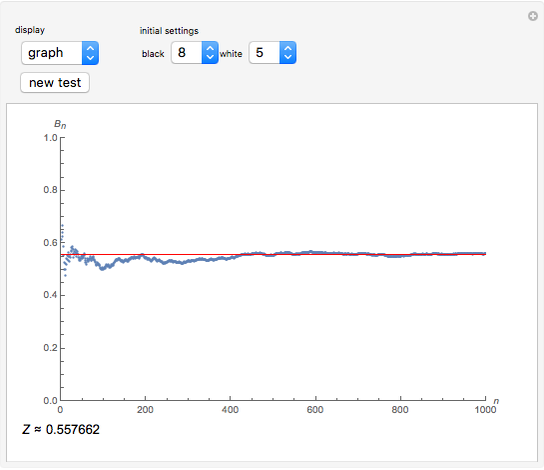
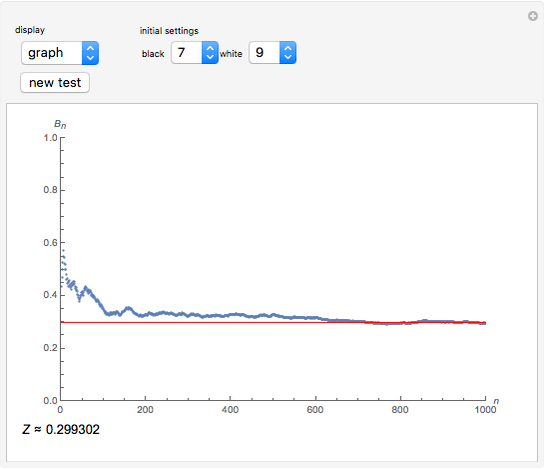
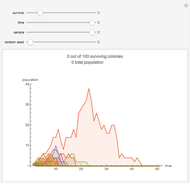
Initially, an urn contains  black marbles and
black marbles and 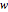 white ones. Every minute, one marble is chosen from the urn at random and replaced, together with another marble of the same color. The proportion of black marbles in the urn after
white ones. Every minute, one marble is chosen from the urn at random and replaced, together with another marble of the same color. The proportion of black marbles in the urn after  minutes is the random variable
minutes is the random variable 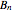 . The sequence of random variables is a martingale, and thus converges almost surely to a limit random variable
. The sequence of random variables is a martingale, and thus converges almost surely to a limit random variable 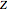 . This limit random variable
. This limit random variable 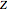 has the beta distribution
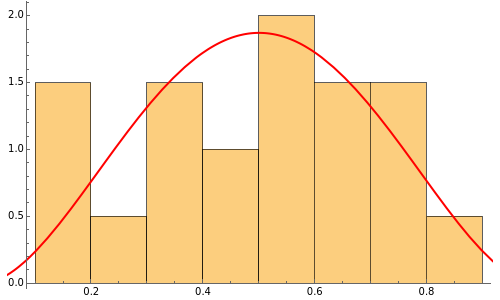
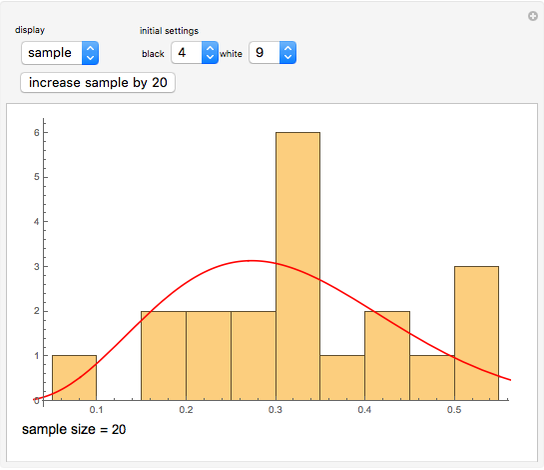
has the beta distribution 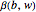 . This Demonstration enables you either to plot the graph of
. This Demonstration enables you either to plot the graph of 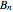 against
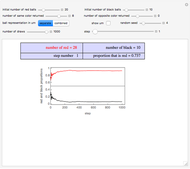
against  for 1000 minutes, demonstrating the convergence, or to take samples of the random variable
for 1000 minutes, demonstrating the convergence, or to take samples of the random variable 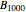 , comparing histograms of the resulting data with the PDF of the beta distribution.
, comparing histograms of the resulting data with the PDF of the beta distribution.
Contributed by: Mark Hennings (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
G. R. Grimmett and D. R. Stirzaker, Probability and Random Processes, Oxford: Oxford University Press, 1982.
"Urn problem," Wikipedia.
Permanent Citation
"Pólya's Urn"
http://demonstrations.wolfram.com/PolyasUrn/
Wolfram Demonstrations Project
Published: March 7 2011