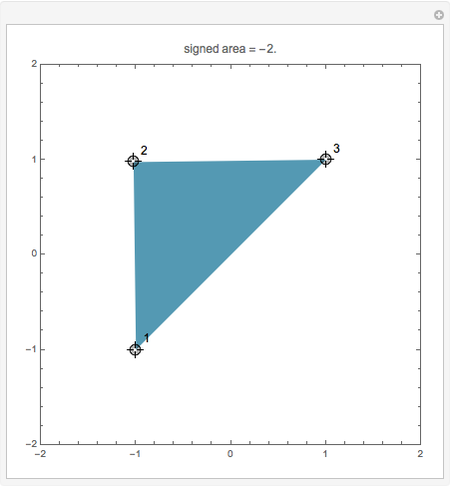
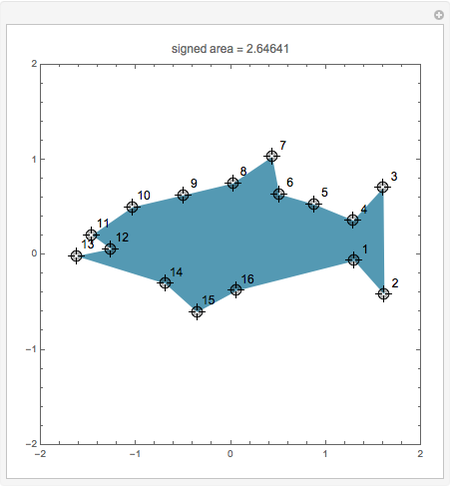
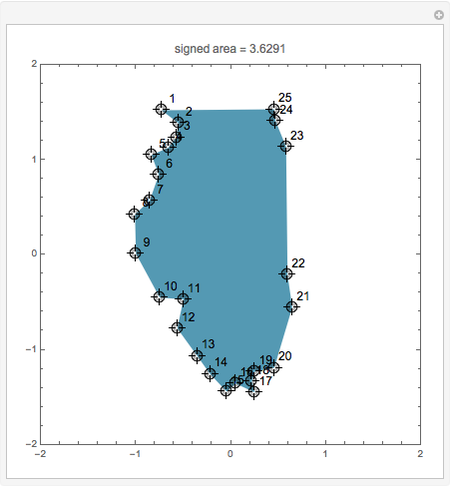
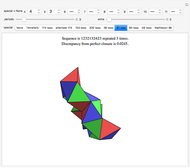
Signed Area of a Polygon

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
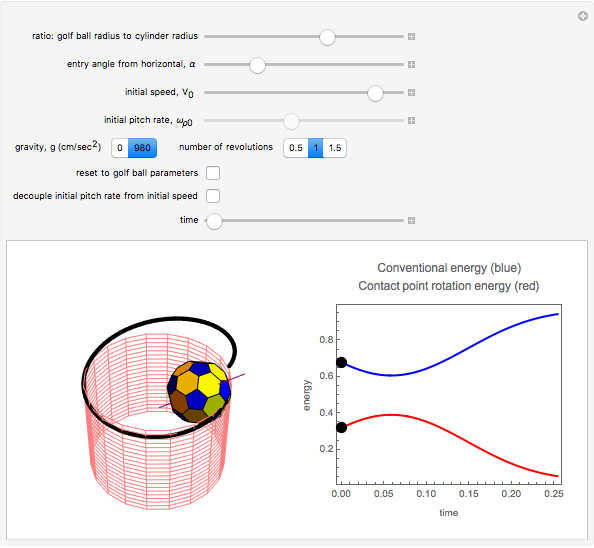
The signed area of a polygon with vertices 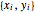 numbered
numbered 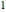 through
through  can be calculated exactly by the formula
can be calculated exactly by the formula 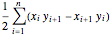 , where
, where 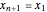 and
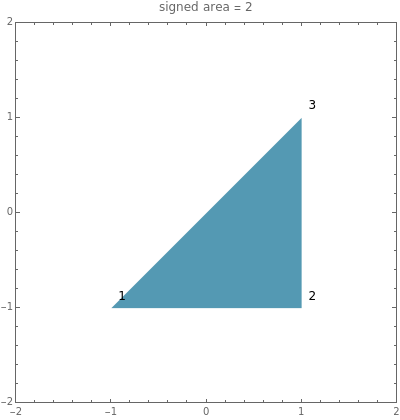
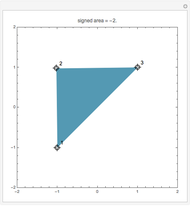
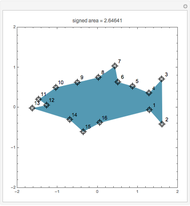
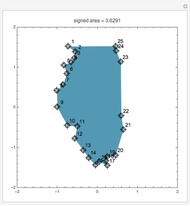
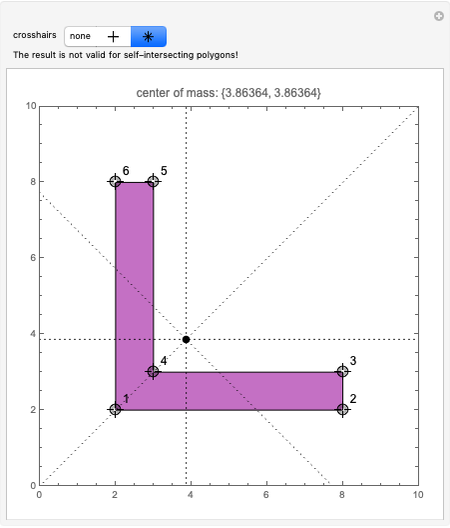
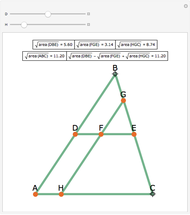
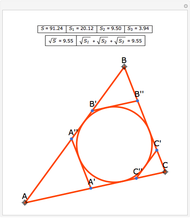
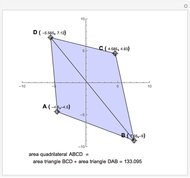
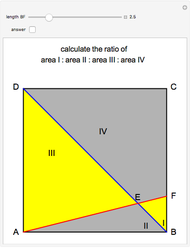
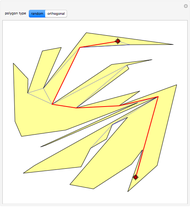
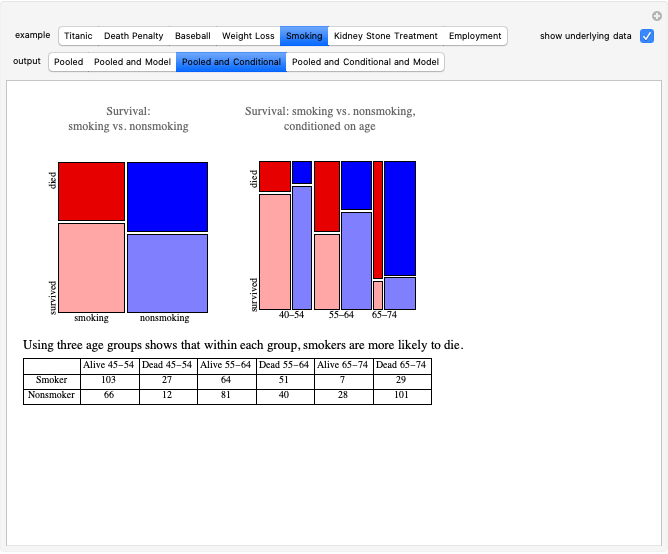
and 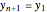 . If the polygon is simple (non-intersecting sides), with the vertices numbered in a counterclockwise direction, the signed area is the area. This formula is surprisingly useful in surveying, architecture, and many other applications.
. If the polygon is simple (non-intersecting sides), with the vertices numbered in a counterclockwise direction, the signed area is the area. This formula is surprisingly useful in surveying, architecture, and many other applications.
Contributed by: Bruce Atwood (Beloit College) and Stan Wagon (Macalester College) (March 2011)
After work by: Stan Wagon
Open content licensed under CC BY-NC-SA
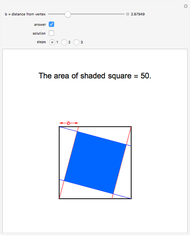
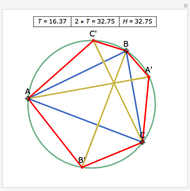
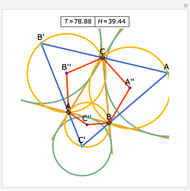
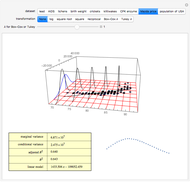
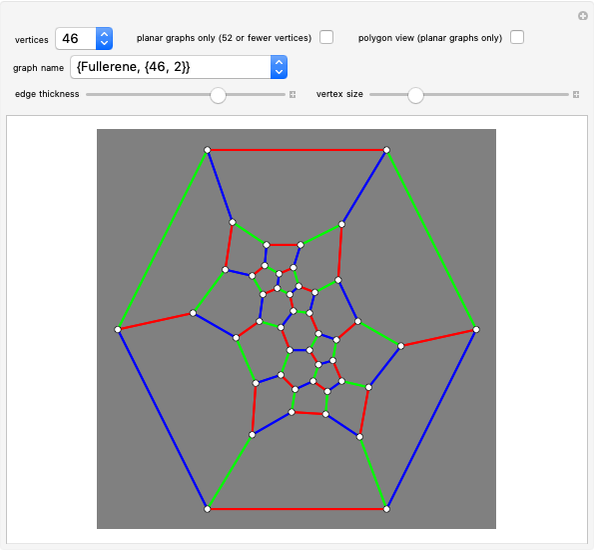
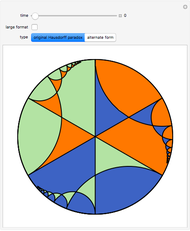
Snapshots
Details
The formula for the area of a simple polygon can be elegantly derived using Green's theorem and extended to moments of the region. S. F. Bockman, "Generalizing the Formula for Areas of Polygons to Moments," Amer. Math. Monthly, 96(2), 1989 pp. 131-132.
For more information, see: S. Wagon, Mathematica in Action, 2nd ed., New York: Springer, 1999.
Permanent Citation
"Signed Area of a Polygon"
http://demonstrations.wolfram.com/SignedAreaOfAPolygon/
Wolfram Demonstrations Project
Published: March 7 2011