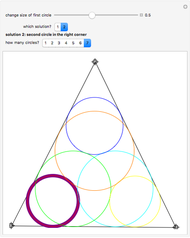
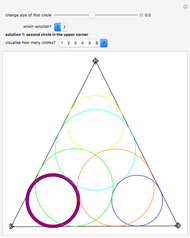
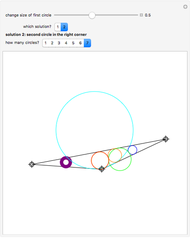
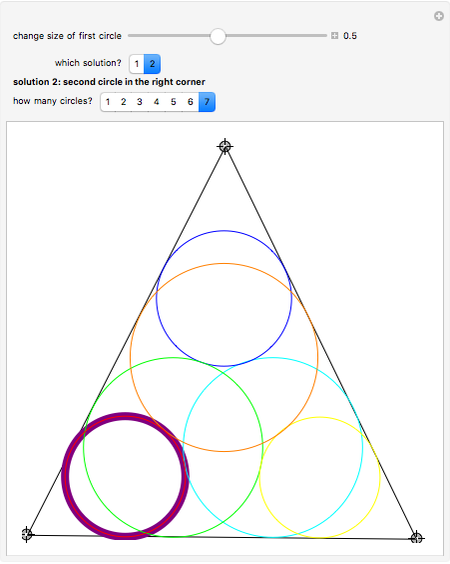
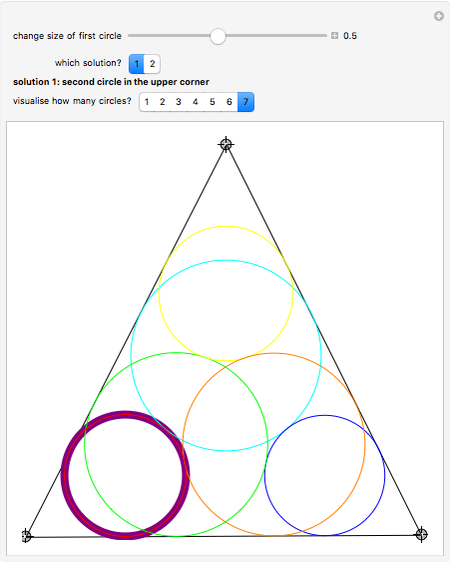
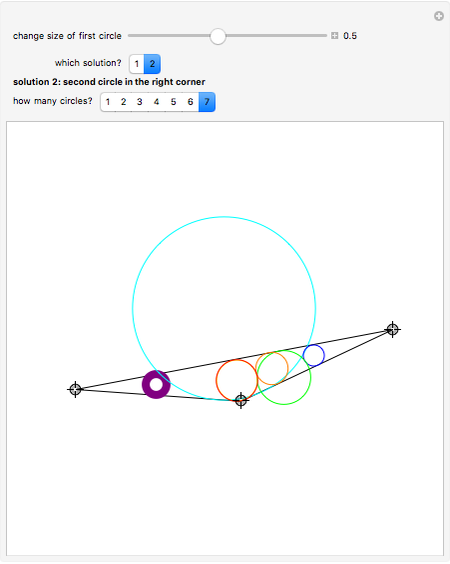
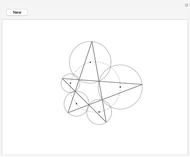
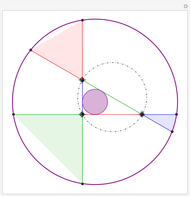
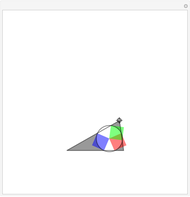
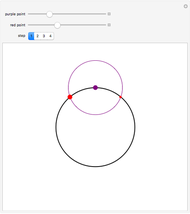
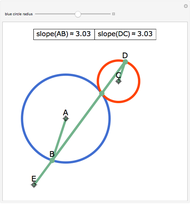
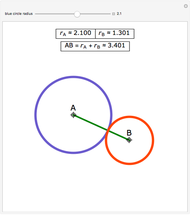
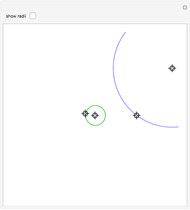
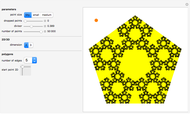
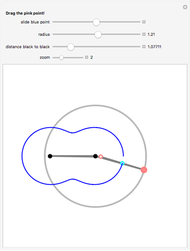
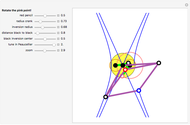
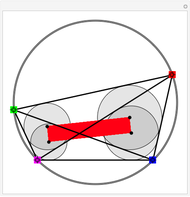
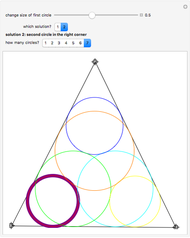
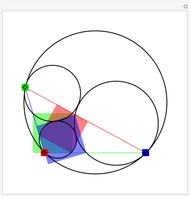
Take a triangle. For each circle tangent to two triangle sides, draw a circle tangent to it and to two sides of the triangle. Iterating the construction you get a circle tangent to the first circle after six steps.
This Demonstration is a sort of visual proof. Choose the triangle with the locators and choose the size of the circle you start with. Choosing solution 1 or 2 selects the corner for the second circle. Increase the number of circles. Finally the seventh circle will appear again as the first one.
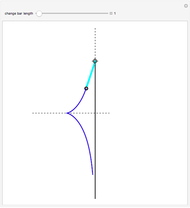
The construction of a circle tangent to two intersecting lines and another circle is not trivial. One has to find the intersection of the bisector and the parabola with focus the center of the first circle. So it seems there is no geometric construction. Therefore we present the analytical construction here. This might explain why this quite instructive theorem was found so late.
C. J. A. Evelyn, G. B. Money-Coutts, J. A. Tyrrell, The Seven Circles Theorem and Other New Theorems, London: Stacey International, 1974 pp. 31–42. (This booklet seems hard to find according to Seven Circles Theorem.)
![[Snapshot]](HTMLImages/index.en/thumbnail_1.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_2.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_3.gif)
Embed Interactive Demonstration 
Browse all topics
