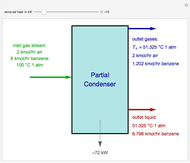
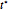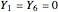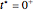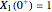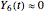Solute Extraction Cascade with Solvent Recycle

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
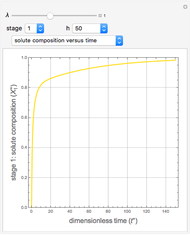
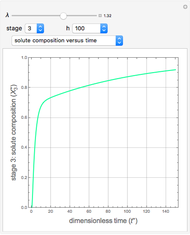
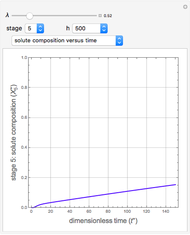
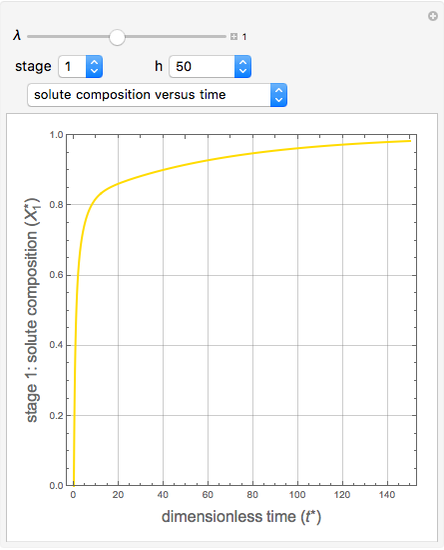
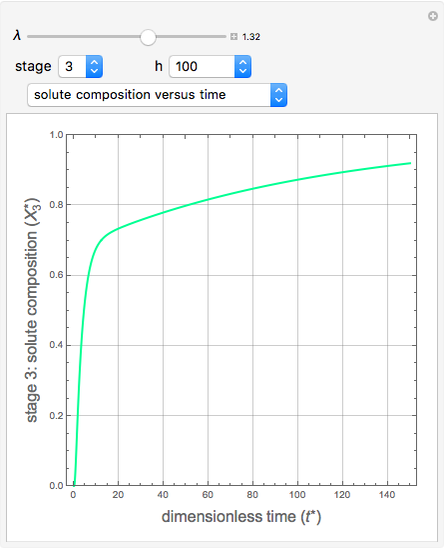
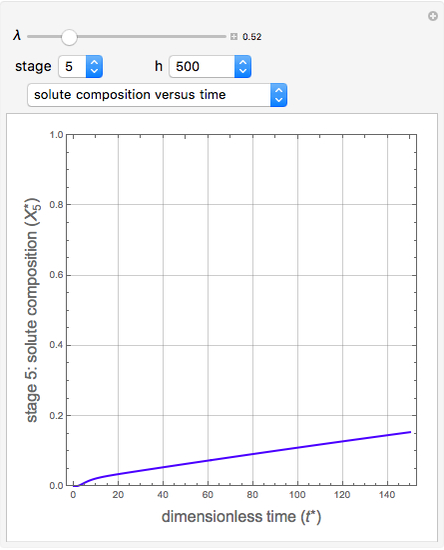
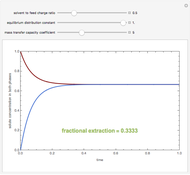
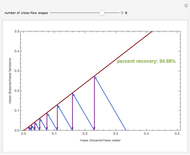
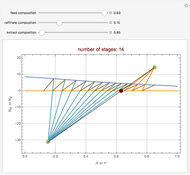
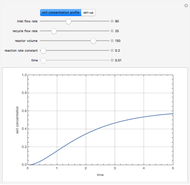
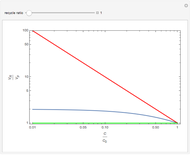
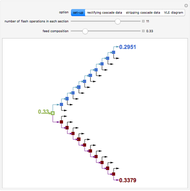
This Demonstration analyzes a solute extraction process. A raffinate stream with volumetric flow 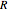 containing an unwanted solute enters a five-stage extraction cascade that is connected to a tank holding the extraction solvent. The solvent container is modeled as a perfectly mixed tank such that the solute composition leaving the tank at any time is the same as the composition in the tank at that time. This problem was solved analytically by Ugo Lelli [1]. Here a numerical solution using Mathematica is given that lets you examine how two key parameters for the extraction process influence the system performance. You can see the effect of varying different values of the solvent container residence time
containing an unwanted solute enters a five-stage extraction cascade that is connected to a tank holding the extraction solvent. The solvent container is modeled as a perfectly mixed tank such that the solute composition leaving the tank at any time is the same as the composition in the tank at that time. This problem was solved analytically by Ugo Lelli [1]. Here a numerical solution using Mathematica is given that lets you examine how two key parameters for the extraction process influence the system performance. You can see the effect of varying different values of the solvent container residence time  (expressed as a dimensionless parameter
(expressed as a dimensionless parameter  ) and the dimensionless parameter
) and the dimensionless parameter 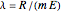 (related to the volumetric flow rates
(related to the volumetric flow rates 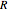 and
and 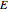 in the raffinate and extract phases).
in the raffinate and extract phases).
Contributed by: Housam Binous, Brian G. Higgins, and Ahmed Bellagi (January 2013)
Open content licensed under CC BY-NC-SA
Snapshots
Details
A material balance over the solute phase for an arbitrary stage  is:
is:
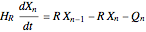 ,
, 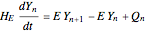 ,
,
where 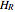 and
and 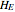 are the stage holdup volumes expressed in
are the stage holdup volumes expressed in  for the raffinate and extract phases;
for the raffinate and extract phases; 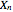 and
and  are the solute mass fractions in the raffinate and extract phases leaving the
are the solute mass fractions in the raffinate and extract phases leaving the  stage, respectively;
stage, respectively; 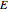 and
and 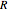 are the volumetric flow rates for the extract and raffinate phases expressed in
are the volumetric flow rates for the extract and raffinate phases expressed in 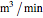 ; and
; and 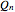 is the volumetric rate of solute that is transferred between phases at the
is the volumetric rate of solute that is transferred between phases at the  stage. If equilibrium is established at each stage so that
stage. If equilibrium is established at each stage so that 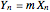 , the solute material balances can be combined to give a single equation for the change in mass fraction of solute in the raffinate at the
, the solute material balances can be combined to give a single equation for the change in mass fraction of solute in the raffinate at the  stage:
stage:
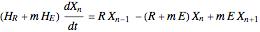 .
.
For computational purposes it is convenient to work with the dimensionless form of this equation, which for the  stage is given by:
stage is given by:
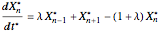 ,
,
where 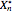 is the dimensionless solute composition given by
is the dimensionless solute composition given by  . For the dilute system approximation to hold, we require that
. For the dilute system approximation to hold, we require that 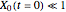 .
.
The specified dimensionless inlet composition of the raffinate stream for 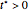 is
is 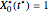 . The dimensionless time is defined as
. The dimensionless time is defined as 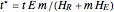 , while the flow rate ratio parameter is given by
, while the flow rate ratio parameter is given by 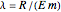 . Finally, the dynamic behavior of the solvent tank is governed by:
. Finally, the dynamic behavior of the solvent tank is governed by:
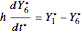 ,
, 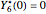 , or equivalently,
, or equivalently, 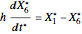 ,
, 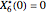 ,
,
where the dimensionless parameter 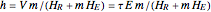 and
and 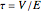 is the residence time of the solvent tank with volume
is the residence time of the solvent tank with volume 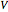 .
.
References
[1] U. Lelli, Annali di Chimica, 56, 1966 pp. 113–125.
[2] J. Ingham, I. J. Dunn, E. Heinzle, and J. E. Prenosil, Chemical Engineering Dynamics, 2nd ed., Weinheim, Germany: Wiley-VCH, 2000.
Permanent Citation