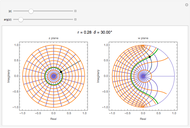
Transformation of s-Reflection Coefficient between Normal and Oblique Incidence

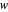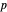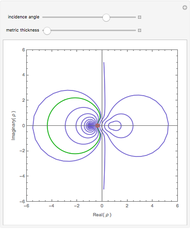
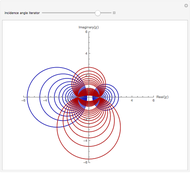
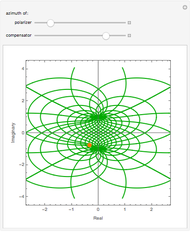
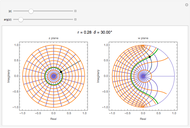
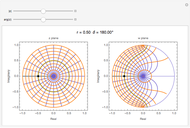
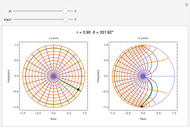
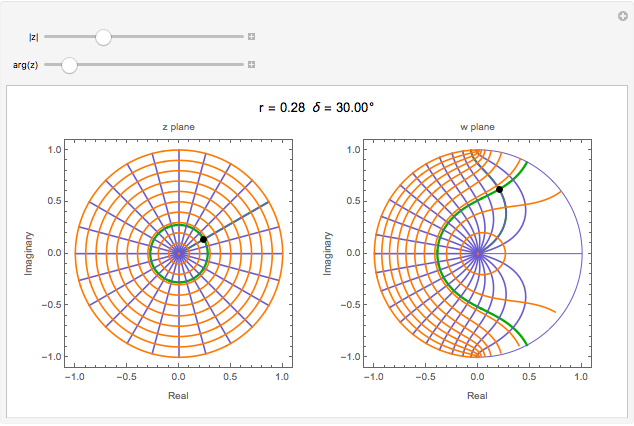
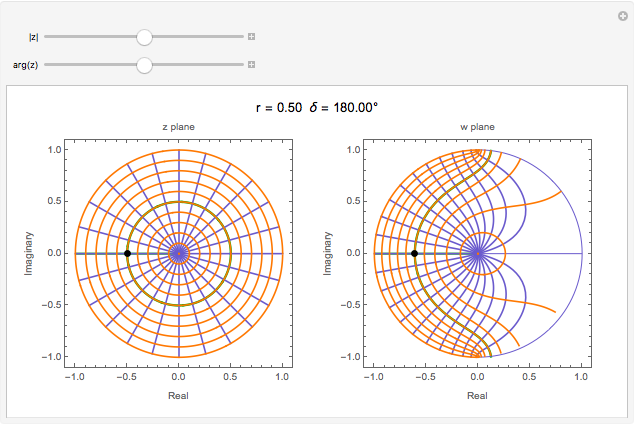
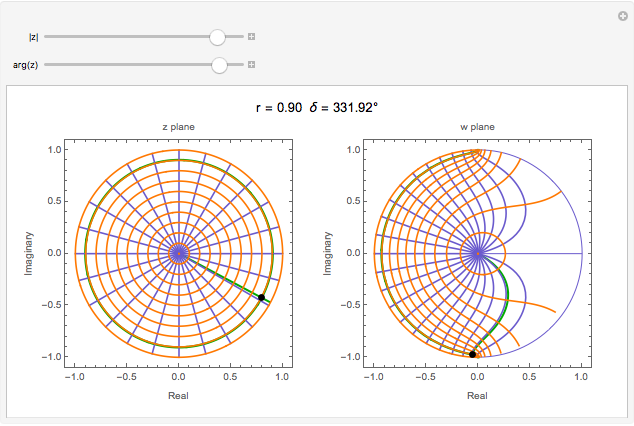
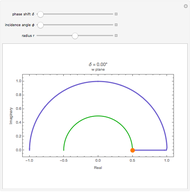
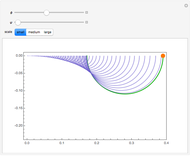
If denotes an interface Fresnel reflection or transmission coefficient for - or -polarized light at an oblique angle of incidence , and denotes the same coefficient at normal incidence, then it can be shown that w is an analytic function of that depends parametrically on the angle of incidence . The mapping between the complex and 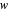planes is illustrated here by one of the Fresnel coefficients (for s reflection) at normal incidence and one oblique angle of incidence (45°). Here 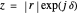, where 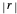and are the normal-incidence amplitude reflectance and phase shift.

This figure shows that the orthogonal (polar) set of straight lines and circles through and around the origin in the plane is mapped onto orthogonal sets of curves in the plane.