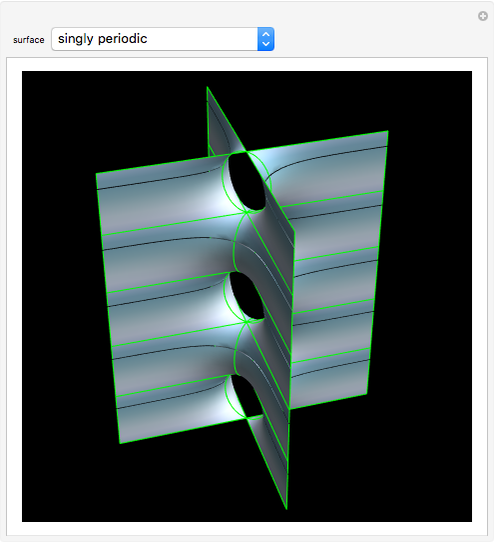
Delaunay Nodoids

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
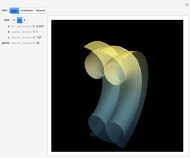
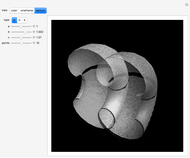
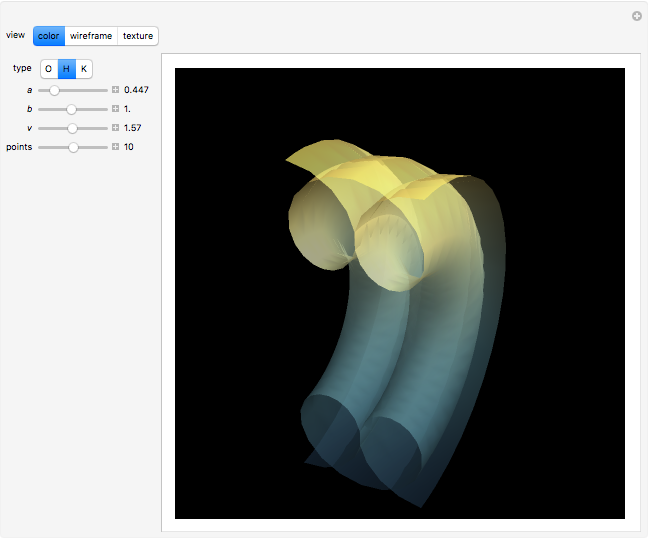
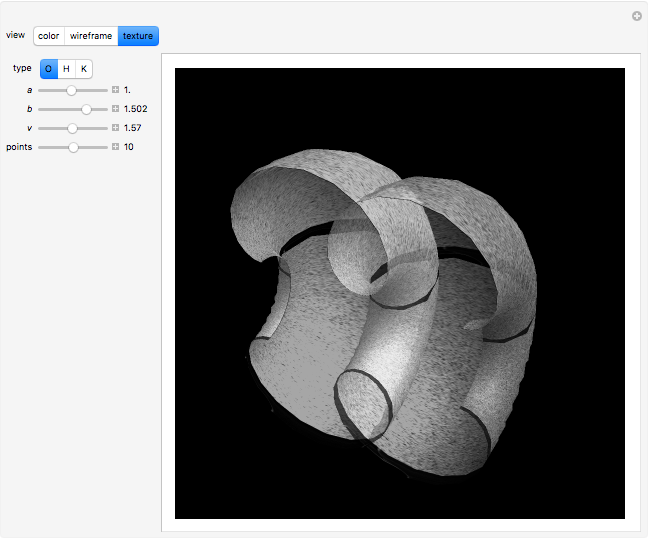
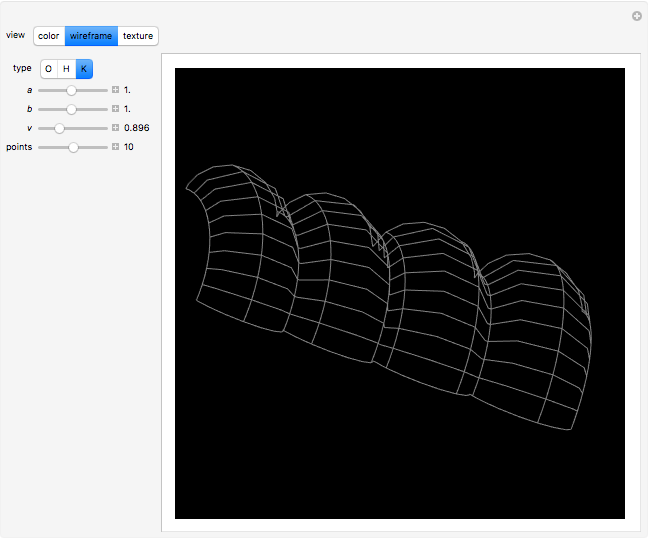
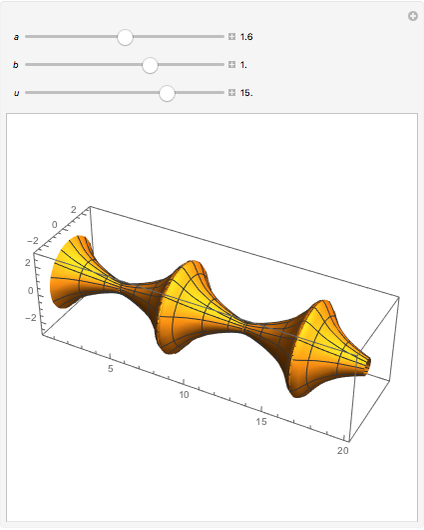
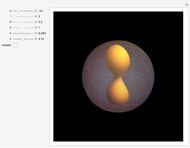
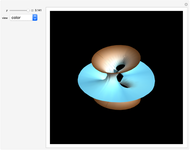
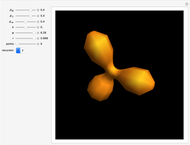
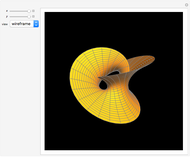
The Delaunay nodoids [1] belong to a family of surfaces of revolution, translationally periodic with constant mean curvature (CMC). The other surfaces in the class are the sphere, the cylinder, the catenoid, and the unduloid [2–4], whose generating curves of revolution come from roulettes of conic sections. To obtain the nodoid, start with the locus of a focus of a hyperbola that rolls without slipping along a straight line, generating a curve known as the nodary; its revolution about the axis of rotation yields the surface.
[more]
Contributed by: Enrique Zeleny (July 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The parametric equations of the nodoid are
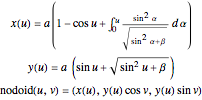
with 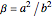 where
where  and
and  are parameters, with mean curvature
are parameters, with mean curvature 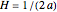 . The different types are parallel surfaces. In type "H", the signs of the first sine and cosine in the expressions for
. The different types are parallel surfaces. In type "H", the signs of the first sine and cosine in the expressions for  and
and 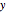 are reversed and in type "K", these terms are dropped.
are reversed and in type "K", these terms are dropped.
References
[1] C. Delaunay, "Sur la Surface de Révolution Dont la Courbure Moyenne Est Constante," Journal de Mathématiques Pures et Appliquées, 1(6), 1841 pp. 309–320.
[2] "Delaunay Surfaces." Geometrie Werkstatt. (Jun 25, 2014) www.math.uni-tuebingen.de/user/nick/gallery/Delaunay.html.
[3] I. M. Mladenov, "Delaunay Surfaces Revisited," Comptes Rendus de l'Academie Bulgare des Sciences, 55, 2002 pp. 5–19. articles.adsabs.harvard.edu//full/2002CRABS..55e..19M/E000023.000.html.
[4] W. Rossman, "The First Bifurcation Point for Delaunay Nodoids," Experimental Mathematics, 14(3), 2005 pp. 331–342. www.emis.de/journals/EM/expmath/volumes/14/14.3/Rossman.pdf.
[5] E. Bendito, M. J. Bowick, A. Medina, and Z. Yao, "Crystalline Particle Packings on Constant Mean Curvature (Delaunay) Surfaces", Physical Review E, 88, 2013 pp. 012405. journals.aps.org/pre/abstract/10.1103/PhysRevE.88.012405.
Permanent Citation