Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

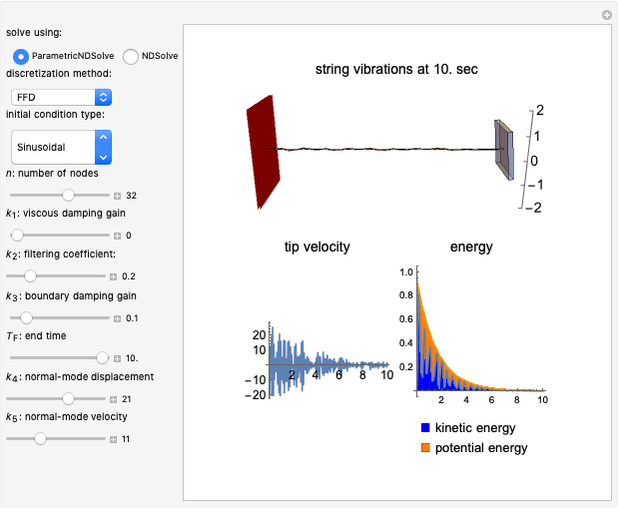
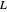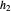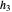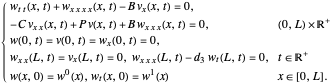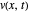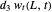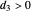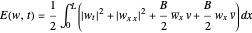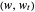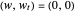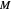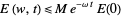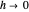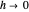Feedback Sensor Design for a Cantilevered Three-Layer Sandwich Beam

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

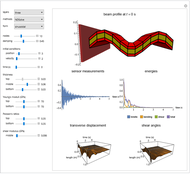
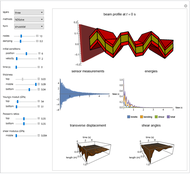
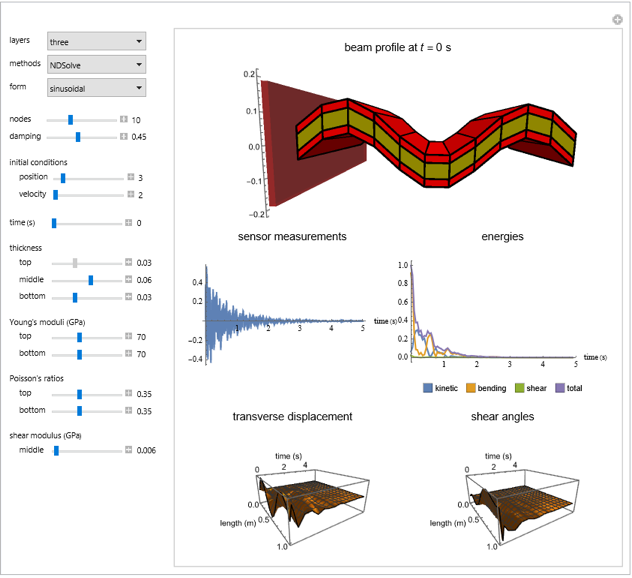
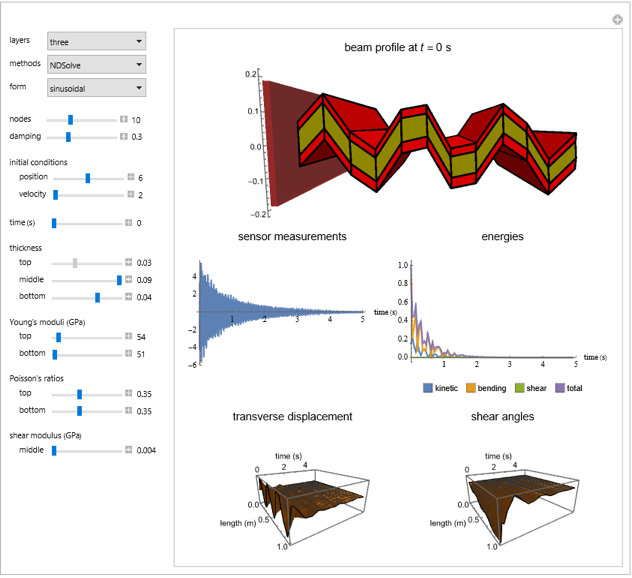
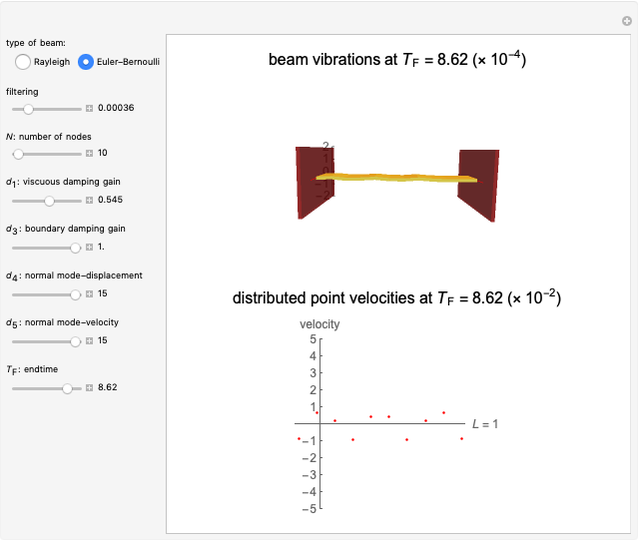
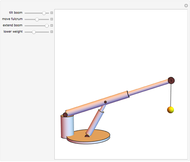
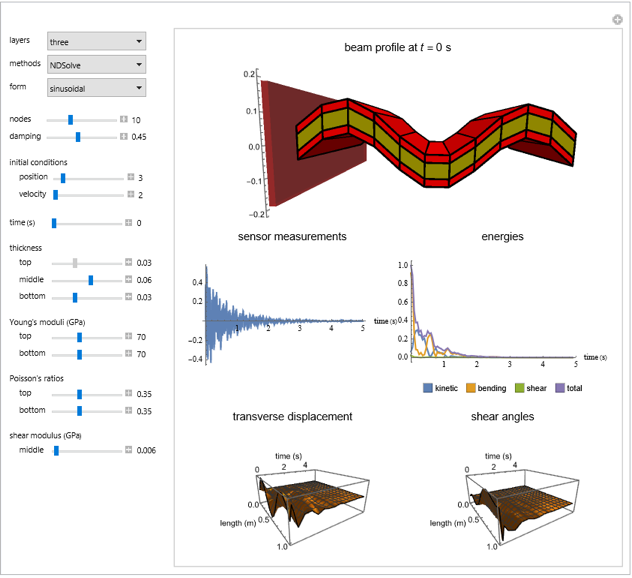
This Demonstration considers boundary-controlled vibrations on a cantilevered three-layer sandwich beam, consisting of a viscoelastic middle layer sandwiched (and perfectly bonded) between two outer elastic/piezoelectric layers. Outer and middle layers are modeled by the Euler–Bernoulli and the Mindlin–Timoshenko small displacement assumptions, respectively [1]. The outer layers are resistive to transverse shear of the filaments, while the middle viscoelastic layer allows transverse shear. Therefore, the only coupling across the layers is due to the shear of the middle viscoelastic layer. The layers, even though bonded, can move longitudinally during the motion. The sensor data with the measurements of the tip velocity of the beam is fed back to the actuator controlling the transverse shear on the boundary. The suppression of vibrations is achieved with this setup of the closed-loop system.

[more]

Let and , , be the length and thicknesses of each layer from the bottom to the top, respectively. The equations of motion follow the infamous Mead–Marcus–type beam equations, which are described by the following partial differential equation (PDE):

(1)

Here represents the overall bending of the beam and represents the shear angle of the middle layer. , and represent physical constants determining shear modulus, Young's moduli, Poisson's ratios and layer thicknesses. Note that the with refers to the boundary damping measurements fed back to the closed-loop dynamics. For more details about constants and the modeling assumptions, refer to [1–3].

This model is shown to maintain the uniform observability property with the measured state. Incidentally, the proof is based on the discrete multipliers, since the eigenvalues can be found explicitly. This leads to energy estimates more difficult to obtain than the ones of the hinged boundary conditions [3, 4]. Finally, the energy

of solutions of (1) are known to decay to equilibrium state exponentially fast; that is, there exists , such that ; see also [1] and the references therein.

A recently introduced approximation technique known as Order-Reduced Finite Differences [5] is applied to (1). This approximation technique reduces the model (1) to a first-order model and it eliminates the need of the filtering of the spurious high-frequency eigenvalues [6]. The resulting reduced model is shown to be uniformly observable as [3, 4]. This implies that the reduced model retains exponential stability as .

[less]

Contributed by: Ahmet Kaan Aydin, Matthew Poynter and Ahmet Özkan Özer (January 2023)
Open content licensed under CC BY-NC-SA

Snapshots

Details

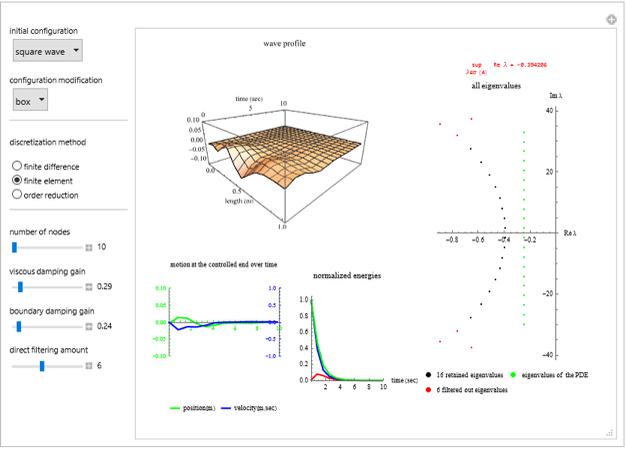
The PDE model (1) is approximated by the so-called Order-Reduced Finite Differences. Let the mesh parameter be 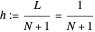, where 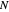is the number of nodes in the uniform discretization in space variable , and 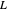is taken to be 1 for simplicity. Now, the nodes of the discretization are

.

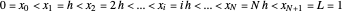Due to the second order boundary conditions, two fictitious points , 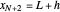are also introduced.

Letting 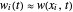, describes the bending and shear angle on the beam, respectively; the reduced model of (1) is proposed as the following:

(2)

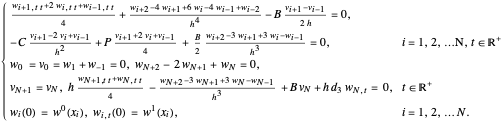
This model is shown to be uniformly observable with the sensor measurement 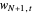at the tip of the beam, and therefore, the solutions of (2) are exponentially stable, mimicking (1).

The initial conditions can be chosen in the form of sinusoidal, pinch, box, square wave, triangular wave or sawtooth wave with the magnitude of 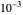. Materials constants and thicknesses of each layer can be determined individually. In order to better visualize the transverse displacements in the beam profile plot, and are scaled up. The single-layer beam model (Euler–Bernoulli) is also shown, which is equivalent to (2) with 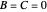[2]. The same filtering method is successfully implemented for the boundary-damped wave equation [3].

This material is based upon work supported by the National Science Foundation under Cooperative Agreement No. 1849213. Any opinions, findings and conclusions or recommendations expressed in this material are those of the author(s) and do not necessarily reflect the views of the National Science Foundation.

References

[1] A. Ö. Özer, "Dynamic and Electrostatic Modeling for a Piezoelectric Smart Composite and Related Stabilization Results," Evolution Equations and Control Theory, 7(4), 2018 pp. 639–668. doi:10.3934/eect.2018031.

[2] S. W. Hansen, "Several Related Models for Multilayer Sandwich Plates," Mathematical Models and Methods in Applied Sciences, 14(8), 2004 pp. 1103–1132. doi:10.1142/S0218202504003568.

[3] A. Ö. Özer and A. K. Aydin, "A Novel Sensor Design for a Cantilevered Mead–Marcus Type Sandwich Beam Model by the Order-Reduction Technique," to be submitted.

[4] A. K. Aydin, "Robust Sensor Design for the Novel Reduced Model of the Mead–Marcus Sandwich Beam Equation," M.Sc. thesis, Department of Mathematics, Western Kentucky University, Bowling Green, KY, 2022. digitalcommons.wku.edu/theses/3603.

[5] J. Liu and B. Z. Guo, "A Novel Semi-discrete Scheme Preserving Uniformly Exponential Stability for an Euler–Bernoulli Beam," Systems & Control Letters, 134(12), 2019 104518. doi:10.1016/j.sysconle.2019.104518.

[6] I. F. Bugariu, S. Micu and I. Roventa, "Approximation of the Controls for the Beam Equation with Vanishing Viscosity," Mathematics of Computation, 85, 2016 pp. 2259–2303. doi:10.1090/mcom/3064.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send