Folding a  Sheet of Labeled Stamps
Sheet of Labeled Stamps

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
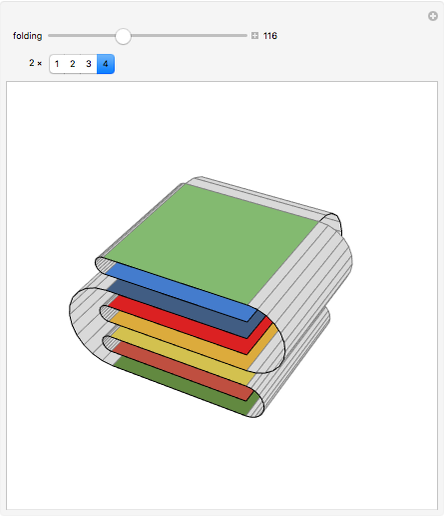
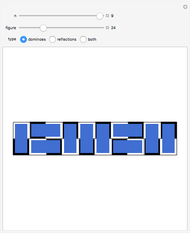
This Demonstration illustrates the different ways to fold a  sheet of distinct stamps into a
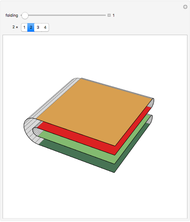
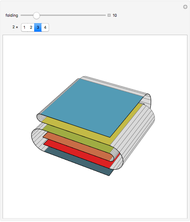
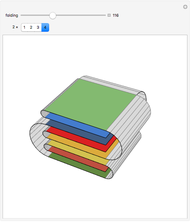
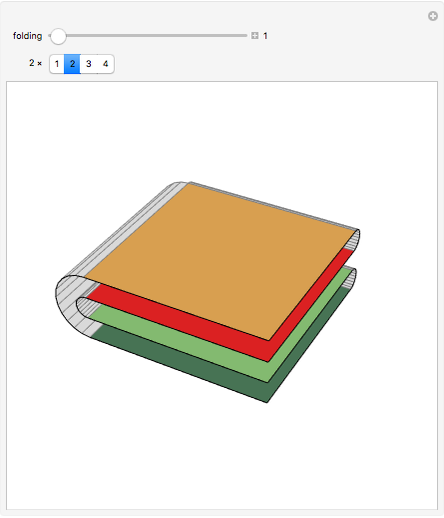
sheet of distinct stamps into a  stack. In each figure, the colored squares represent the stamps and the gray half-cylinders represent the perforation between adjacent stamps.
stack. In each figure, the colored squares represent the stamps and the gray half-cylinders represent the perforation between adjacent stamps.
Contributed by: Robert Dickau (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
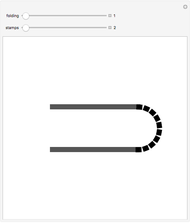
Snapshot 1: for a  sheet of stamps, all eight foldings have the same shape; it is clear that the number of foldings is less than
sheet of stamps, all eight foldings have the same shape; it is clear that the number of foldings is less than  , since two stamps initially diagonal from each other cannot be adjacent in the final stack
, since two stamps initially diagonal from each other cannot be adjacent in the final stack
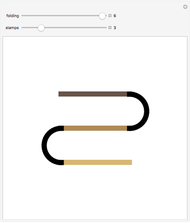
Snapshots 2, 3: for larger sheets, the counts are further diminished by rejecting foldings in which initial vertical or horizontal perforations would intersect
It is somewhat surprising that no general formula is known for counting the number of foldings of an  sheet of stamps. The problem is sometimes called the map-folding problem.
sheet of stamps. The problem is sometimes called the map-folding problem.
M. Gardner, "The Combinatorics of Paper Folding," in Wheels, Life and Other Mathematical Amusements, New York: W. H. Freeman, 1983 pp. 60–61.
Permanent Citation