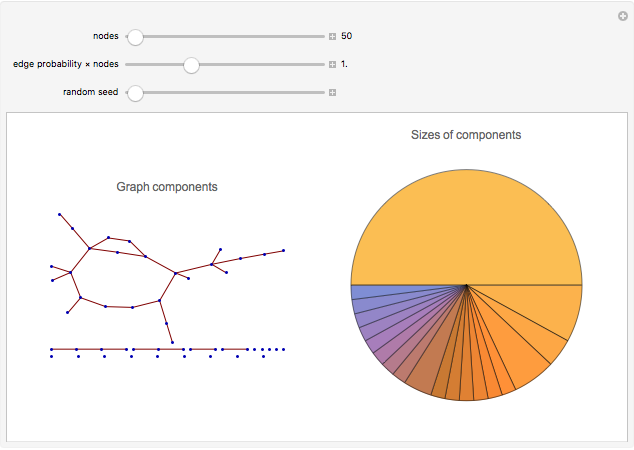
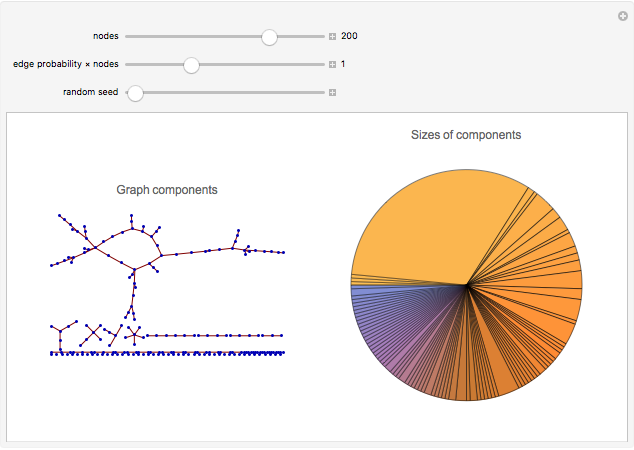
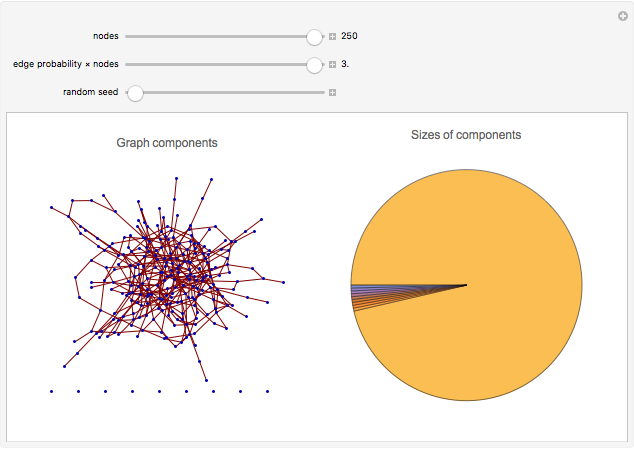
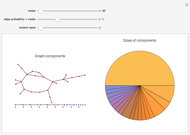
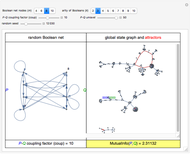
A random graph is a graph with nodes where the probability of finding an edge between two nodes is . When tends to a constant (as grows), the graph will almost surely contain a "giant" connected component, absorbing a considerably large fraction of the nodes. This phenomenon is often mentioned as an example of emergence in random graph behavior.
The emergence of a giant connected component plays a crucial role in the theory of autocatalytic chemical networks, which attempts to explain the appearance of life in the universe ("abiogenesis") as a self-organization phenomenon occurring within chemical systems. The idea was first proposed by Stuart Kauffman in 1995.
![[Snapshot]](HTMLImages/index.en/thumbnail_1.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_2.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_3.gif)
Embed Interactive Demonstration 
Browse all topics
