Global Minimum of a Non-Convex Function

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
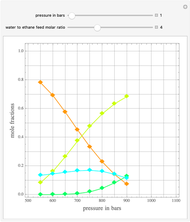
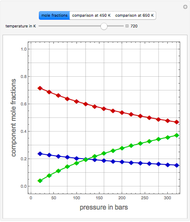
Consider the function family  , which depends on three parameters—
, which depends on three parameters— ,
,  , and
, and  —that you can vary. For the specified domain
—that you can vary. For the specified domain  ,
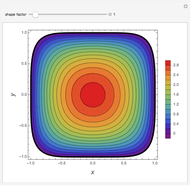
,  has many local minima, the locations of which depend on the parameters. Because
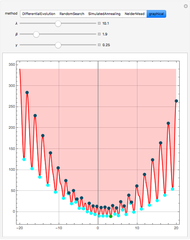
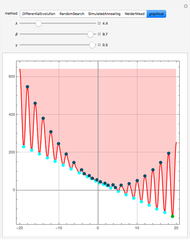
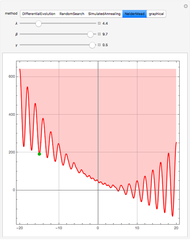
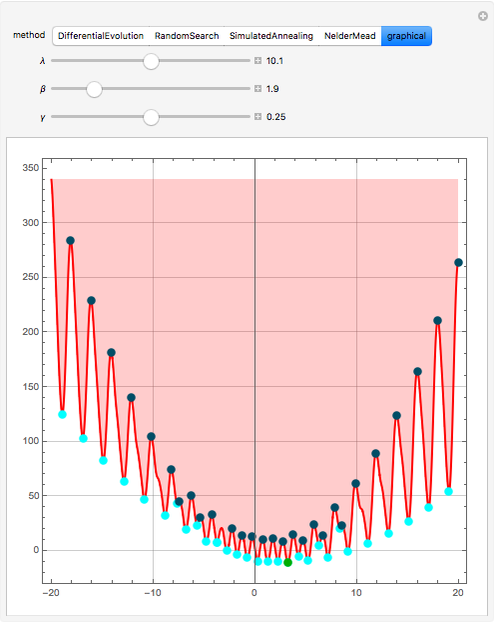
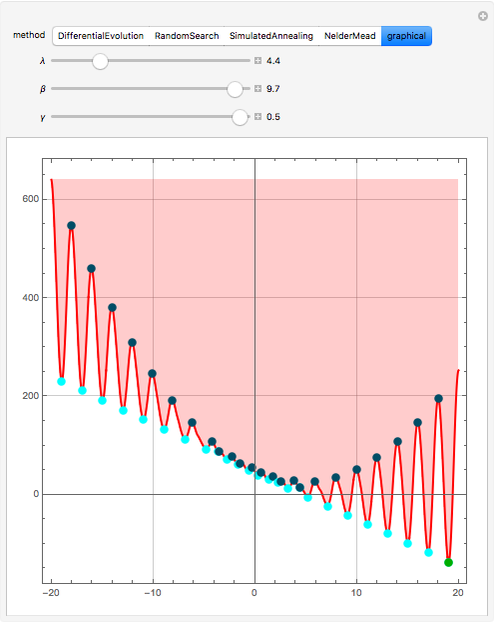
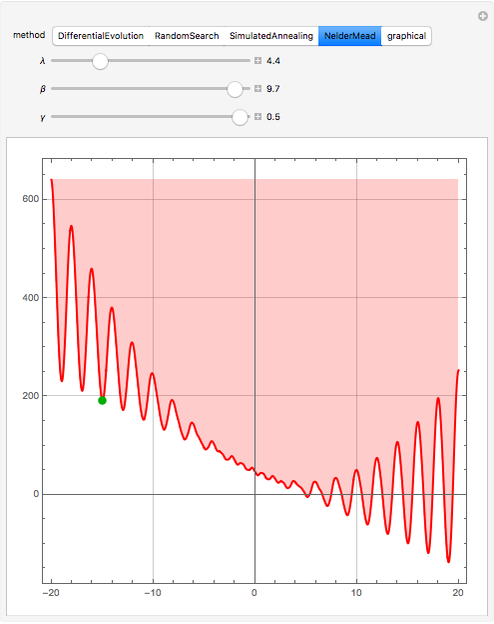
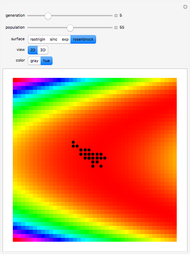
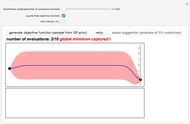
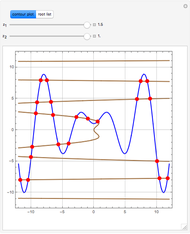
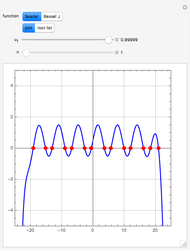
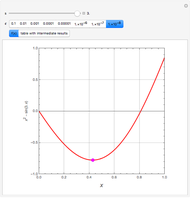
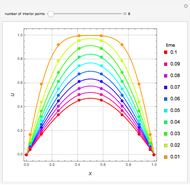
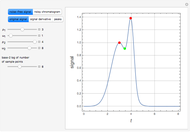
has many local minima, the locations of which depend on the parameters. Because  is nonlinear there is no guarantee that Mathematica's built-in function NMinimize will find a global minimum for a given set of its arguments. This Demonstration determines the position of all the local minima and maxima as well as the global minimum of
is nonlinear there is no guarantee that Mathematica's built-in function NMinimize will find a global minimum for a given set of its arguments. This Demonstration determines the position of all the local minima and maxima as well as the global minimum of  To do so, all roots of
To do so, all roots of  are computed using a graphical method developed by Wagon [1]. When
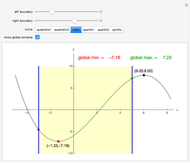
are computed using a graphical method developed by Wagon [1]. When  , local minima occur at
, local minima occur at  (cyan dots) and local maxima at
(cyan dots) and local maxima at  (the dark blue dots). The green dot indicates the position of the purported global minimum. Finally, the result obtained using the built-in Mathematica function NMinimize is given for comparison. You can select from four of the methods used by NMinimize to assess a method's capability to find the global minimum of
(the dark blue dots). The green dot indicates the position of the purported global minimum. Finally, the result obtained using the built-in Mathematica function NMinimize is given for comparison. You can select from four of the methods used by NMinimize to assess a method's capability to find the global minimum of  in the range
in the range  .
.
Contributed by: Housam Binous and Brian G. Higgins (March 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Reference
[1] S. Wagon, Mathematica in Action: Problem Solving through Visualization and Computation, 3rd ed., Berlin: Springer–Verlag, 2010.
Permanent Citation
"Global Minimum of a Non-Convex Function"
http://demonstrations.wolfram.com/GlobalMinimumOfANonConvexFunction/
Wolfram Demonstrations Project
Published: March 20 2012