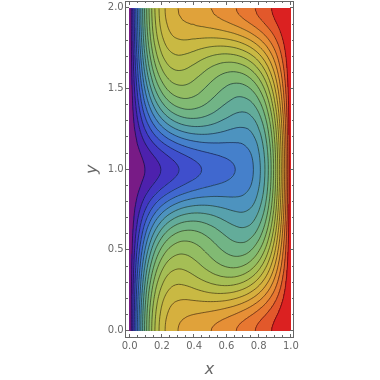
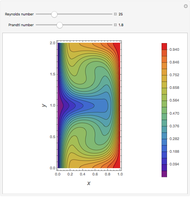
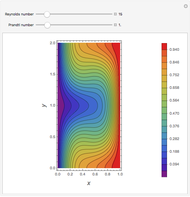
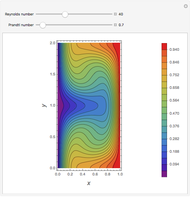
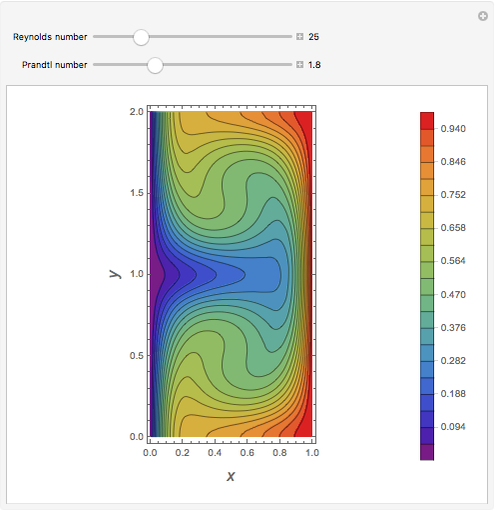
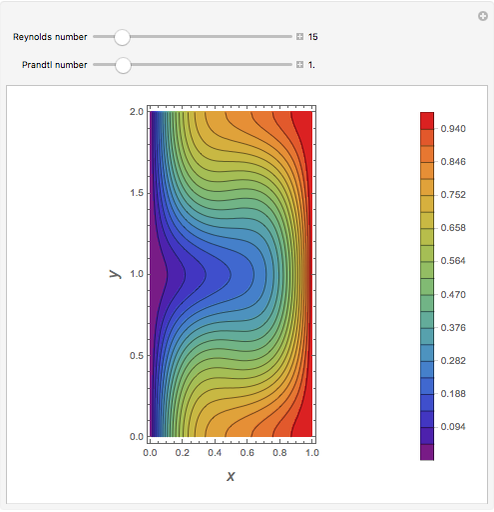
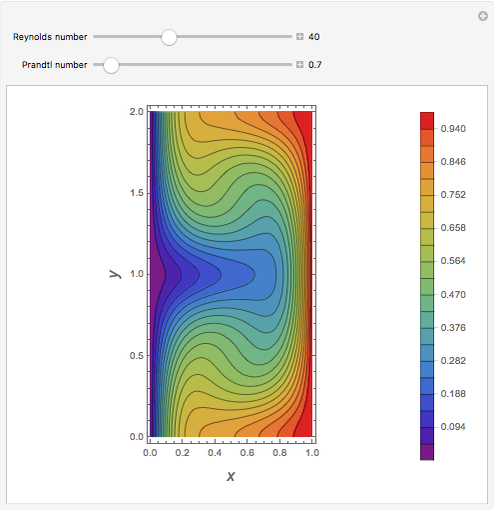
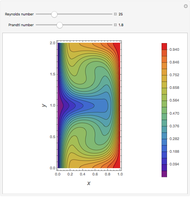
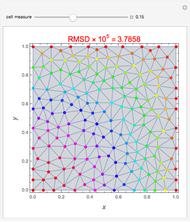
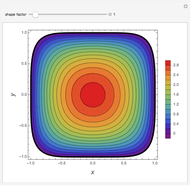
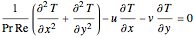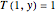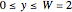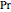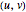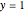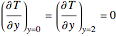Steady-State Two-Dimensional Convection-Diffusion Equation
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
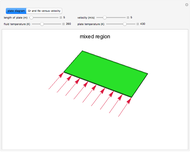
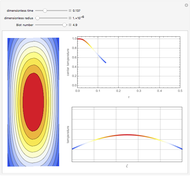
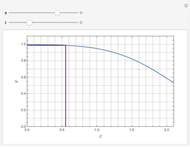
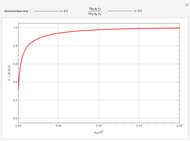
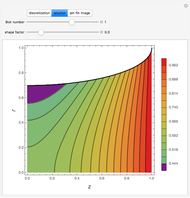
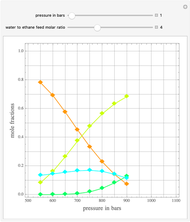
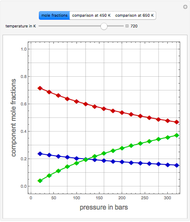
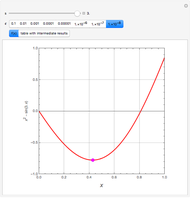
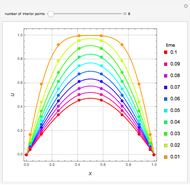
Consider the following two-dimensional convection-diffusion problem [1]:
[more]
Contributed by: Housam Binous, Ahmed Bellagi, and Brian G. Higgins (September 2015)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Reference
[1] S. Biringen and C.-Y. Chow, An Introduction to Computational Fluid Mechanics by Example, New York: Wiley, 2011.
Permanent Citation