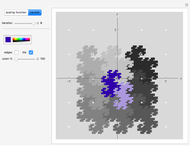
Ideal Nth-Band Discrete Filters

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
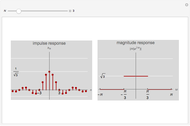
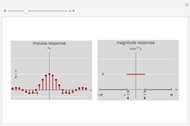
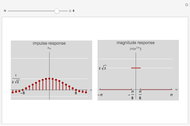
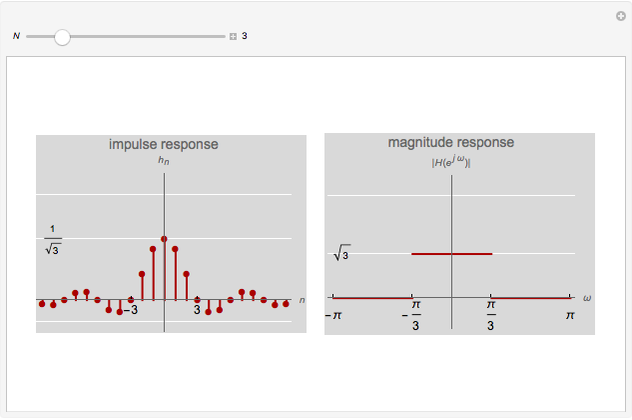
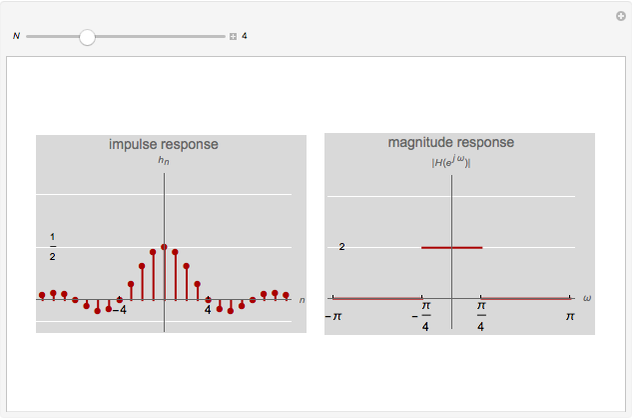
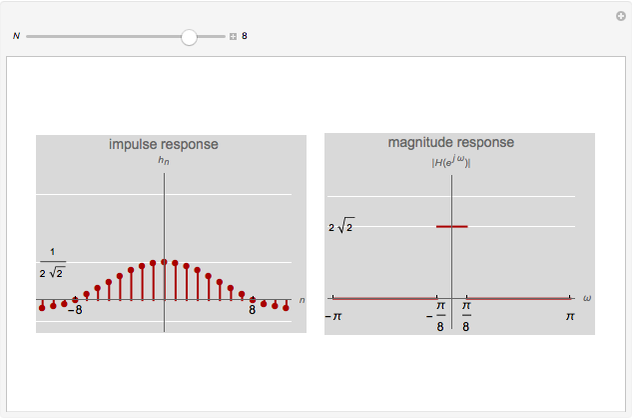
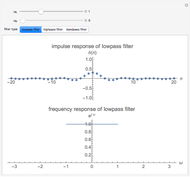
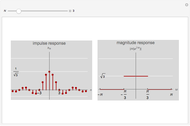
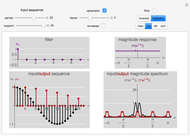
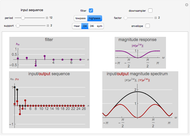
This Demonstration shows impulse and magnitude responses of ideal 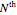 -band lowpass discrete filters for
-band lowpass discrete filters for 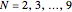 . While such filters are not realizable in practice, they serve as a desired template for passing certain frequencies while blocking others. The impulse response of an ideal lowpass filter is a sinc sequence (of unit norm in the figure), while its magnitude response is constant in the passband.
. While such filters are not realizable in practice, they serve as a desired template for passing certain frequencies while blocking others. The impulse response of an ideal lowpass filter is a sinc sequence (of unit norm in the figure), while its magnitude response is constant in the passband.
Contributed by: Jelena Kovacevic (May 2012)
Open content licensed under CC BY-NC-SA
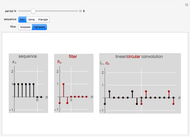
Snapshots
Details
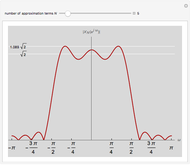
An ideal 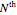 -band discrete filter is a non-realizable filter whose magnitude response takes a single nonzero value in its passband. For example, an ideal lowpass filter passes frequencies below some cut-off frequency
-band discrete filter is a non-realizable filter whose magnitude response takes a single nonzero value in its passband. For example, an ideal lowpass filter passes frequencies below some cut-off frequency 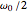 and blocks the others; its passband is thus the interval
and blocks the others; its passband is thus the interval 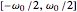 .
.
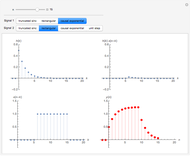
The impulse response of an ideal lowpass 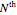 -band filter is a sinc sequence; its unit-norm version is
-band filter is a sinc sequence; its unit-norm version is
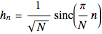 for
for 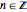 .
.
The impulse response at  is thus
is thus 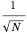 (see figure).
(see figure).
The magnitude response of a unit-norm 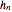 is (see figure)
is (see figure)
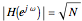 for
for 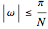 , and 0 otherwise.
, and 0 otherwise.
References
[1] M. Vetterli, J. Kovačević, and V. K. Goyal, Foundations of Signal Processing, Cambridge: Cambridge University Press, 2014. www.fourierandwavelets.org.
[2] Wikipedia. "Sinc Filter." (Nov 10, 2014) en.wikipedia.org/wiki/Sinc_filter.
Permanent Citation