Details

Recall that the derivative of a function is defined to be . For arbitrarily small values of , this says , or (A)

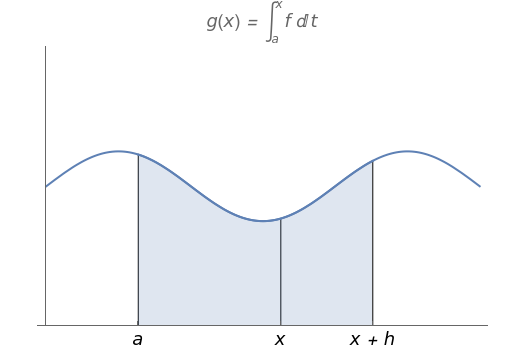
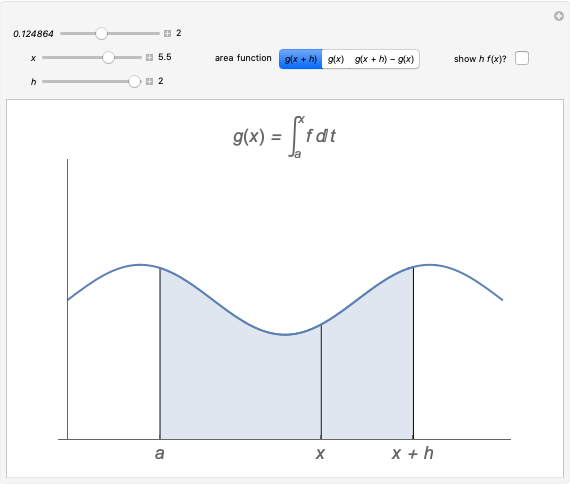
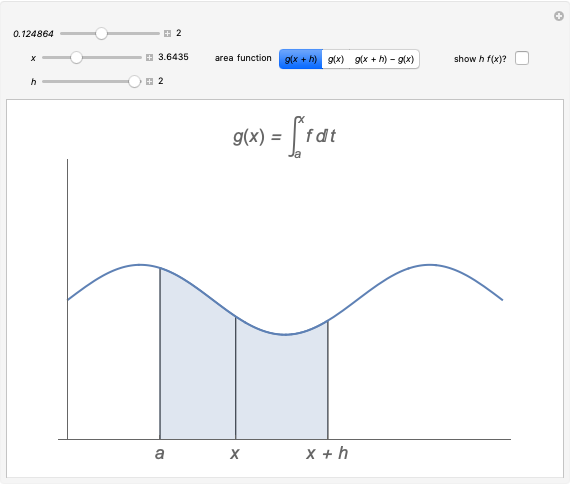
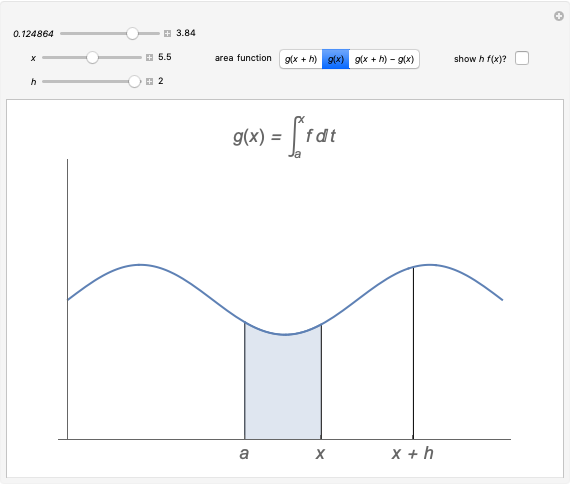
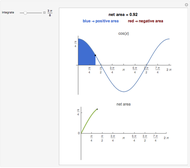
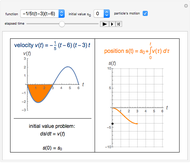
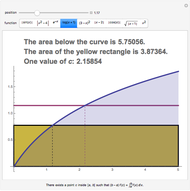
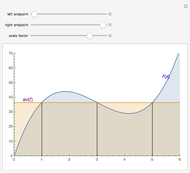
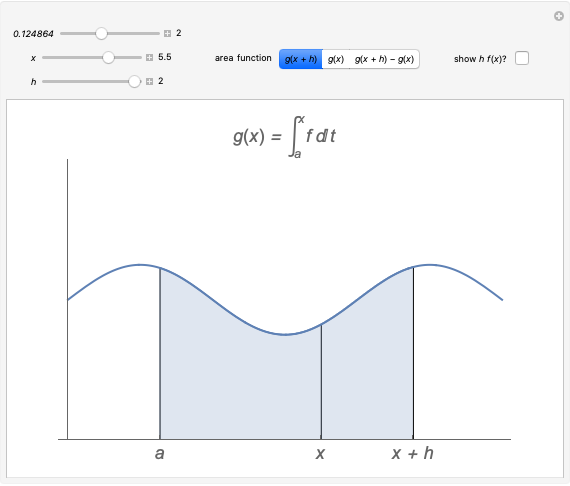
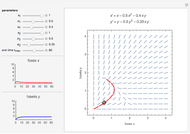
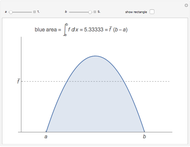
We use this idea to help develop the fundamental theorem of calculus. Define to be the area function, that is, . Then the Demonstration shows that and so by (A), (since the approximation is in fact an equality as we take the limit as ). Thus is the derivative of the area function, that is, as desired.