Laplace's Equation on a Circle

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
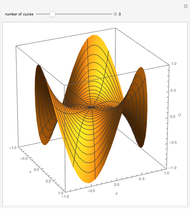
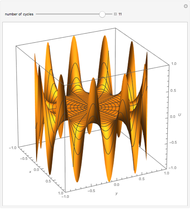
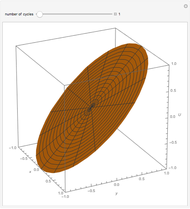
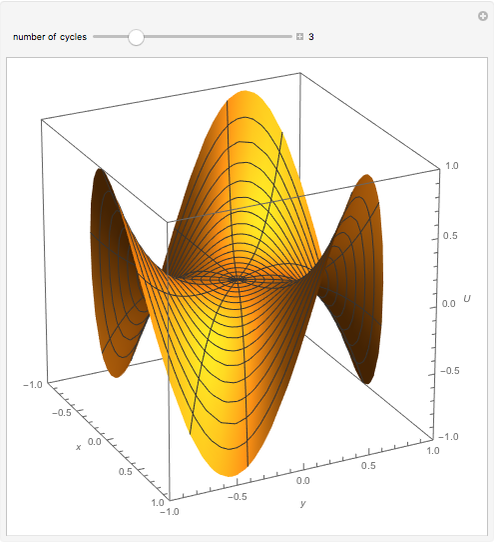
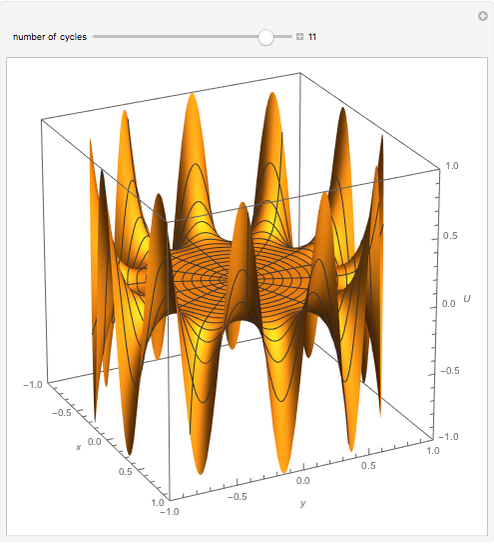
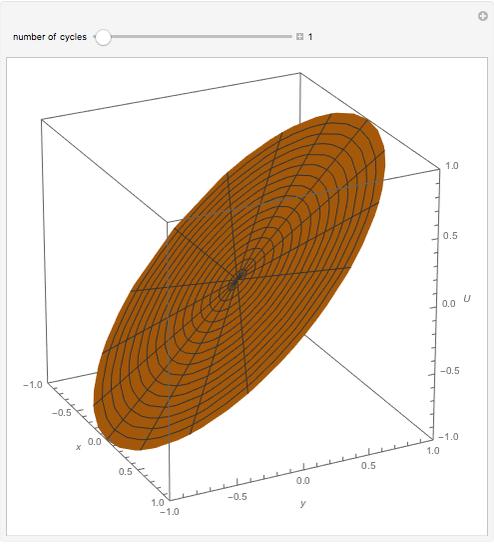
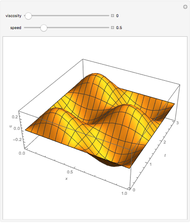
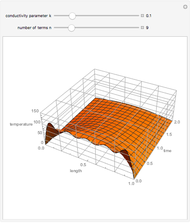
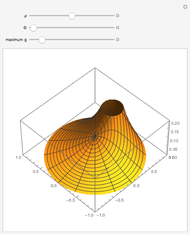
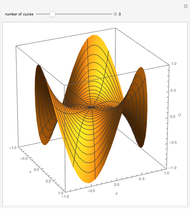
Given Dirichlet boundary conditions on the perimeter of a circle, Laplace's equation can be solved for the value of the surface in the interior of the circle. This Demonstration does so for a particularly simple boundary condition given by  cycles of a sine wave.
cycles of a sine wave.
Contributed by: David von Seggern (University of Nevada, Reno) (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Laplace's equation in two dimensions is given by
 .
.
Let a circular membrane  have a Dirichlet condition everywhere on the boundary, where the condition is
have a Dirichlet condition everywhere on the boundary, where the condition is  for
for  . The formal solution is
. The formal solution is

(P. Franklin, An Introduction to Fourier Methods and the Laplace Transformation, New York: Dover, 1958 p. 158). Solutions to Laplace's equation are called harmonic functions. One of the properties of harmonic functions is that they will not attain any local minima or maxima inside the boundary; thus the minima and maxima are on the boundaries, as defined by the Dirichlet conditions. Another property is that the solution at any point  has a value that is the average of the values over the area of a circle defined with
has a value that is the average of the values over the area of a circle defined with  at its center.
at its center.
Permanent Citation