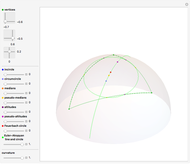
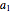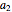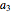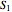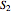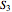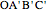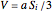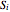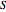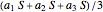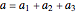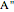Locus of Points at Constant Distance from the Edges of a Trihedron
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
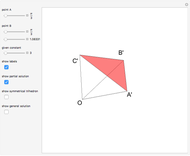
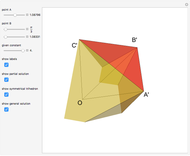
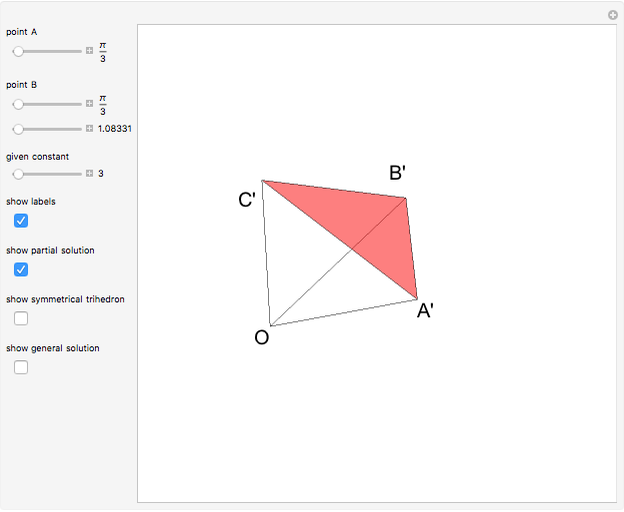
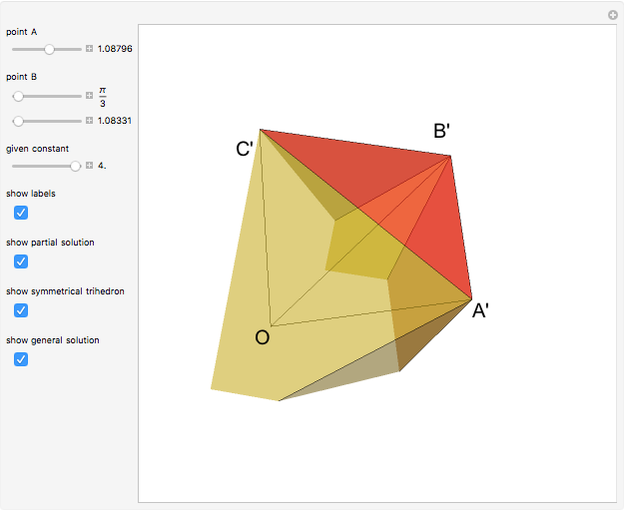
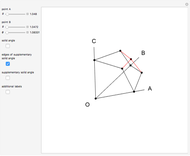
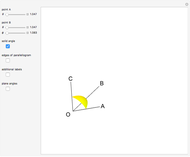
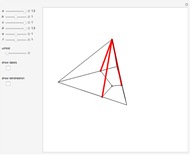
Choose a constant  and a trihedron with apex
and a trihedron with apex 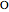 and edges
and edges 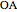 ,
, 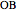 ,
, 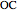 . How do you find the locus of points
. How do you find the locus of points 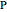 such that the sum of the distances of
such that the sum of the distances of 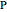 to the faces of the trihedron equals
to the faces of the trihedron equals  ?
?
Contributed by: Izidor Hafner (April 2017)
Open content licensed under CC BY-NC-SA
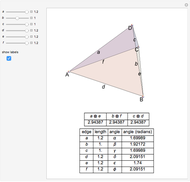
Snapshots
Details
This problem was posed in [1, p. 220]. For the original solution, see [1, p. 225].
Reference
[1] V. V. Prasolov and I. F. Sharygin, Problems in Stereometry (in Russian), Moscow: Nauka, 1989.
Permanent Citation