Magnetic Braking

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
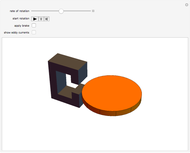
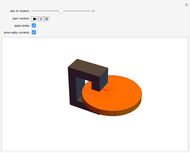
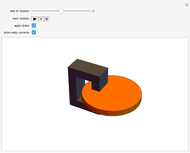
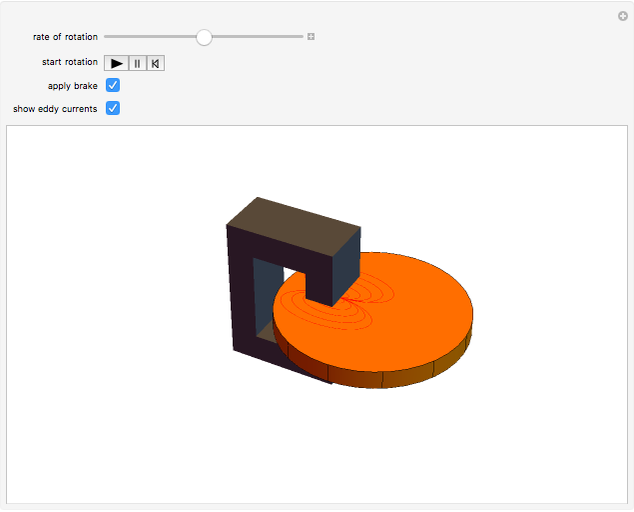
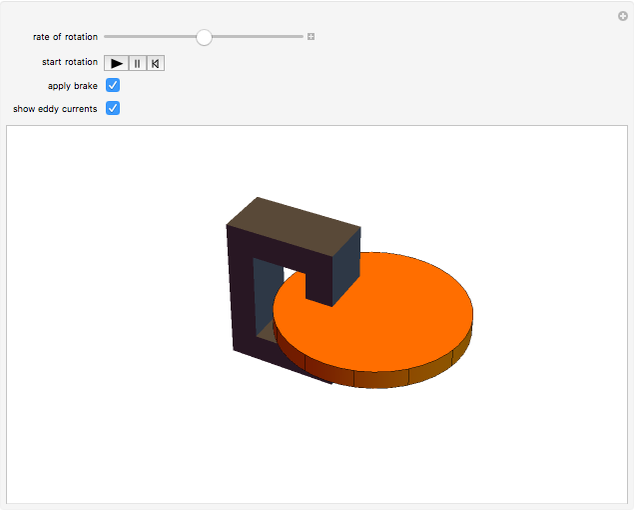
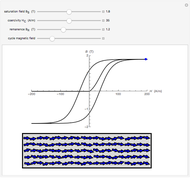
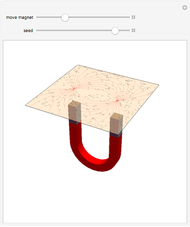
When an electrical conductor, such as copper or aluminum, moves through the field of a permanent magnet or an electromagnet, electromagnetic induction creates eddy currents, which dissipate some of the kinetic energy into Joule heat and results in slowing the motion of the conductor. This principle is utilized in the construction of magnetic brakes. This Demonstration shows magnetic braking applied to a rotating metallic disk. This might, for example, serve to control resistance to motion in exercise machines. Magnetic braking can also find applications in roller coasters and railroad trains, in which the metallic conductor has the shape of a linear rail. In contrast to conventional friction brakes, there is no direct contact between interacting surfaces, which makes magnetic braking more reliable and reduces wear and tear.
[more]
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Snapshot 1: metallic disk set into rotation
Snapshot 2: magnetic brake is applied; eddy currents are shown
Snapshot 3: disk brought to rest as eddy currents are damped out
Reference: H. D. Wiederick, N. Gauthier, D. A. Campbell, and R. Rochon, "Magnetic Braking: Simple Theory and Experiment," American Journal of Physics, 55(6), 1987 pp. 500–503.
Permanent Citation