Bell's Theorem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
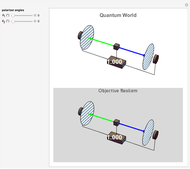
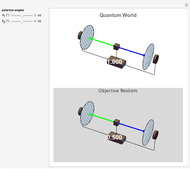
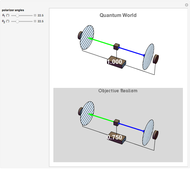
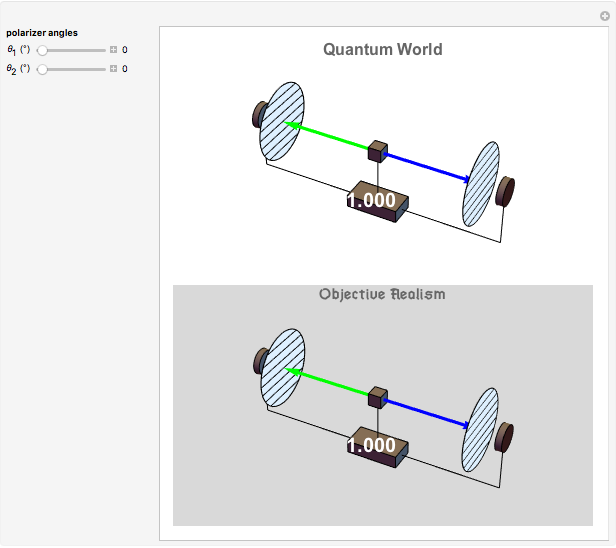
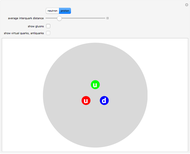
The Einstein-Podolsky-Rosen (EPR) Gedankenexperiment sought to demonstrate that quantum mechanics, although invariably predicting results in agreement with experiment, was an incomplete theory. In particular, the desirable philosophical feature of objective reality was lacking. According to the widely accepted Copenhagen interpretation, certain variables in a quantum system do not acquire definite values until they are measured. Thus they have no physical meaning in the absence of observation. Einstein disagreed vehemently. As he put it so succinctly, "Is the Moon still there when nobody looks?" Bohm reformulated the EPR experiment in terms of a pair of entangled spin- particles (also applicable to polarized photons) emitted from a compact source. It is a known fact that measurement of the polarization of one particle unambiguously determines the polarization of the other. From the viewpoint of objective realism, the states of both particles is assumed to exist independent of observation, perhaps as hidden variables not yet incorporated into the formalism of quantum mechanics.
particles (also applicable to polarized photons) emitted from a compact source. It is a known fact that measurement of the polarization of one particle unambiguously determines the polarization of the other. From the viewpoint of objective realism, the states of both particles is assumed to exist independent of observation, perhaps as hidden variables not yet incorporated into the formalism of quantum mechanics.
Contributed by: S. M. Blinder (May 2010)
Open content licensed under CC BY-NC-SA
Snapshots
Details
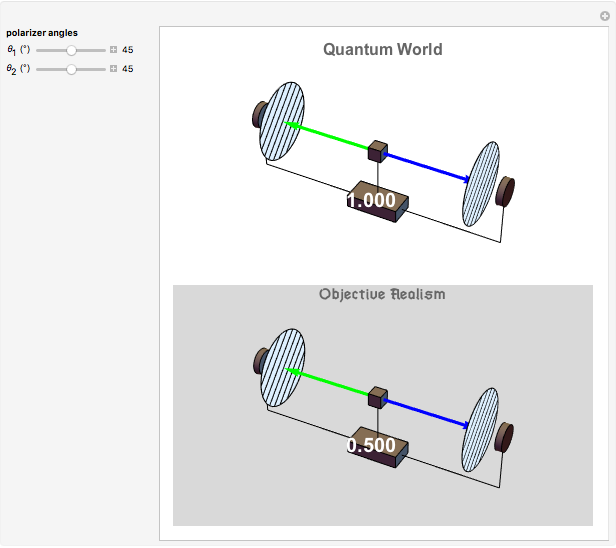
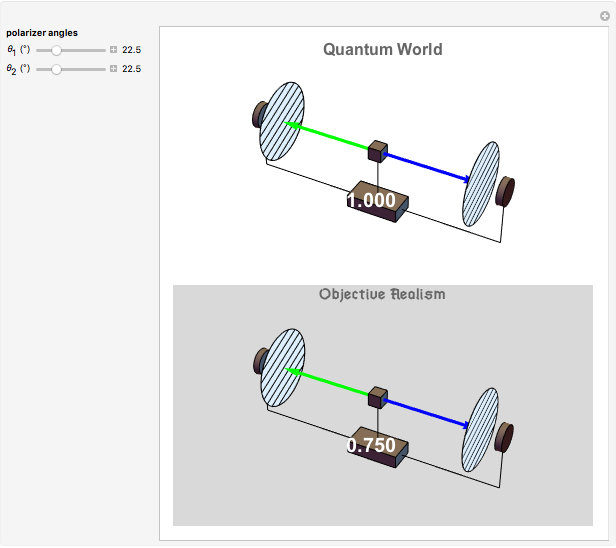
Correlated photons of wavelengths 551.3 nm (green) and 422.7 nm (blue) are produced by spontaneous emission from an excited state of atomic calcium produced by two-photon absorption.
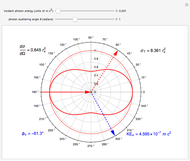
According to classical electromagnetic theory, linearly-polarized light will be transmitted by the fraction  through a polarizer oriented at the angle θ to the polarization plane. Objective reality assumes that both emitted photons carry this information. It can accordingly be surmised that the fraction
through a polarizer oriented at the angle θ to the polarization plane. Objective reality assumes that both emitted photons carry this information. It can accordingly be surmised that the fraction  will give a coincidence count. According to quantum mechanics, however, the coincidence fraction equals
will give a coincidence count. According to quantum mechanics, however, the coincidence fraction equals  -
- ). Since
). Since  , except when one or both of
, except when one or both of  and
and  equals 0 or
equals 0 or  , the predictions of QM and OR disagree.
, the predictions of QM and OR disagree.
References: J. S. Bell, "On the Einstein Podolsky Rosen paradox," Physics, 1(3) 1964 pp. 195-200. Reprinted in J. S. Bell, Speakable and Unspeakable in Quantum Mechanics, 2nd ed., Cambridge: Cambridge University Press, 2004; S. M. Blinder, Introduction to Quantum Mechanics, Amsterdam: Elsevier, 2004 pp. 272-277.
Permanent Citation