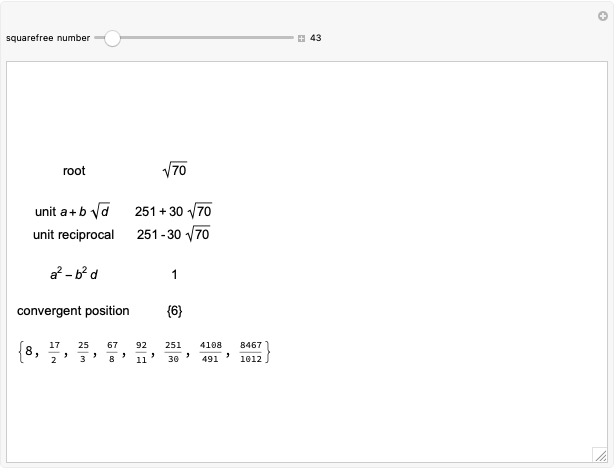
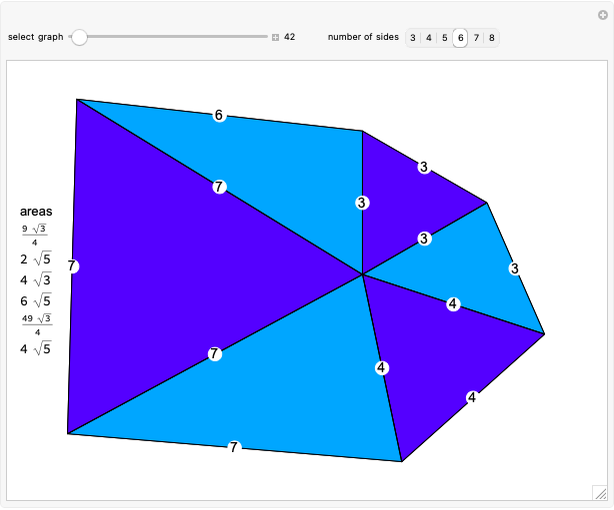
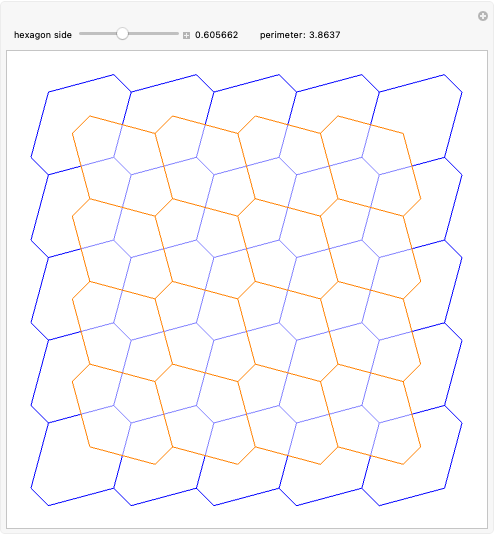
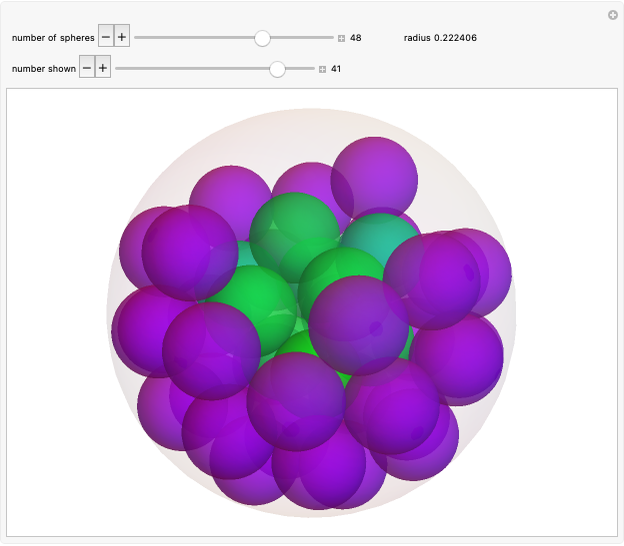
Melvyn Knight's Problem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
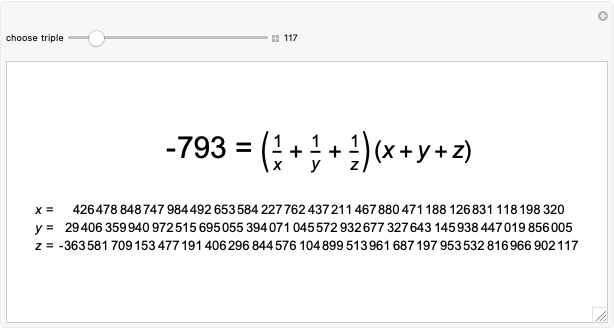
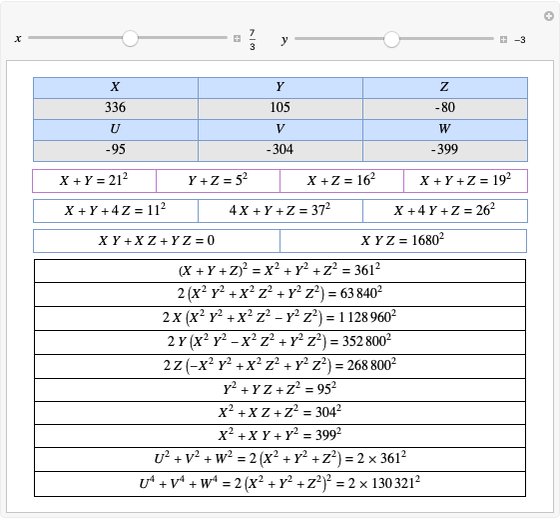
Melvyn Knight once asked [1] for which integers  is there an integer triple
is there an integer triple  so that
so that  .
.
Contributed by: Ed Pegg Jr (June 13)
Open content licensed under CC BY-NC-SA
Details
The original problem [1]

may be rewritten as
 ,
,
and further rewritten as
 .
.
Solving for  leads to a complicated expression equivalent to the elliptic curve:
leads to a complicated expression equivalent to the elliptic curve:
 .
.
A tabulation of the integers that can be represented is given in [2].
References
[1] A. Bremner, R. K. Guy and R. J. Nowakowski, "Which Integers Are Representable as the Product of the Sum of Three Integers with the Sum of Their Reciprocals?," Mathematics of Computation, 61(203), 1993 pp. 117–130. www.ams.org/journals/mcom/1993-61-203/S0025-5718-1993-1189516-5/S0025-5718-1993-1189516-5.pdf.
[2] H. Pfoertner. "Integers n representable as the product of the sum of three nonzero integers with the sum of their reciprocals." The On-Line Encyclopedia of Integer Sequences. (Jan 24, 2023) oeis.org/A085514.
Snapshots
Permanent Citation