Multiplication Tables for the Group of Integers Modulo n

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
Given a positive integer  , the set
, the set  of positive integers coprime to
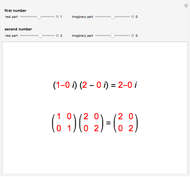
of positive integers coprime to  satisfies the axioms for an Abelian group under the operation of multiplication modulo
satisfies the axioms for an Abelian group under the operation of multiplication modulo  . For instance,
. For instance,  and
and  because
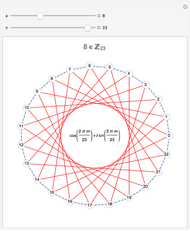
because  . This Demonstration shows the array plot of the multiplication table modulo
. This Demonstration shows the array plot of the multiplication table modulo  corresponding to
corresponding to  .
.
Contributed by: Jaime Rangel-Mondragon (August 2012)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The order of  is given by Euler's totient function
is given by Euler's totient function  , implemented in Mathematica as EulerPhi[n], which for
, implemented in Mathematica as EulerPhi[n], which for  has values
has values  .
.  is cyclic only if
is cyclic only if  is
is  , or
, or  , where
, where  is an odd prime and
is an odd prime and  . The first few values for which
. The first few values for which  is not cyclic are
is not cyclic are  . Any generator in the cyclic case is called a primitive root modulo
. Any generator in the cyclic case is called a primitive root modulo 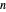 .
.
Reference
[1] Wikipedia. "Multiplicative Group of Integers Modulo n." (Jul 31, 2012) en.wikipedia.org/wiki/Multiplicative_group_of _integers _modulo _n.
Permanent Citation