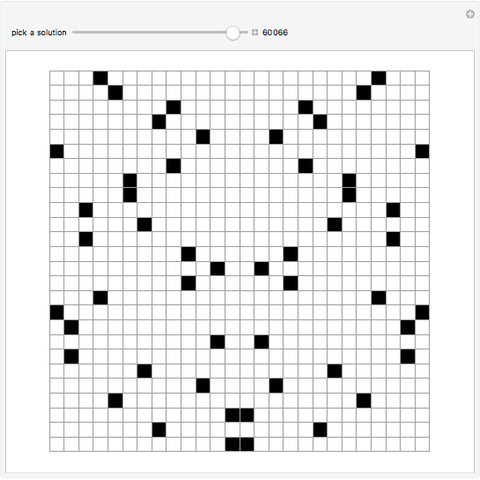
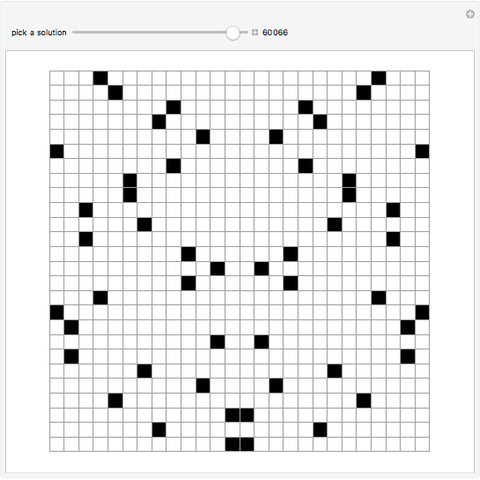
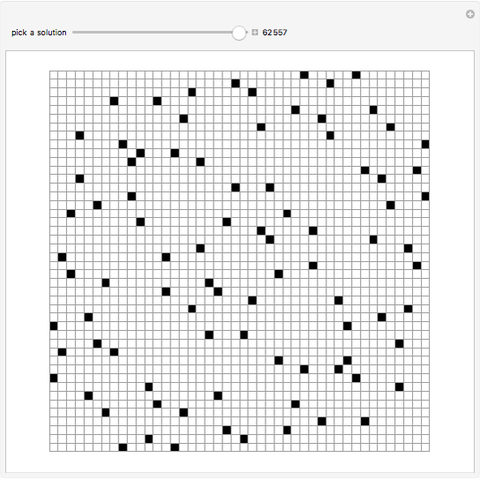
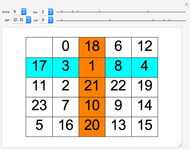
In 1917, Henry Dudeney asked for the maximum number of points selectable from an × grid so that no three points are collinear. Obviously, no more than two points can be selected from any row or column, so the maximal amount of points is 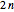. Calling a solution an × grid with a maximal selection of 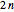points with no three points collinear, a 52×52 grid with 104 selected points is the largest known solution. This Demonstration gives all solutions up to order 18×18, and some of the known solutions for larger-sized grids.