Orthonormal Polynomials under Different Inner Product Measures

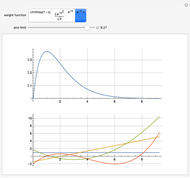
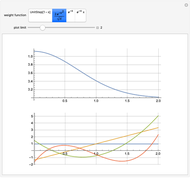
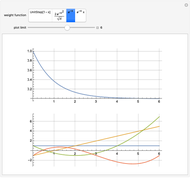
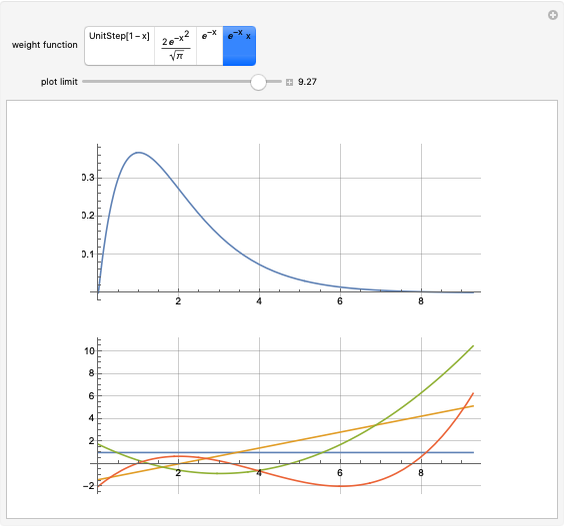
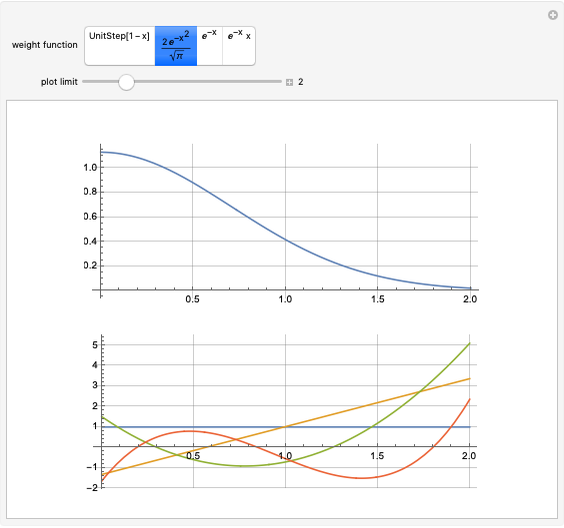
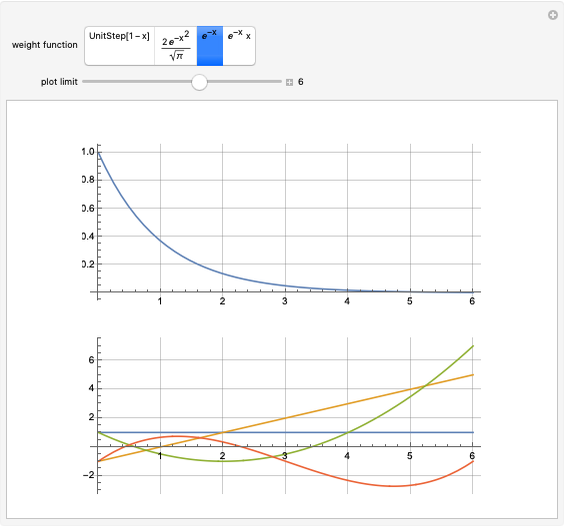
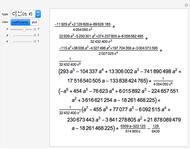
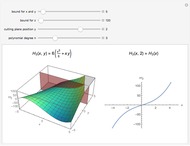
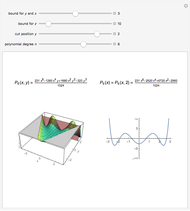
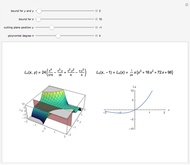
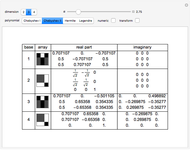
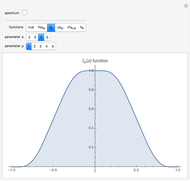
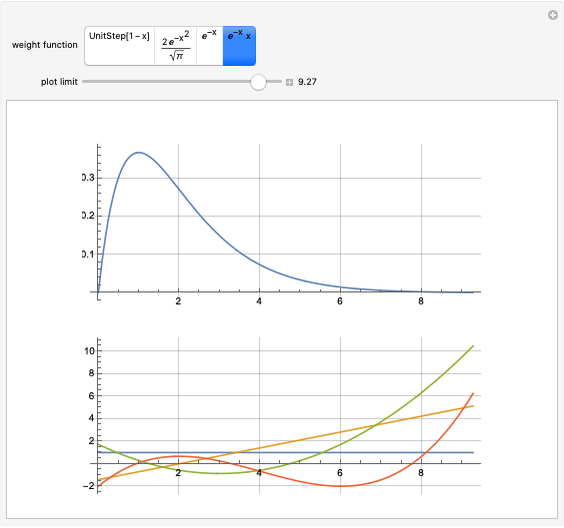
This Demonstration shows how the orthonormal polynomials of a space where the inner product is a weighted integral vary with the weight function , . Here, the monomial basis is orthonormalized by the Gram–Schmidt process with respect to the inner product 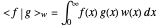and the weight functions are probability measures (so that ) corresponding to the Legendre, Hermite, Laguerre and generalized Laguerre polynomials.