The Geometry of Hermite Polynomials

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
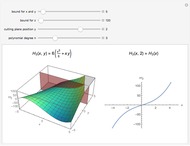
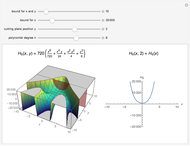
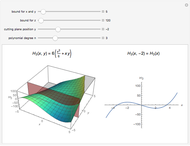
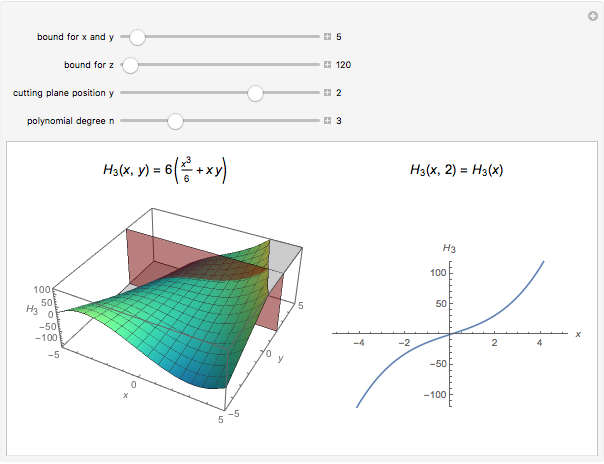
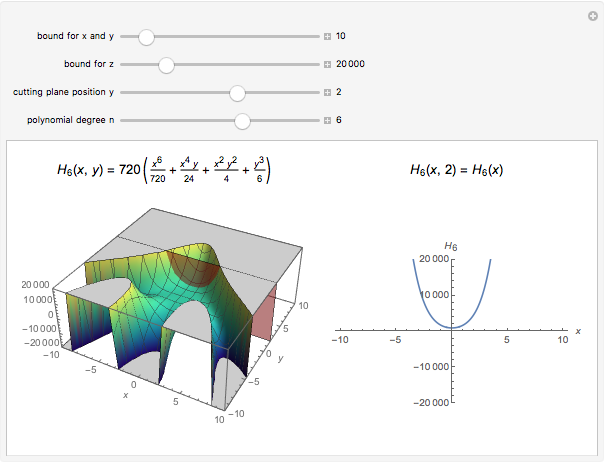
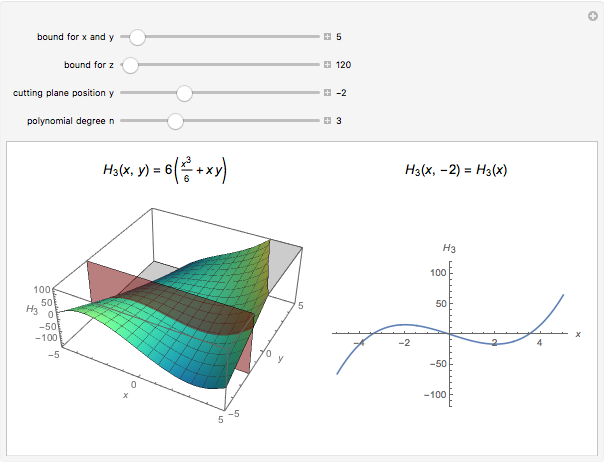
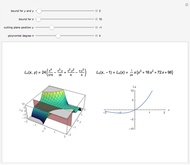
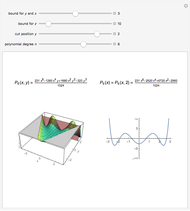
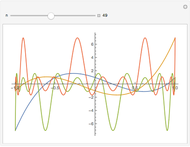
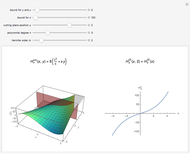
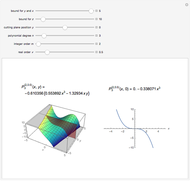
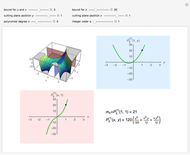
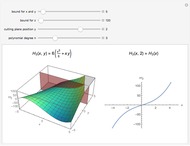
On the left is a three-dimensional plot of a Hermite polynomial in two variables  and
and  , and on the right is a 2D plot of the surface cut by a plane perpendicular to the
, and on the right is a 2D plot of the surface cut by a plane perpendicular to the  axis.
axis.
Contributed by: Marcello Artioli and Giuseppe Dattoli (March 2015)
Open content licensed under CC BY-NC-SA
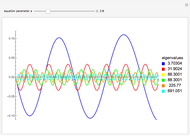
Snapshots
Details
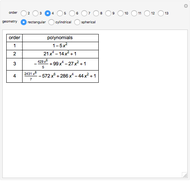
The two-variable Hermite polynomial

has been shown to be the solution of the heat equation

with boundary condition
 .
.
The solution written in an operational form reads
 ,
,
which can be exploited to infer a kind of geometrical understanding of the Hermite polynomials in 3D.
The geometrical content of this operational identity is shown in  -
- -
- space. The exponential operator transforms an ordinary monomial into a special polynomial of the Hermite type. The monomial-polynomial evolution is shown by moving the cutting plane orthogonal to the
space. The exponential operator transforms an ordinary monomial into a special polynomial of the Hermite type. The monomial-polynomial evolution is shown by moving the cutting plane orthogonal to the  axis. For a specific value of the polynomial degree
axis. For a specific value of the polynomial degree  , the polynomials lie on the cutting plane, as shown in the snapshots. It is worth stressing that only for negative values of
, the polynomials lie on the cutting plane, as shown in the snapshots. It is worth stressing that only for negative values of  do the polynomials exhibit zeros (snapshots 3 and 4), in accordance with the fact that in this region they realize an orthogonal set.
do the polynomials exhibit zeros (snapshots 3 and 4), in accordance with the fact that in this region they realize an orthogonal set.
References
[1] P. Appell and Kampé de Fériét, Fonctions hypergéométriques et hypersphériques polynômes d'Hermite, Paris: Gautier-Villars, 1926.
[2] G. Dattoli, "Generalized Polynomials, Operational Identities and Their Applications," Journal of Computational and Applied Mathematics, 118(1–2), 2000 pp. 19–28. doi:10.1016/S0377-0427(00)00283-1.
Permanent Citation