Wolfram Demonstrations Project

13,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Pendulum Towing a Rolling Disk

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

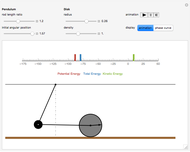
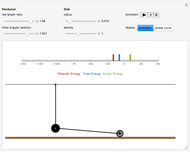
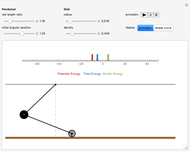
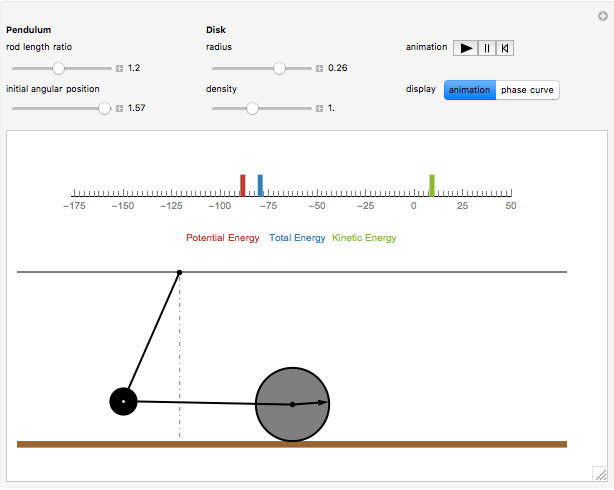
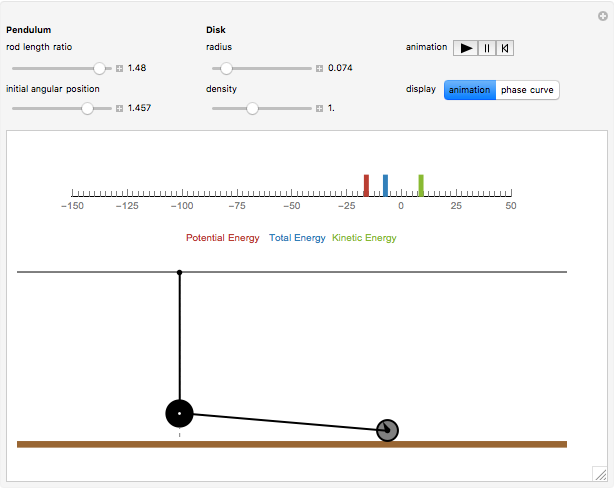
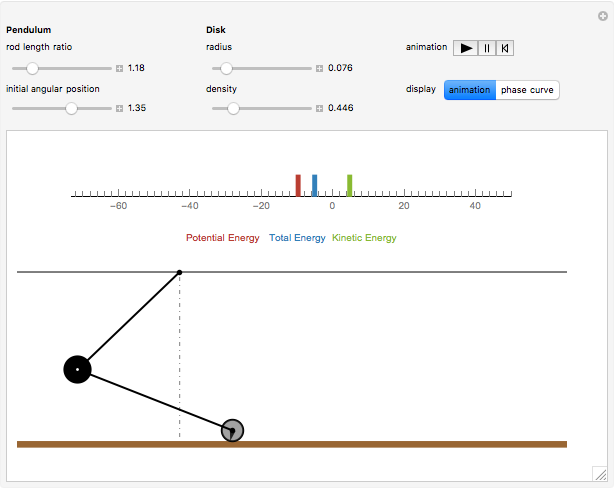
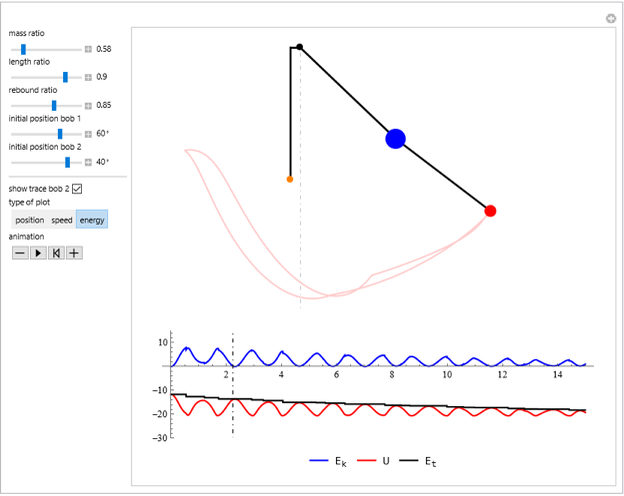
This Demonstration simulates the motion of a double pendulum with a rolling disk attached to the end of its lower rod. The disk is free to roll without friction over a horizontal plane.

[more]

Lagrangian mechanics are used to determine the equations of motion. You can change the geometrical parameters using the sliders.

A phase curve gives an overview of the state of the system. A horizontal energy gauge shows the total energy of the system, which remains constant while the potential and kinetic energies change in opposite directions.

[less]

Contributed by: Erik Mahieu (October 2014)
Open content licensed under CC BY-NC-SA

Snapshots

Details

The pendulum's upper and lower rods have lengths and and angular positions and . The upper bob has mass . The disk has angular position , mass , and radius .

This system has only one degree of freedom: . The other variables are fixed by the algebraic constraints:

,

.

The potential energy of the disk and pendulum system is .

The kinetic energy is .

The Lagrangian of this system is . Substituting this in the Euler–Lagrange equations for and gives

,

,

which results in the equation of motion:

,

where and .

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send