Disk Rolling inside a Rotating Ring

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
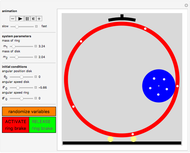
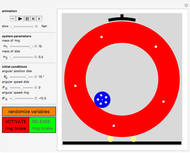
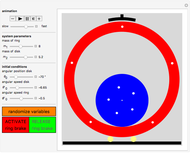
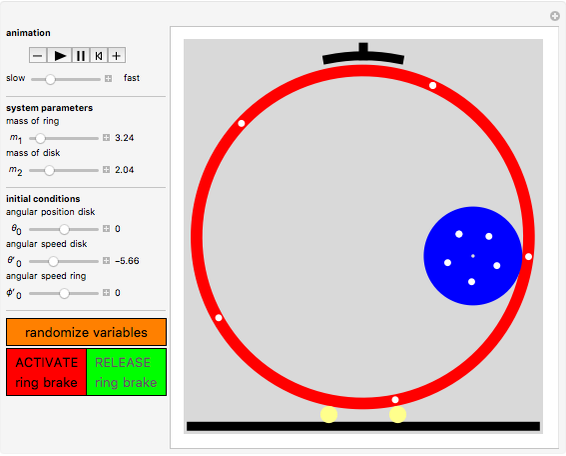
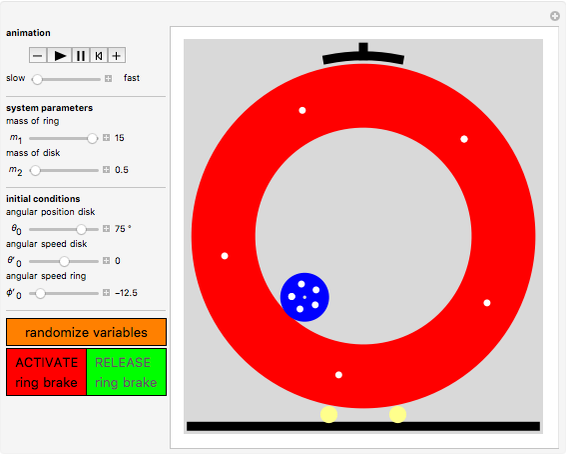
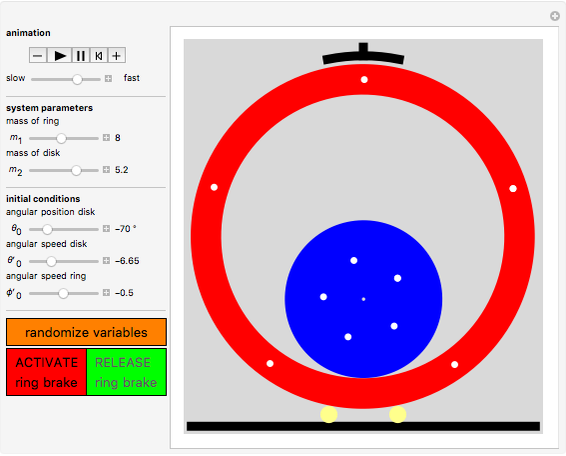
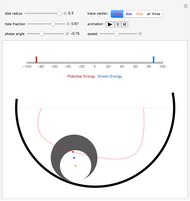
This Demonstration shows a disk rolling, without slipping, inside a rotating ring.
[more]
Contributed by: Erik Mahieu (September 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
A steel ring of mass 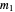 is supported at the bottom by two frictionless Teflon rollers. This ring can be rotated with an initial angular speed
is supported at the bottom by two frictionless Teflon rollers. This ring can be rotated with an initial angular speed 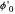 . Inside the ring, a steel disk of mass
. Inside the ring, a steel disk of mass 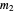 is released from angular position
is released from angular position 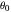 with an angular speed
with an angular speed 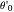 . The friction between the ring and disk is sufficient to avoid sliding.
. The friction between the ring and disk is sufficient to avoid sliding.
Lagrangian mechanics are used to extract the equations of motion for this system with two degrees of freedom:
1.  or the angle between the vertical and the line connecting the center of the disk with the center of the ring;
or the angle between the vertical and the line connecting the center of the disk with the center of the ring;
2.  or the angle of rotation of the ring around its center.
or the angle of rotation of the ring around its center.
Since no slipping is allowed between the disk and the ring, 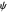 or the angle of rotation of the disk around its center can be calculated from
or the angle of rotation of the disk around its center can be calculated from  and
and  and the radii of the disk and ring:
and the radii of the disk and ring: 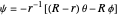 , where
, where 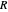 is the inner radius of the ring and
is the inner radius of the ring and  is the radius of the disk.
is the radius of the disk.
The Lagrangian of the system is the difference between the kinetic and potential energies:
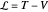 ,
,
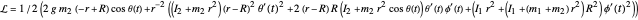
where 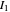 and
and 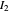 are the moments of inertia of the ring and disk.
are the moments of inertia of the ring and disk.
The equations of motion can be derived from the Euler–Lagrange equations:
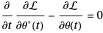 ,
,
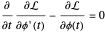 ,
,
resulting in:
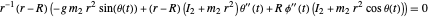 ,
,
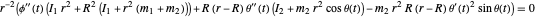 .
.
In order to better verify the rotations of ring and disk, you may have to slow down the animation to eliminate stroboscopic effects. Run the bookmark "sliding check" at different speeds to convince yourself.
See also a similar Demonstration by Christoph Meyer: "Solid Cylinder Rolling in a Turnable Hollow Cylinder".
Permanent Citation