Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Riemann Hypothesis

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

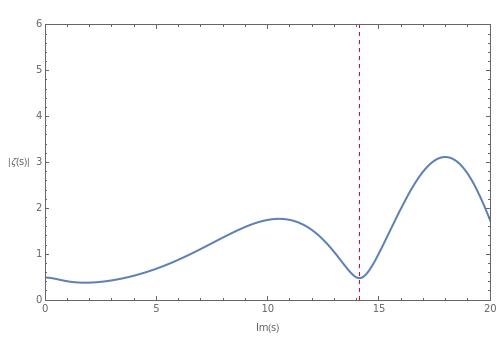
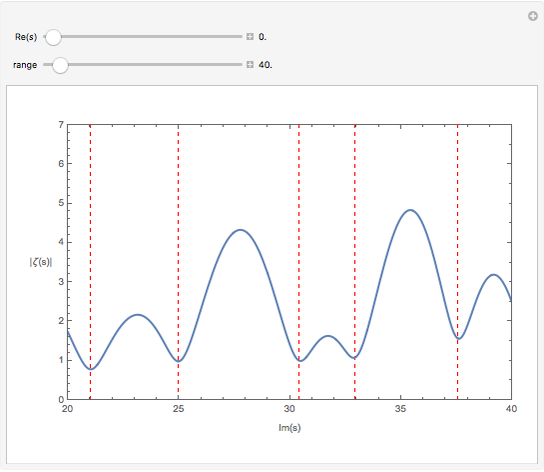
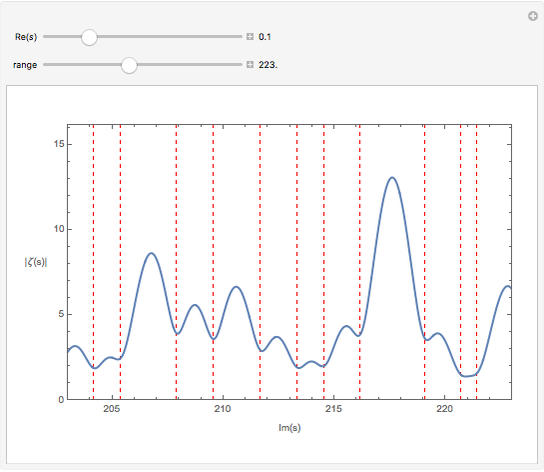
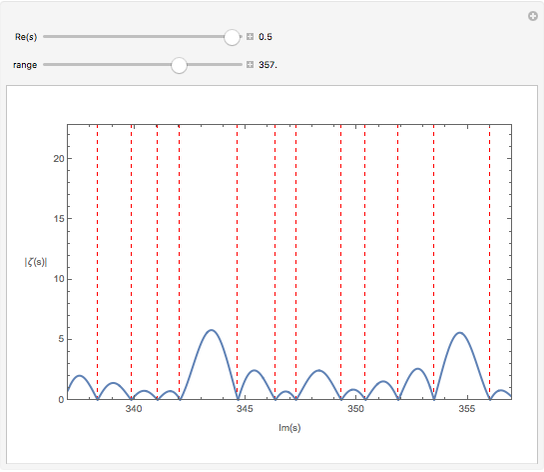
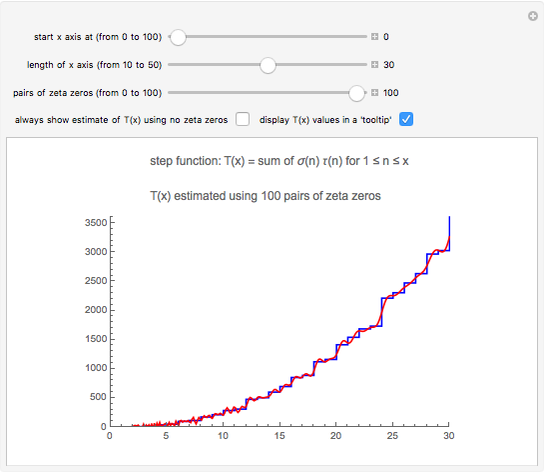
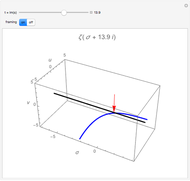
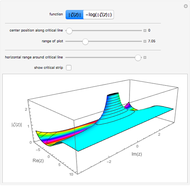
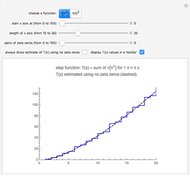
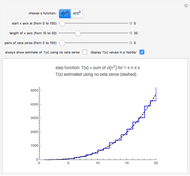
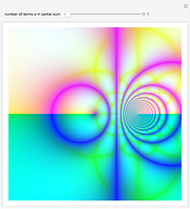
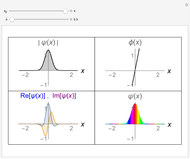
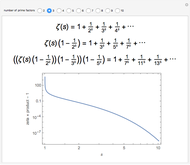
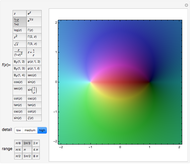
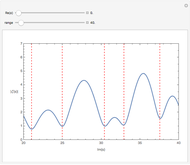
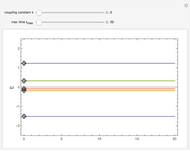
The Riemann hypothesis is one of today's most important problems in mathematics. The hypothesis states that all of the nontrivial zeros of the Riemann zeta function are located on the critical line . A $1,000,000 prize has been offered by the Clay Mathematics Institute for the first correct proof of the hypothesis.

[more]

The hypothesis was first formulated by Riemann in 1859 and has remained unsolved since then. It is known that the nontrivial zeros are located in the crtical strip , moreover if we define , then , which shows that the zeros must be symmetric with respect to the critical line.

This Demonstration plots the absolute value of the zeta function with respect to the imaginary part of its argument. You can change the range of the plot and the real part of . The dashed red lines show the position of the imaginary part of the zeros.

[less]

Contributed by: Baris Altunkaynak (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send