The Integraph: An Integrating Mechanism

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
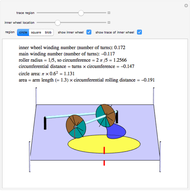
Before the Differential Analyzer and long before Mathematica or Wolfram|Alpha, mechanical instruments were used to perform computations. The "Integraph" [1] in this Demonstration plots the integral curve  starting from a given curve
starting from a given curve  .
.
Contributed by: Erik Mahieu (December 2015)
Open content licensed under CC BY-NC-SA
Snapshots
Details
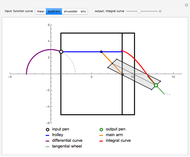
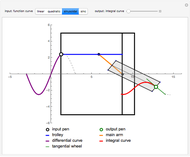
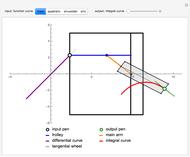
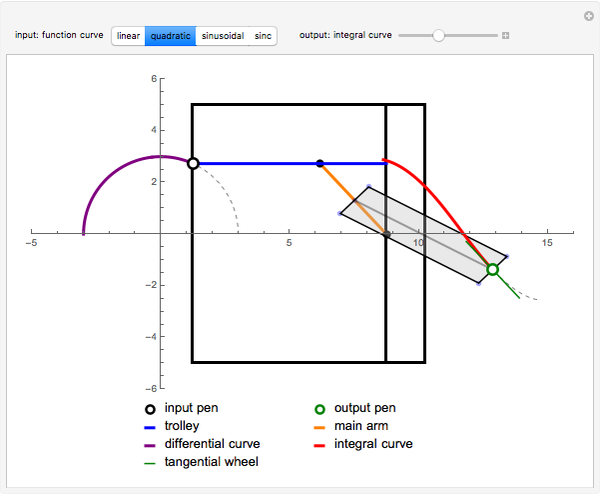
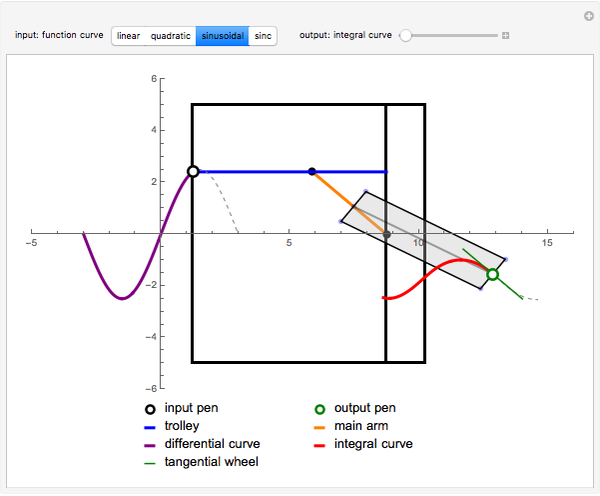
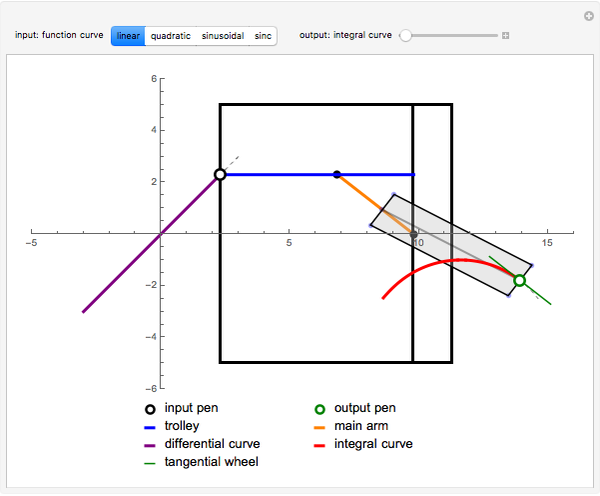
The integral curve is drawn by a pen that moves in a (tangential) direction proportional to the local value of the given curve. This is achieved by the following mechanism.
The integraph consists of a rectangular carriage that moves left to right on rollers along the  axis.
axis.
Drag the input pen along the given curve, following a given path  . The input pen moves a trolley up and down the carriage and rotates the main arm around a fixed point on the carriage. The direction
. The input pen moves a trolley up and down the carriage and rotates the main arm around a fixed point on the carriage. The direction  of the main arm is a function of the input
of the main arm is a function of the input  .
.
 with
with  being the length of the main arm.
being the length of the main arm.
The output pen is guided parallel to the main arm by means of a tangential wheel connected to the main arm by a parallelogram linkage. This way the tangent  of the output curve
of the output curve  will be proportional to the input
will be proportional to the input  . This is expressed by the differential equation
. This is expressed by the differential equation
 ,
,
with solution  .
The parallelogram keeps the wheel parallel to the main arm, independent of the vertical position of the output pen.
.
The parallelogram keeps the wheel parallel to the main arm, independent of the vertical position of the output pen.
References
[1] Wikipedia. "Integraph." (Nov 20, 2015) en.wikipedia.org/wiki/Integraph.
[2] Wikipedia. "Bruno Abakanowicz." (Nov 20, 2015) en.wikipedia.org/wiki/Bruno_Abakanowicz.
[3] G. Coradi, "Intégraphes Nos. 40 et 41," Original-Katalog, No. 37, p. 12. www.g.coradi.com.
[4] P. Garcia. "Integraphs," DrawingMachines.org. (Dec 4, 2015) drawingmachines.org/category.php?id=36.
Permanent Citation