Associated Surface of a Minimal Möbius Strip

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
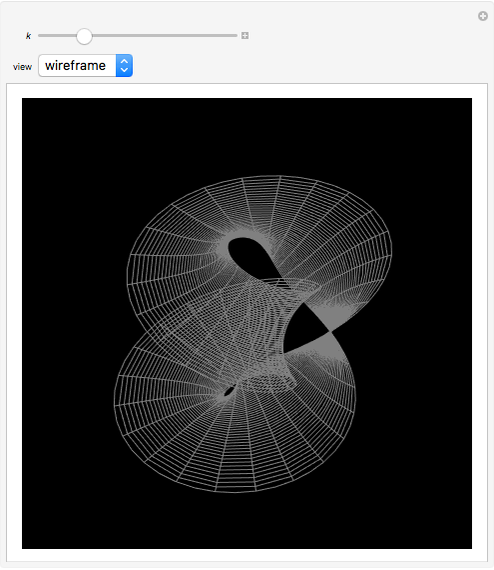
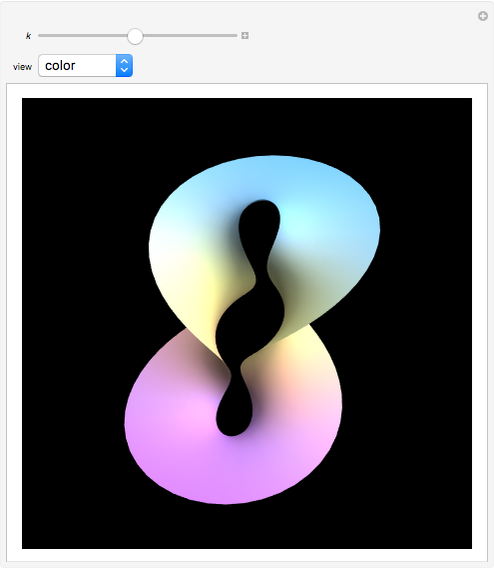
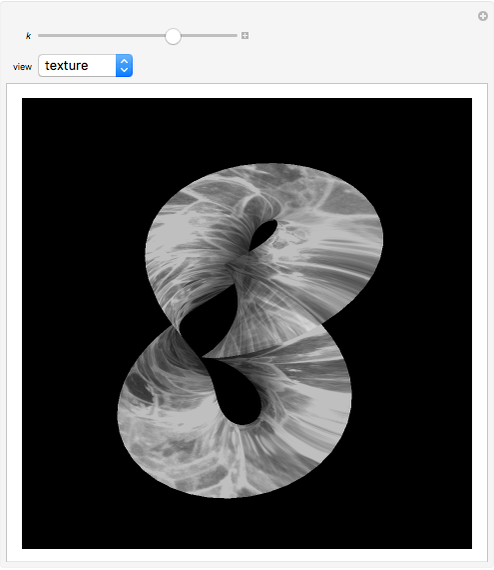
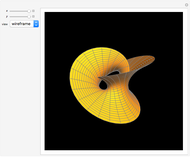
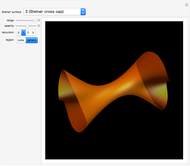
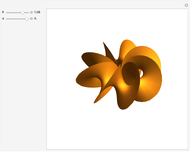
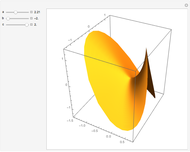
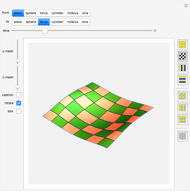
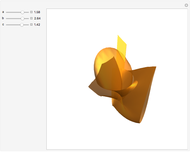
This Demonstration shows a minimal version of the celebrated Möbius strip (a nonorientable surface or one-sided surface). The surface is obtained by computing a Björling curve [1], extrapolating a surface when a determined curve (in this case the circle) is known, along with a given unit normal. The associated surface shown here can be generated by means of a complex conformal transformation, simply by multiplying the minimal curve by the factor 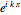 [2].
[2].
Contributed by: Enrique Zeleny (June 2014)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Starting with a circle 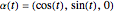 , we construct a winding normal that is
, we construct a winding normal that is  . Using the Björling formula with these curves, we obtain the minimal Möbius strip
. Using the Björling formula with these curves, we obtain the minimal Möbius strip
 .
.
References
[1] P. Mira, "Complete Minimal Möebius Strips in  and the Björling Problem," Journal of Geometry and Physics, 56(9), 2006 pp. 1506–1515. filemon.upct.es/~pmira/pdf/MobiusCTF.pdf.
and the Björling Problem," Journal of Geometry and Physics, 56(9), 2006 pp. 1506–1515. filemon.upct.es/~pmira/pdf/MobiusCTF.pdf.
[2] H. Gollek. "Natural Equations and Deformations of Minimal Curves." (Jun 20, 2014) www-irm.mathematik.hu-berlin.de/~gollek/MinSurfs/min.ps.
Permanent Citation