Bernoulli's Theorem

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
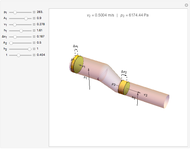
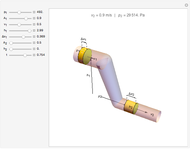
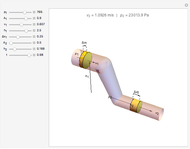
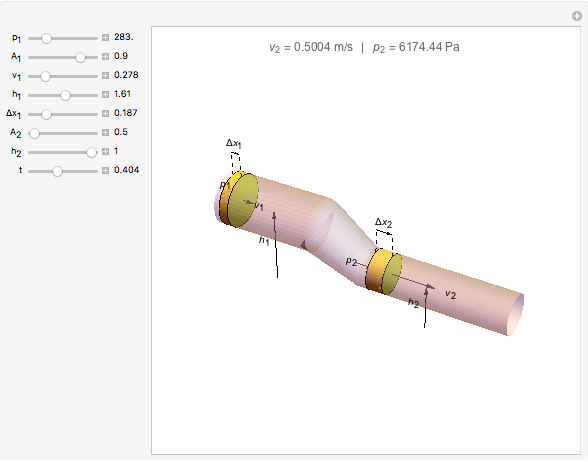
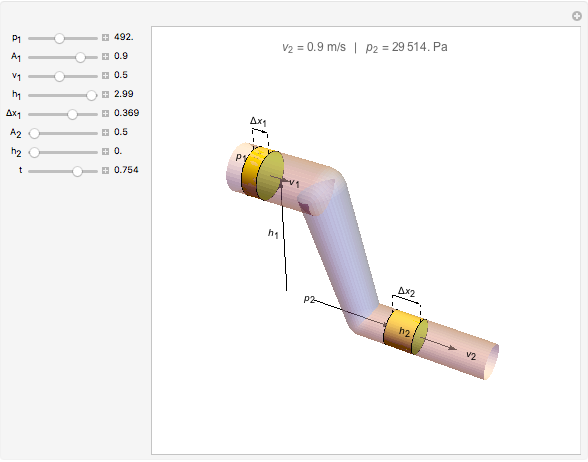
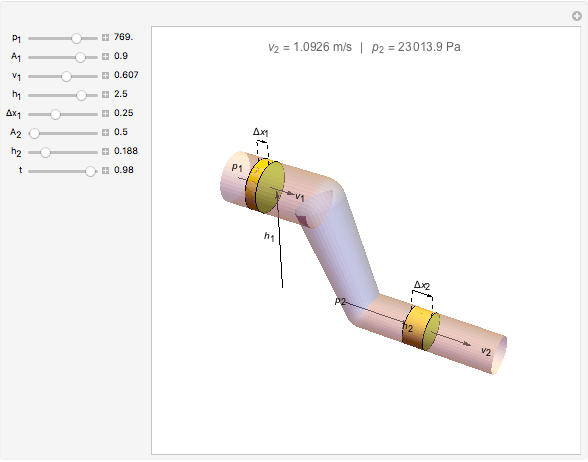
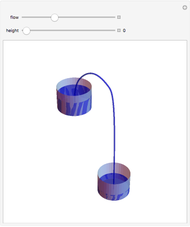
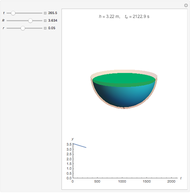
Bernoulli's theorem is implied by the conservation of mass and energy in fluid flow. Consider a nonviscous, incompressible fluid flowing through a pipe with cross-sectional area  and pressure
and pressure  , such that an element
, such that an element  is moved a distance
is moved a distance  . The theorem states that the sum of the pressure, the potential, and kinetic energy per unit volume is equal to a fixed constant at any point of a fluid.
. The theorem states that the sum of the pressure, the potential, and kinetic energy per unit volume is equal to a fixed constant at any point of a fluid.
Contributed by: Enrique Zeleny (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
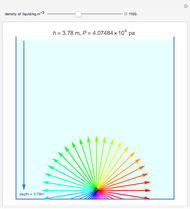
In symbols  , where
, where  is the pressure,
is the pressure,  is the density (of water, in this case),
is the density (of water, in this case),  is gravitational acceleration,
is gravitational acceleration,  is the height, and
is the height, and  is the velocity. In the graphic, the subscript 1 denotes quantities on the left and right sides of the pipe.
is the velocity. In the graphic, the subscript 1 denotes quantities on the left and right sides of the pipe.
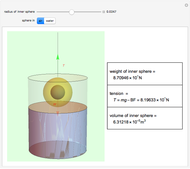
The arrow that represents  is drawn at one-tenth its real size because it grows too much compared to
is drawn at one-tenth its real size because it grows too much compared to  .
.
Permanent Citation