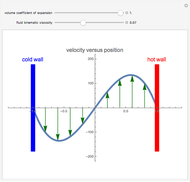
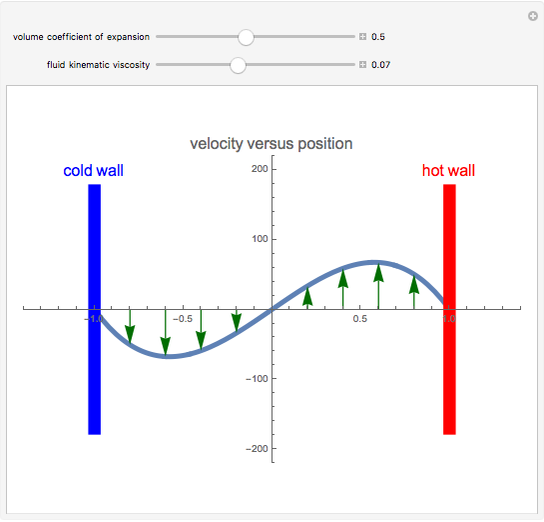
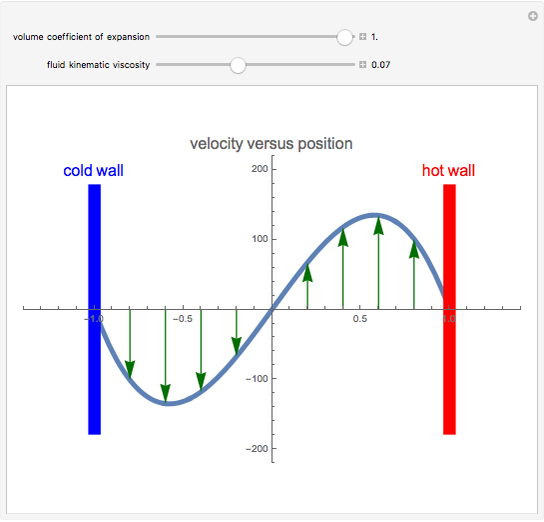
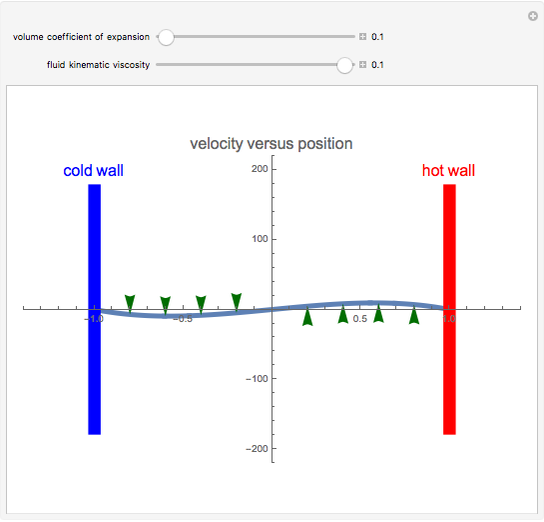
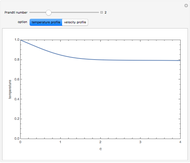
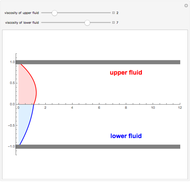
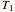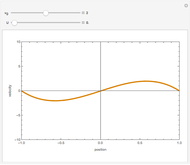
Two parallel walls are maintained at uniform temperatures (a hot temperature, , and a cold temperature, 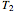). You can set the viscosity and volume coefficient of expansion of the fluid that fills the space between the two walls. The steady state velocity profile of the resulting natural convection is displayed.
The temperature variation in the direction is assumed to be linear:
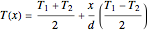, where 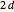is the space between the two walls.
The density is not constant and varies according to 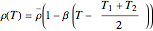, where is the density at the mean temperature, .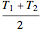
The governing equation is given by .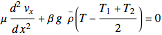
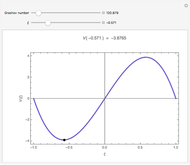
It is possible to derive an analytical expression of the dimensionless velocity using the built-in Mathematica function DSolve:
,
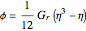where is the dimensionless velocity, the dimensionless length, and 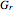the Grashof number, a dimensionless number that arises in problems on free convection.
![[Snapshot]](HTMLImages/index.en/thumbnail_1.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_2.gif)
![[Snapshot]](HTMLImages/index.en/thumbnail_3.gif)
Embed Interactive Demonstration 
Browse all topics
