Rational Linear Combinations of Pure Geodetic Angles, Part 2

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
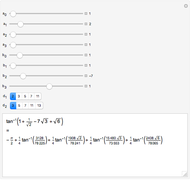
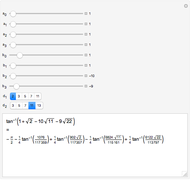
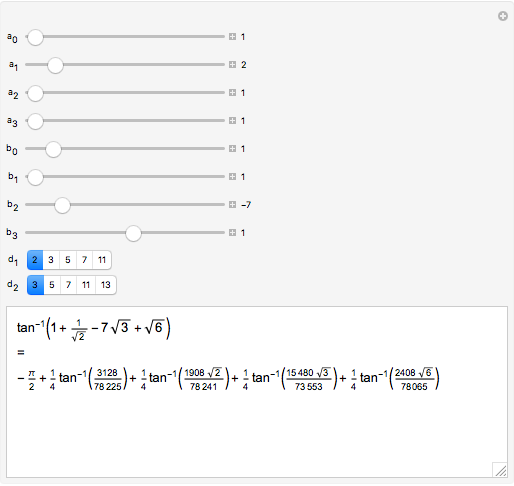
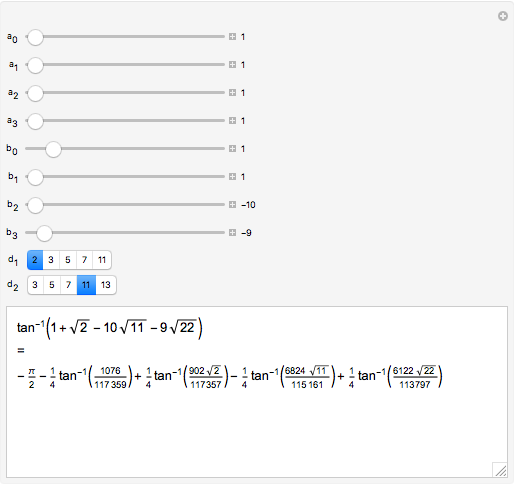
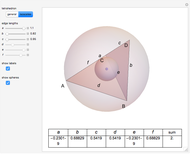
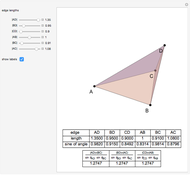
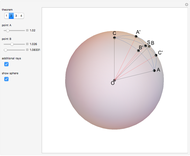
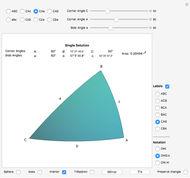
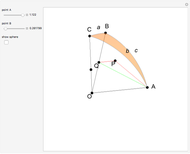
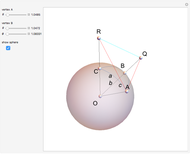
A "pure geodetic" angle is an angle  such that any of the six squared trigonometric functions of
such that any of the six squared trigonometric functions of  is rational or infinite. This Demonstration shows how an angle whose tangent is of the form
is rational or infinite. This Demonstration shows how an angle whose tangent is of the form 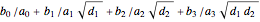 can be expressed as a rational linear combination of pure geodetic angles and an integral multiple of
can be expressed as a rational linear combination of pure geodetic angles and an integral multiple of 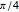 , that is, it finds rational
, that is, it finds rational 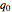 ,
, 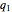 ,
,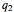 , and
, and 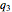 , such that
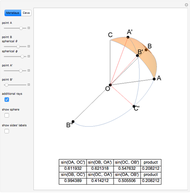
, such that 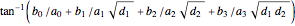 is a sum of a rational linear combination of
is a sum of a rational linear combination of 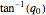 ,
, 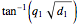 ,
, 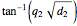 , and
, and 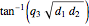 plus an integer multiple of
plus an integer multiple of 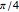 .
.
Contributed by: Izidor Hafner (March 2011)
Open content licensed under CC BY-NC-SA
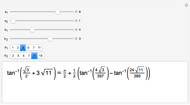
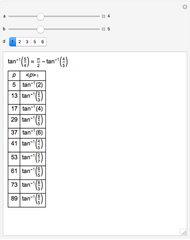
Snapshots
Details
Reference
[1] J. H. Conway, C. Radin, and L. Sadun, "On Angles Whose Squared Trigonometric Functions Are Rational," Discrete & Computational Geometry, 22(3), 1999 pp. 321–332.
Permanent Citation
"Rational Linear Combinations of Pure Geodetic Angles, Part 2"
http://demonstrations.wolfram.com/RationalLinearCombinationsOfPureGeodeticAnglesPart2/
Wolfram Demonstrations Project
Published: March 7 2011