Relaxation Method for Electrostatic Problems
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
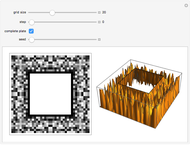
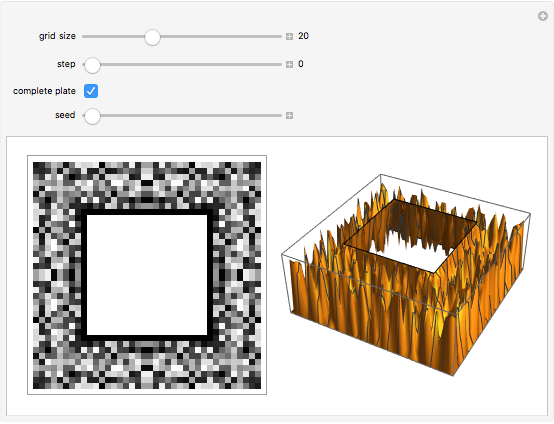
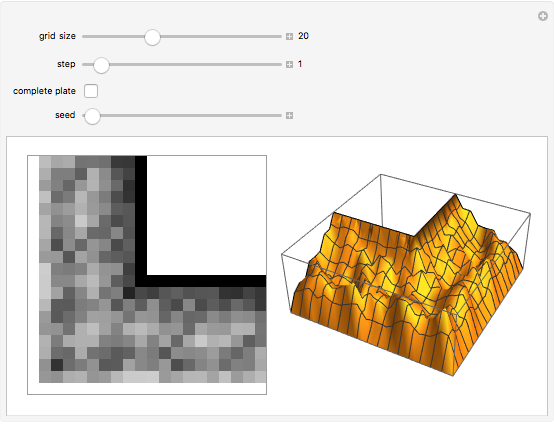
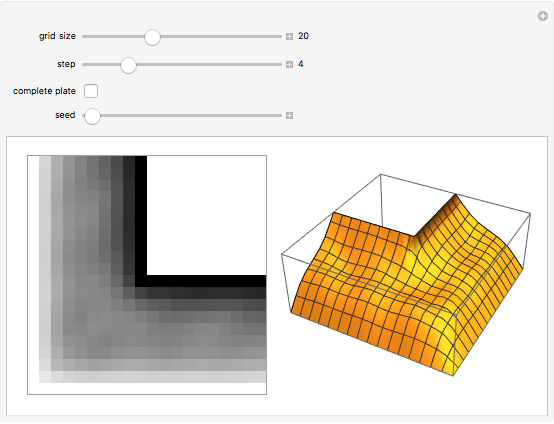
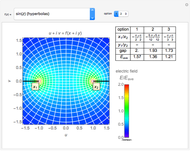
We use the relaxation method to approximate solutions of Laplace's equation. Starting with a grid of points with fixed values at the boundaries, the value of the solution at a point is the mean of its four neighbors. (This can be seen with a Taylor series expansion around that point.)
[more]
Contributed by: Enrique Zeleny (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Permanent Citation