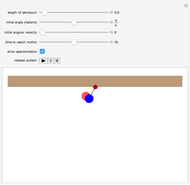
Series Approximation for the Nonlinear Pendulum

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
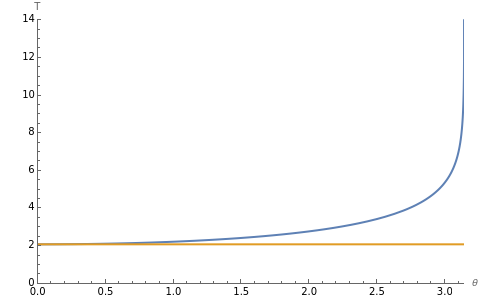
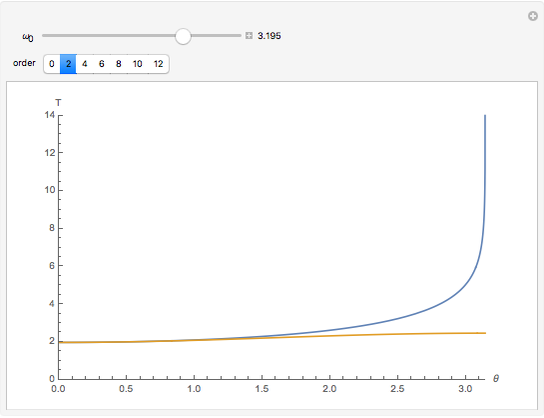
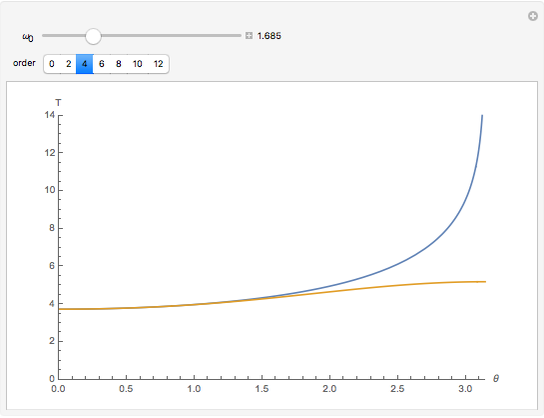
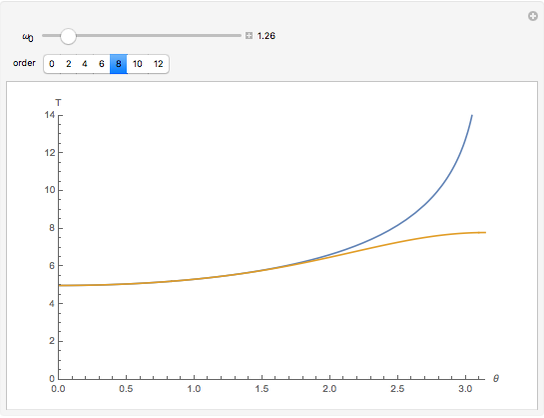
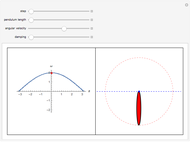
The plot shows the difference between the closed form of the solution for the nonlinear pendulum (in blue), and the series approximation for the corresponding elliptic integral of the first kind 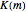 , plotting the initial amplitude
, plotting the initial amplitude  versus the oscillation period
versus the oscillation period 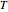 , for a given frequency
, for a given frequency 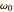 . The nonlinear oscillation arises when no assumption of small oscillations is made; that is, the approximation
. The nonlinear oscillation arises when no assumption of small oscillations is made; that is, the approximation 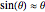 is not assumed here.
is not assumed here.
Contributed by: Enrique Zeleny (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The elliptic integral of the first kind 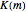 is represented in Mathematica by EllipticK.
is represented in Mathematica by EllipticK.
The complete analytical treatment can be found in G. Baumann, Mathematica for Theoretical Physics, New York: Springer, 2nd. ed., 2005.
Permanent Citation