Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

A Concurrency from Midpoints of Arcs of the Circumcircle

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

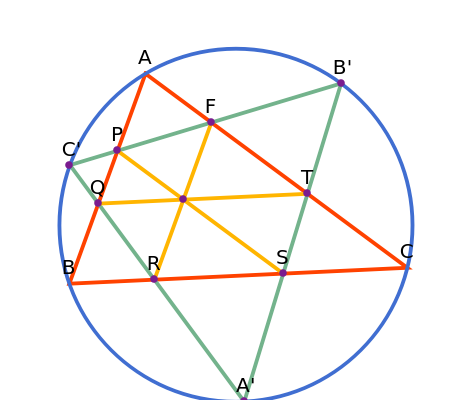
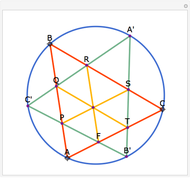
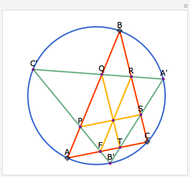
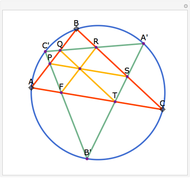
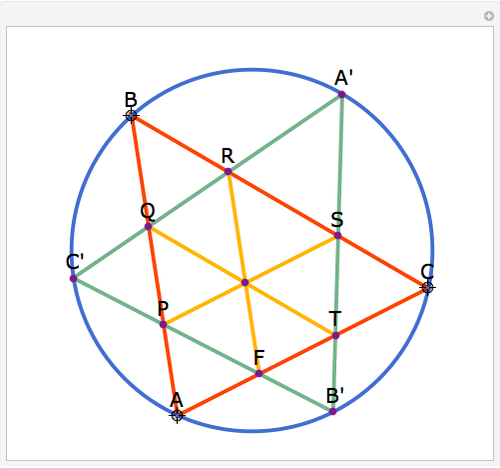
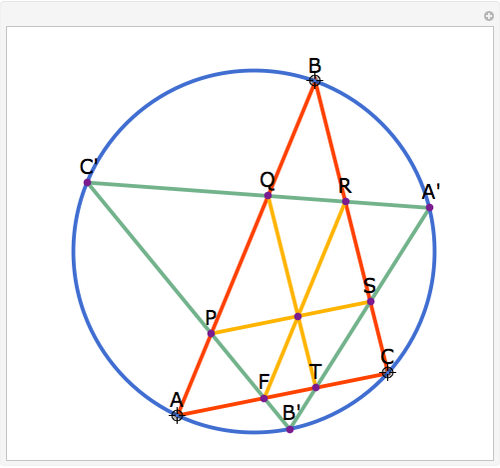
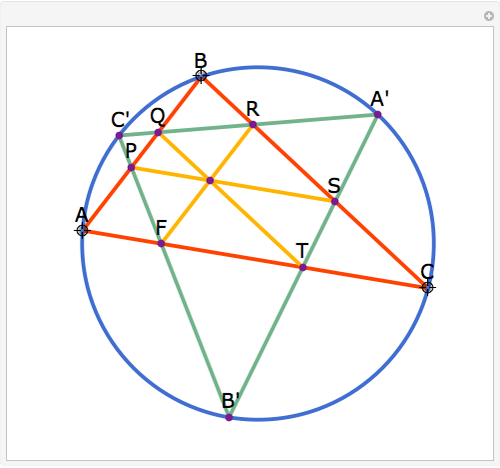
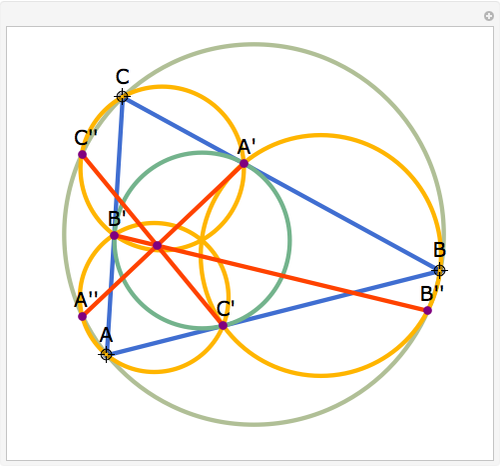
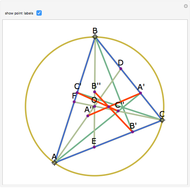
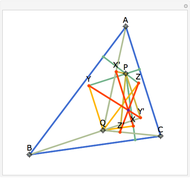
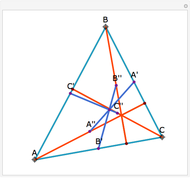
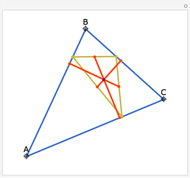
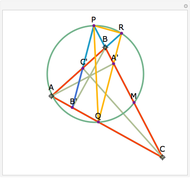
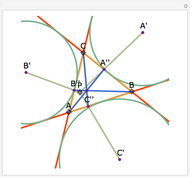
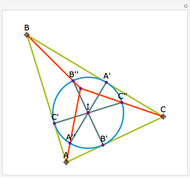
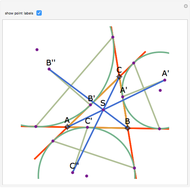
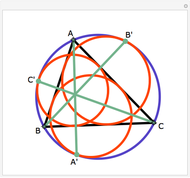
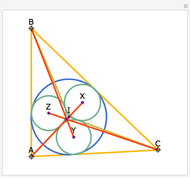
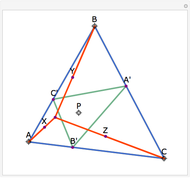
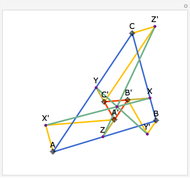
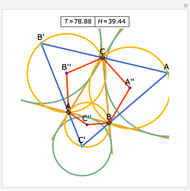
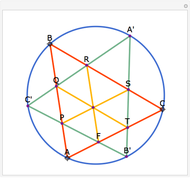
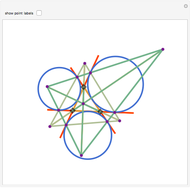
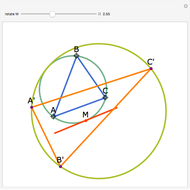
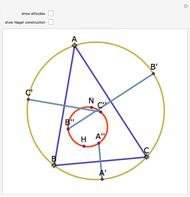
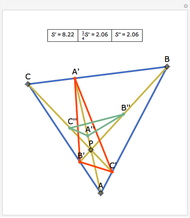
Let ABC be a triangle. Let A', B', and C' be the midpoints of the arcs BC, CA, and AB of the circumcircle of ABC, respectively. Let A'B' meet BC and AC at S and T, B'C' meet AC and AB at F and P, and C'A' meet AB and BC at Q and R. Then PS, QT, and FR are concurrent.

Contributed by: Jay Warendorff (October 2008)
Open content licensed under CC BY-NC-SA

Snapshots

Details

See Harder Problems 1, Collinearity and Concurrence by Po-Shen Loh.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send