Addition of Angular Momenta in Quantum Mechanics

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
Angular momentum in quantum mechanics is a quantized vector with magnitude  and component
and component  in any direction, conventionally chosen as the
in any direction, conventionally chosen as the  axis. The quantum numbers are restricted to integer or half-integer values:
axis. The quantum numbers are restricted to integer or half-integer values:  , with
, with  . Vector addition of two angular momenta
. Vector addition of two angular momenta  is restricted by a triangle inequality
is restricted by a triangle inequality  with
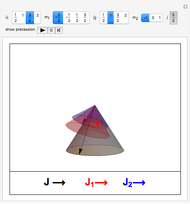
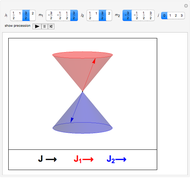
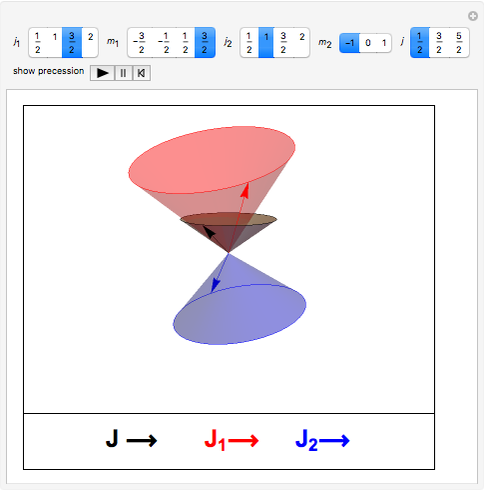
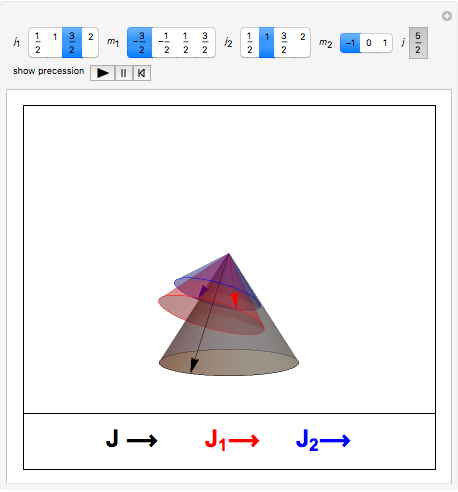
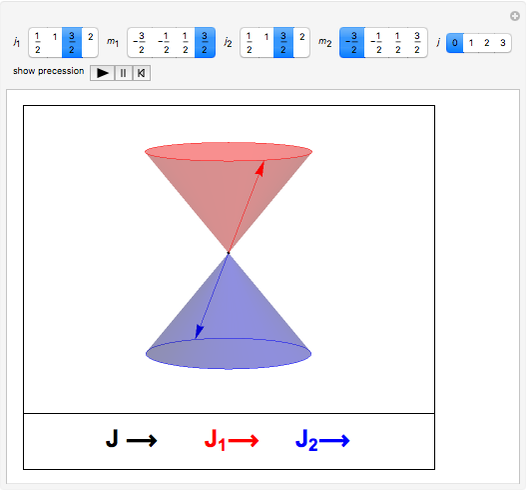
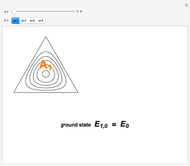
with  . Although quantum formalism is indifferent to such interpretations, the addition of angular momentum in the absence of any electric or magnetic field can be pictured by a vector model in which
. Although quantum formalism is indifferent to such interpretations, the addition of angular momentum in the absence of any electric or magnetic field can be pictured by a vector model in which  and
and  precess about
precess about  , which itself precesses about a
, which itself precesses about a  axis. The amplitude for addition of
axis. The amplitude for addition of  and
and  to give
to give  with component
with component  can be expressed in terms of Clebsch–Gordan coefficients as
can be expressed in terms of Clebsch–Gordan coefficients as  with the sum restricted by
with the sum restricted by  . You can set the precessions into motion with the trigger control. To choose a new set of
. You can set the precessions into motion with the trigger control. To choose a new set of  and
and  values, pause and reset the trigger.
values, pause and reset the trigger.
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
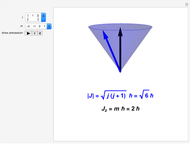
Snapshots
Details
Reference: S. M. Blinder, Introduction to Quantum Mechanics, Amsterdam: Elsevier, 2004 pp. 85–86.
Permanent Citation
"Addition of Angular Momenta in Quantum Mechanics"
http://demonstrations.wolfram.com/AdditionOfAngularMomentaInQuantumMechanics/
Wolfram Demonstrations Project
Published: March 7 2011