Comparing Exact and Approximate Censored Normal Likelihoods

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
A random sample of size  is simulated from a left-censored standard normal distribution with censor point
is simulated from a left-censored standard normal distribution with censor point  , where
, where  is the censor rate and
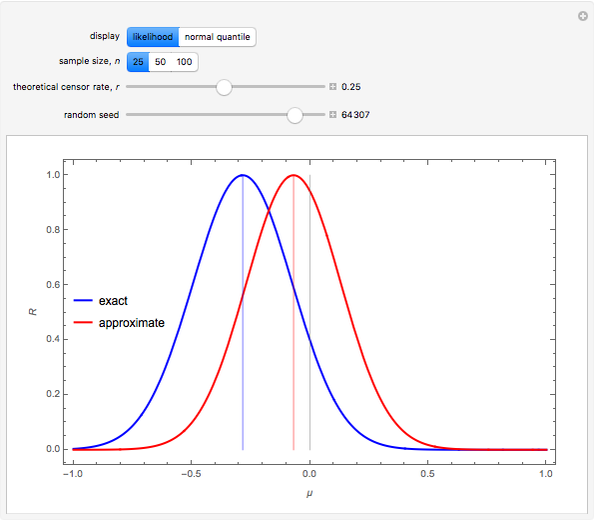
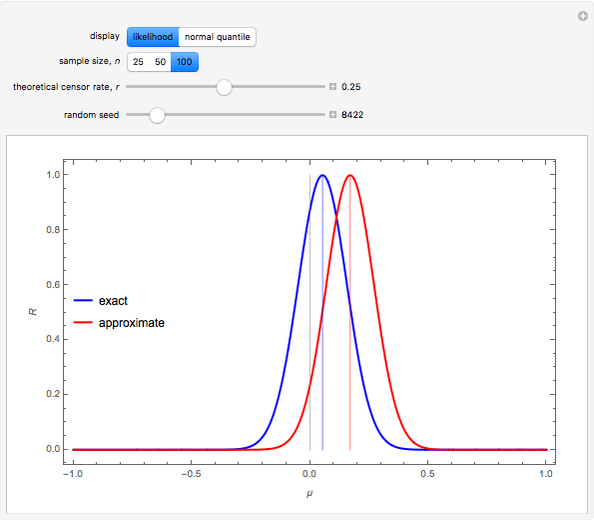
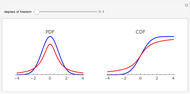
is the censor rate and  is the inverse cumulative distribution function of the standard normal distribution. The Demonstration shows the exact relative likelihood function for the mean of the underlying distribution (blue curve) as well as a crude approximation (red curve) that ignores censoring. When
is the inverse cumulative distribution function of the standard normal distribution. The Demonstration shows the exact relative likelihood function for the mean of the underlying distribution (blue curve) as well as a crude approximation (red curve) that ignores censoring. When  is close to zero, the crude approximation is reasonable, but as
is close to zero, the crude approximation is reasonable, but as  increases, the exact method becomes more and more accurate. The blue and red lines show corresponding point estimates for the exact and approximate estimates. while the gray line shows the true parameter value. By varying the random seed, you see the stochastic nature of estimates; for example, sometimes the crude estimator may be more accurate, even though overall, on average, it is less accurate when
increases, the exact method becomes more and more accurate. The blue and red lines show corresponding point estimates for the exact and approximate estimates. while the gray line shows the true parameter value. By varying the random seed, you see the stochastic nature of estimates; for example, sometimes the crude estimator may be more accurate, even though overall, on average, it is less accurate when  .
.
Contributed by: Ian McLeod and Nagham Muslim Mohammad (July 2013)
Department of Statistical and Actuarial Sciences, Western University
Open content licensed under CC BY-NC-SA
Snapshots
Details
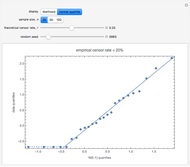
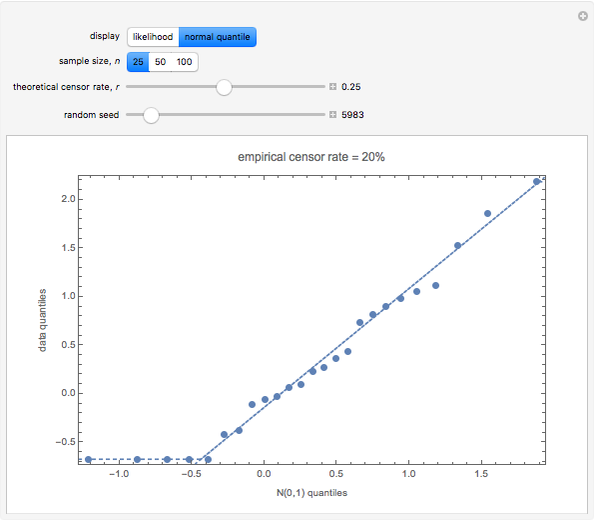
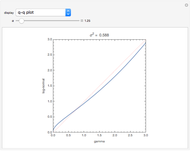
Snapshot 1. The quantile plot of the data used in the thumbnail is shown. From the plot,  and there were five censored values, so the empirical censor rate was 20%.
and there were five censored values, so the empirical censor rate was 20%.
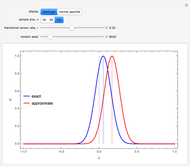
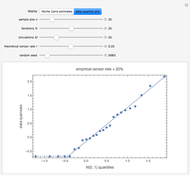
Snapshot 2. Even though the crude estimator is always statistically less efficient, it can happen by chance that the crude approximation is more accurate than the exact maximum likelihood method as shown in snapshot 2. A simulation experiment found that this happens about 43% of the time with  even though the crude estimator has only about 72% efficiency. As
even though the crude estimator has only about 72% efficiency. As  or
or  increases, the efficiency of the crude estimator decreases.
increases, the efficiency of the crude estimator decreases.
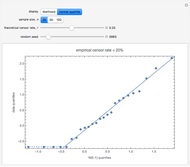
Snapshot 3. The sample size is increased to 100 and the resulting relative likelihoods become narrower, indicating the increase in precision.
The right-censored case is discussed in [1] and the results for the left-censored case may be derived in a similar way. As in [1], the EM algorithm [1] is used to compute the exact maximum likelihood estimate. For computation efficiency, Mathematica's built-in function Compile is used. The relative likelihood  is the likelihood rescaled so its maximum value is 1.0. It can be used directly for statistical inference [2].
is the likelihood rescaled so its maximum value is 1.0. It can be used directly for statistical inference [2].
References
[1] C. P. Robert and G. Casella, Monte Carlo Statistical Methods, 2nd ed., New York: Springer, 2004.
[2] Wikipedia. "Likelihood Function." (Jul 2, 2013) en.wikipedia.org/wiki/Likelihood_function# Relative_likelihood.
Permanent Citation