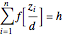Congressional Apportionment Using General Divisor Methods

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
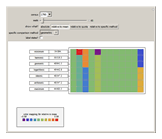
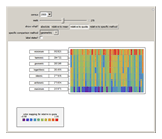
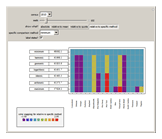
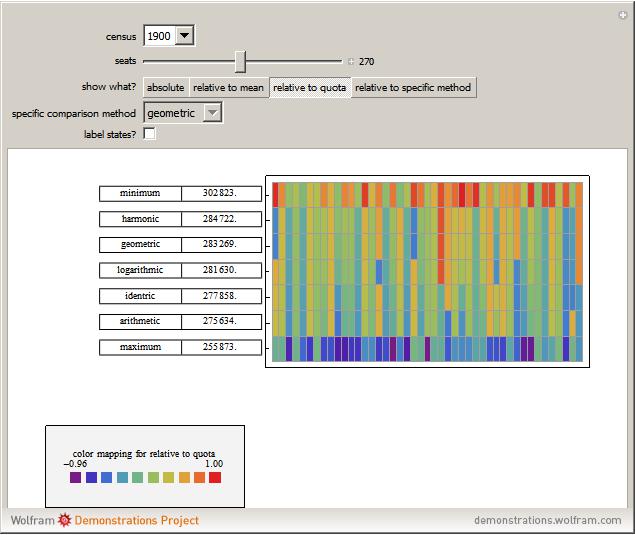
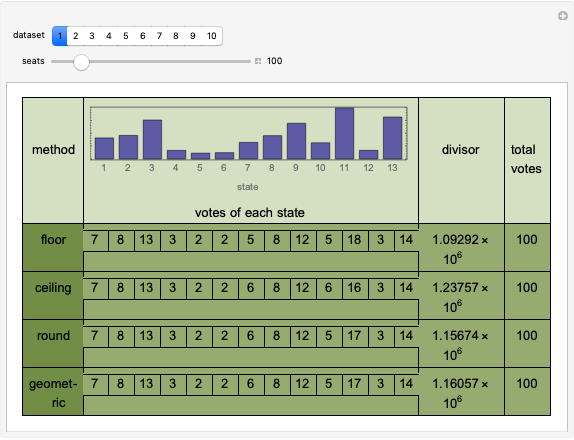
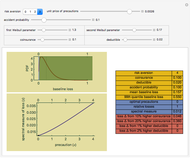
The United States Constitution states that representatives in the House of Representatives "shall be apportioned among the several States according to their respective numbers, counting the whole number of persons in each State, excluding Indians not taxed" (14th amendment, section 2). The Constitution further places a ceiling on the number of total representatives at 1 per 30,000 of the total population and states that every state shall have at least one member in the House of Representatives (Article I, section 2). The Constitution does not specify, however, how to deal with the issue of rounding in the resulting computations.
[more]
Contributed by: Seth J. Chandler (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
The data for this article was compiled by the author using publicly available census data, publicly available data on the times at which the various states were admitted to the United States, and using Mathematica to manipulate that data as needed. As specified by the United States Constitution until the ratification of the 14th amendment in 1868, slaves count as only 3/5 of a person for purposes of state representation in the House of Representatives.
An article on general divisor methods may be found in E. Park, "The Mathematics of Apportionment", The University of Chicago Law School Roundtable, 7, 2000 pp. 227–235. An article on alternative rounding methods and the relationship of the divisor problem to constrained optimization may be found in R. Agnew, "Optimal Congressional Apportionment", The American Mathematical Monthly, 115, 2008 pp. 297–303. This Demonstration draws significantly on both of these works.
The "floor" method is essentially that of Thomas Jefferson and was used from 1790 through 1830. The "round" method is essentially that proposed by Daniel Webster and was used in 1840 and again with minor variation in 1910 and 1930. The geometric method is roughly what is used today. The identric mean and logarithmic methods have newly been suggested but have never been adopted.
Permanent Citation
"Congressional Apportionment Using General Divisor Methods"
http://demonstrations.wolfram.com/CongressionalApportionmentUsingGeneralDivisorMethods/
Wolfram Demonstrations Project
Published: March 7 2011