Wolfram Demonstrations Project

12,000+ Open Interactive Demonstrations
Powered by Notebook Technology »

Current versus Cohort Life Tables and the Regulation of Life Insurance

Initializing live version
Download to Desktop

Requires a Wolfram Notebook System

Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.

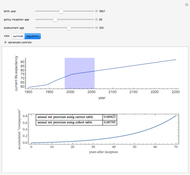
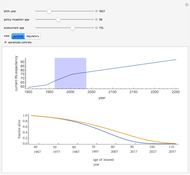
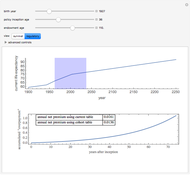
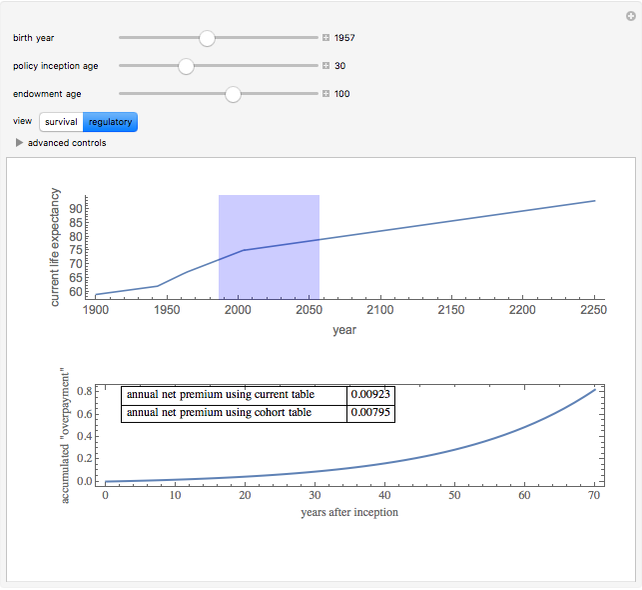
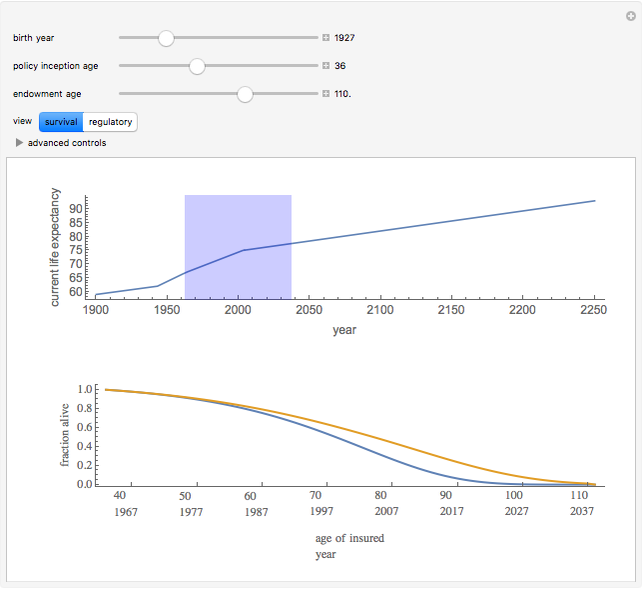
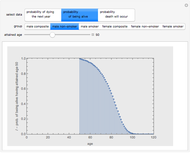
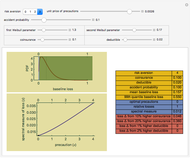
Mortality tables generally used in life insurance regulation and many life insurance computations are what are called "current tables": they show the rates of death for each age at some point in time, such as 1980 or 2003. Individuals do not go through their lives, however, as if it were always 1980 or 2003. Thus, an individual born in 1957 dies at the rate for 53-year-olds set forth by 2010 current mortality tables, dies at the rate for 63-year-olds set forth by 2020 current mortality tables, and dies at the rate for 73-year-olds set forth by 2030 current mortality tables. The death rates that this individual thus experiences can be recapitulated in what is known as a "cohort life table."

[more]

This Demonstration permits you to generate cohort life tables and compare them with current life tables. It further shows the misestimation of premiums that can result from use of current life tables, as well as the resulting potential for seriously erroneous computation of insurance "reserves".

Using the locators in the top graphic, you determine how mean life expectancy changes over time. You select the birth year of the insured, the age at which an insured's whole life insurance policy incepts, and the "endowment age" of the policy, i.e. an age when the insured is automatically deemed "dead" for life insurance purposes. In "survival view", you see a comparison of the survival curves when the "hazard function" is fixed at the inception of the policy (current) and when the "hazard function" evolves with time (cohort). In regulatory view, you see a graphic showing the relationship between the age of the policy and the amount by which the future value of the accumulated premiums that have been paid using current tables exceeds the future value of accumulated premiums that would have been paid had a cohort table been used. An inset compares these two premium values. As shown by the Demonstration, this difference can grow to quite large amounts under certain conditions.

Policies are assumed to pay one unit ($) at the end of the policy year in which death occurs. Premiums are due at the beginning of each policy year.

[less]

Contributed by: Seth J. Chandler (March 2011)
Open content licensed under CC BY-NC-SA

Snapshots

Details

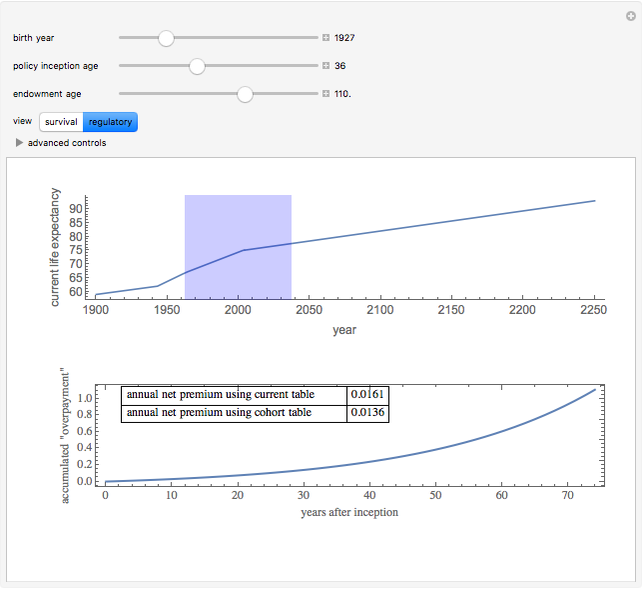
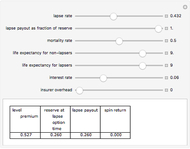
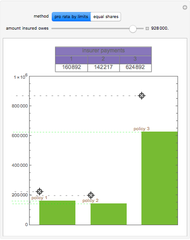
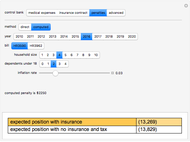
So long as life expectancy increases over time, the premiums derived using a current table will be higher than those using a cohort table. Insurers adopt different contractual rules and policies within constraints provided by regulations regarding how the difference between the accumulated premiums that result is allocated among insurer and insureds. The insurer, for example, may pay a dividend to insureds to compensate them for mortality better than that predicted by current tables.

This Demonstration assumes a two-parameter Gompertz–Makeham hazard function in which the second parameter remains constant and life expectancy is changed through alteration of the first parameter. You can change the second parameter of the distribution with the advanced controls. A second advanced control lets you change the interest rate used by the insurer in computing premiums and the future value of the difference between premiums under a cohort life table and a current life table.

To permit free movement of the locators but ensure the amount of data necessary to perform the computations required by this Demonstration, you are barred from having fewer than two locators, and it is additionally assumed that life expectancy was 30 in the year 0 and will be 100 in the year 5000.

For simplicity, it is assumed that policies incept on January 1st of each year. Nothing material would change in the outputs of this Demonstration were this assumption relaxed.

A true cohort life table cannot be fully constructed until all the persons born in some cohort die.

An excellent treatment of the mathematics of life insurance, including the "commutation functions" used in the code for this Demonstration, may be found in H. U. Gerber, Life Insurance Mathematics, 3rd ed., New York: Springer, 1997.

Embed Code

Take advantage of the Wolfram Notebook Emebedder for the recommended user experience.

Related Demonstrations More by Author
Browse all topics
Feedback (field required)
Email (field required) Name
Occupation Organization
Note: Your message & contact information may be shared with the author of any specific Demonstration for which you give feedback.
Send