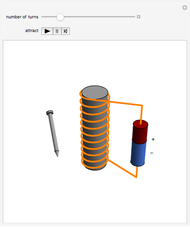
Einstein-de Haas Effect

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
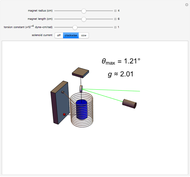
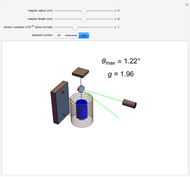
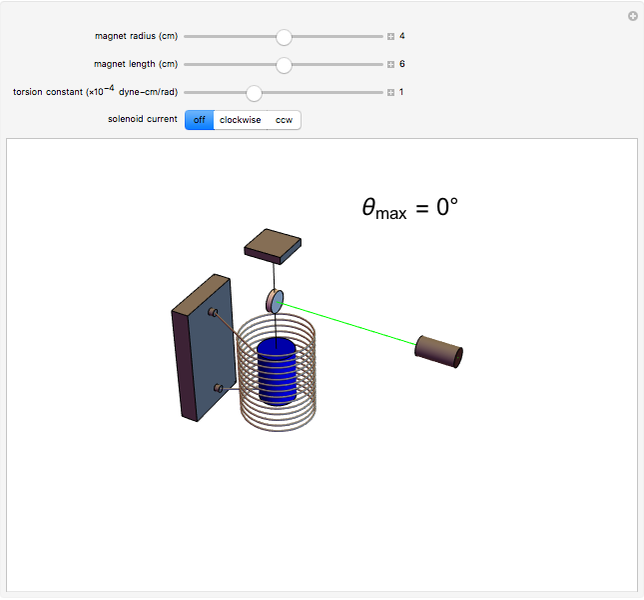
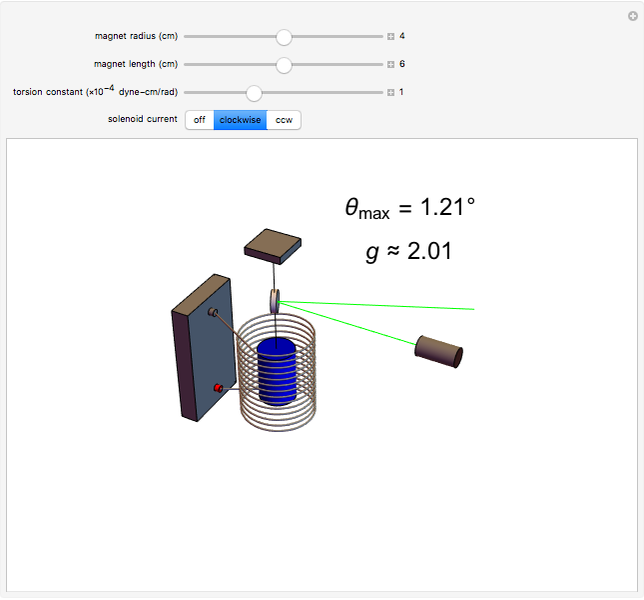
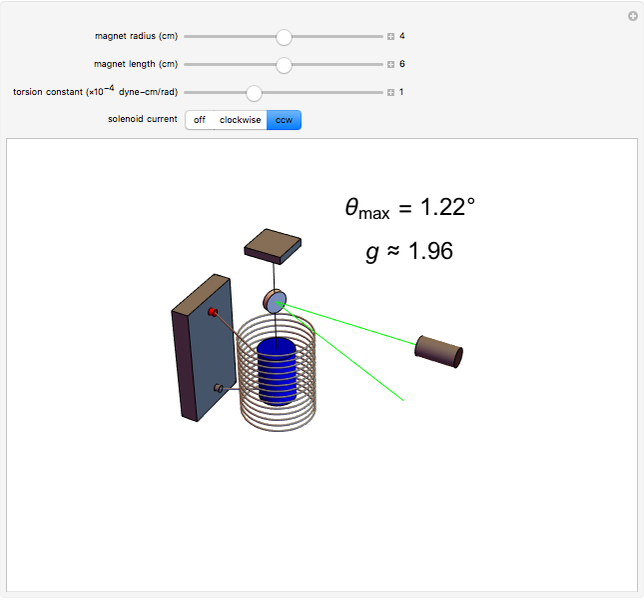
The only experimental work ever published by Albert Einstein was carried out in 1915 in collaboration with Wander Johannes de Haas, who was the son-in-law of H. A. Lorentz. Einstein had long contemplated Ampère's conjecture in 1820 that magnetism is caused by circulation of electric charge. This Demonstration describes a technologically updated version of the original Einstein–de Haas experiment. A cylindrical soft-iron magnet is supported by a thin quartz fiber attached to a mirror. The magnet is suspended within a solenoid connected to a reversible DC power source. The current is large enough to create a magnetic field strong enough to saturate the cylinder's magnetization in either direction. If Ampère was right, this should create an angular displacement of the magnet, which can be detected by deflection of a laser beam directed at the rotating mirror.
[more]
Contributed by: S. M. Blinder (March 2011)
Open content licensed under CC BY-NC-SA
Snapshots
Details
 An iron cylinder with a saturation magnetization of
An iron cylinder with a saturation magnetization of  gauss has a net magnetic dipole moment of
gauss has a net magnetic dipole moment of  directed along the axis of the solenoid. If this magnetic dipole is associated with charge circulation, then
directed along the axis of the solenoid. If this magnetic dipole is associated with charge circulation, then  , where
, where  is the angular momentum of the magnet and
is the angular momentum of the magnet and  is the magnetogyric ratio. The maximum kinetic energy of rotation equals
is the magnetogyric ratio. The maximum kinetic energy of rotation equals  , where
, where  is the moment of inertia of the magnet. This is converted into potential energy of the torsional balance at its maximum angular displacement, given by
is the moment of inertia of the magnet. This is converted into potential energy of the torsional balance at its maximum angular displacement, given by  . From the preceding formulas, the
. From the preceding formulas, the  -factor is determined by
-factor is determined by  , where
, where  InlineMath (expressed in degrees) is the maximum deflection of the reflected laser beam. Reversing the magnetization of the iron should create an angular displacement in the opposite direction as the angular momentum of the charge carriers is reversed.
InlineMath (expressed in degrees) is the maximum deflection of the reflected laser beam. Reversing the magnetization of the iron should create an angular displacement in the opposite direction as the angular momentum of the charge carriers is reversed.
Reference: Einstein–de Haas effecton Wikipedia.
Permanent Citation