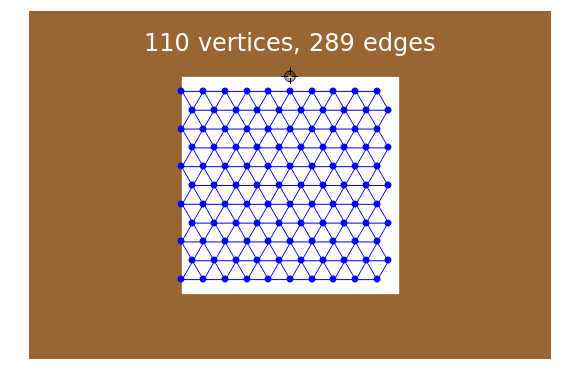
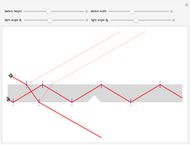
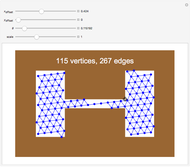
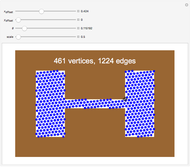
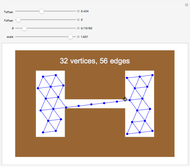
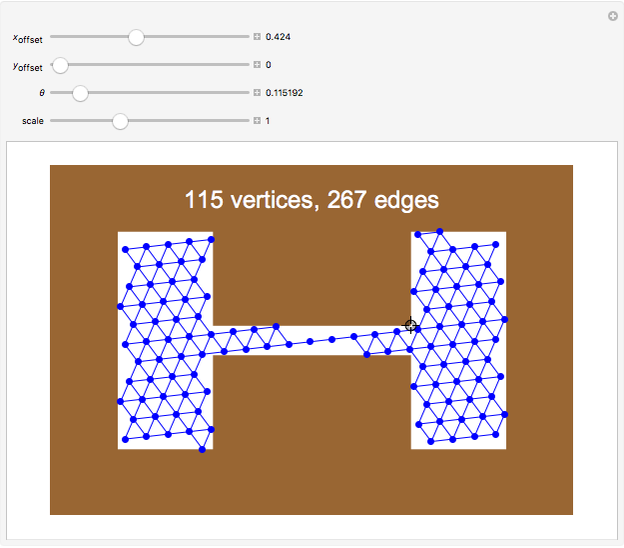
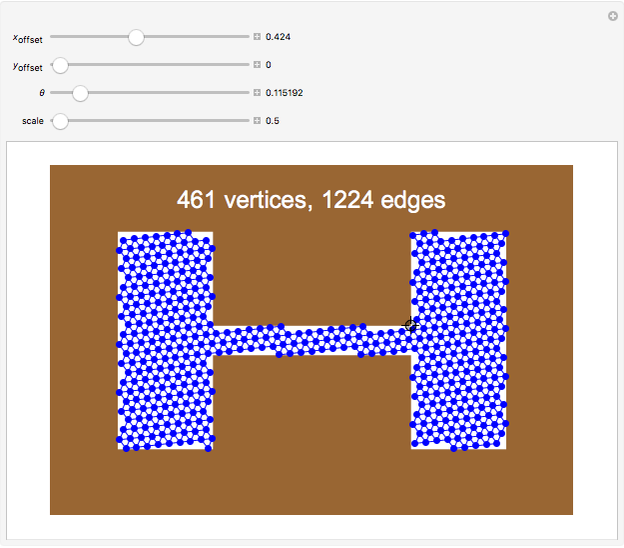
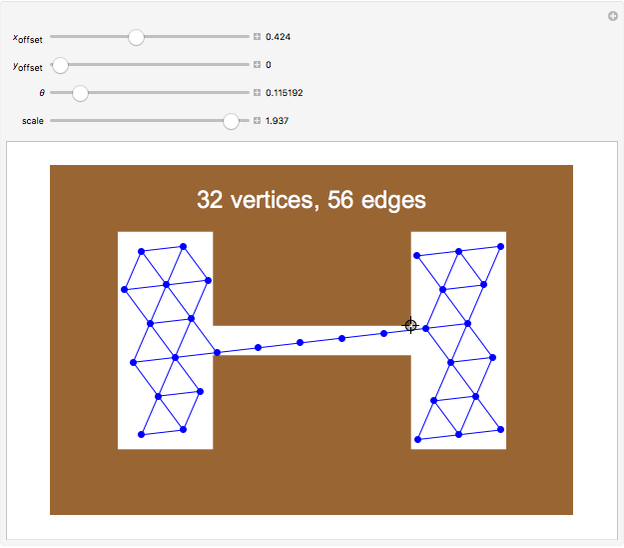
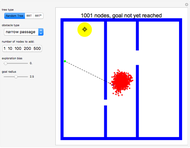
An equilateral triangular lattice is parameterized by four variables: an and offset for the initial vertex, the angle of the grid with respect to the horizontal, and a scale parameter for the edge lengths. In this Demonstration, you can vary these parameters and see how many vertices and edges fit in the open workspace (white). Drag the locator to create a "narrow passage," while keeping the area of the workspace constant.