Molecular Graph Theory Applied to Fullerenes

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
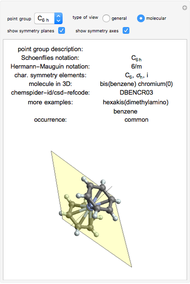
Chemical graph theory classifies molecules using a topological characterization of their chemical structures. The aim is to model new structures with predictable properties.
[more]
Contributed by: Guenther Gsaller (July 2012)
(Institute of Organic Chemistry, Johannes Kepler University, Linz, Austria, http://www.jku.at/orc/)
Open content licensed under CC BY-NC-SA
Snapshots
Details
In 1985, Kroto, Heath, O'Brien, Curl, and Smalley suggested the  structure to explain the pronounced abundance of the
structure to explain the pronounced abundance of the  cluster in their graphite laser experiment. A confirmation came in 1990, when a method for bulk production including IR
spectroscopic evidence was delivered by Krätschmer, Lamb, Fostiropoulos, and Huffman. Starting with these two papers, fullerene research exploded.
cluster in their graphite laser experiment. A confirmation came in 1990, when a method for bulk production including IR
spectroscopic evidence was delivered by Krätschmer, Lamb, Fostiropoulos, and Huffman. Starting with these two papers, fullerene research exploded.
Theoretical tools are used in modern chemistry to develop molecular models of chemical reactions, processes involving physics and chemistry, events throughout medicine, and toxicology. One aim is to find a quantitative structure-property relationship (QSPR) between the property, e.g. melting point, and the structure of the molecule.
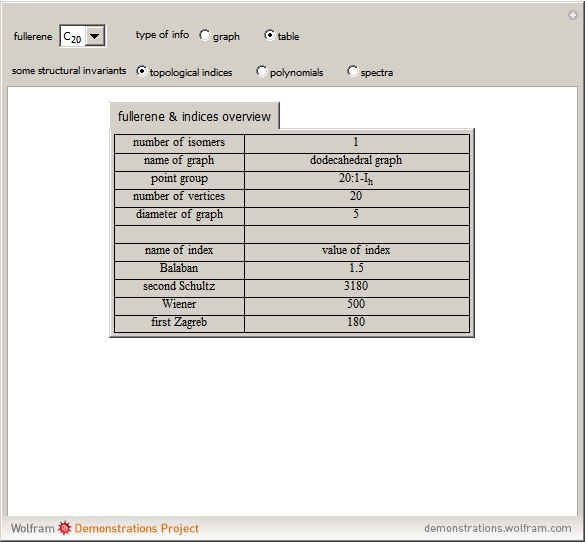
Molar graphs can represent the structural formula of a molecule. Topological indices, polynomials, and spectra of molar graphs are so-called structural invariants.
Two examples for QSPRs:
1. The roots of the Laplacian polynomial of a molecular graph determine the distribution function of the radius of the gyration of a molecule.
2. The min-max values of the spectrum of a molar graph can be used as indicators for graph branching or for the estimation of the HOMO-LUMO separation.
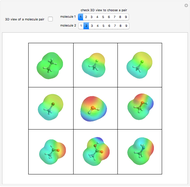
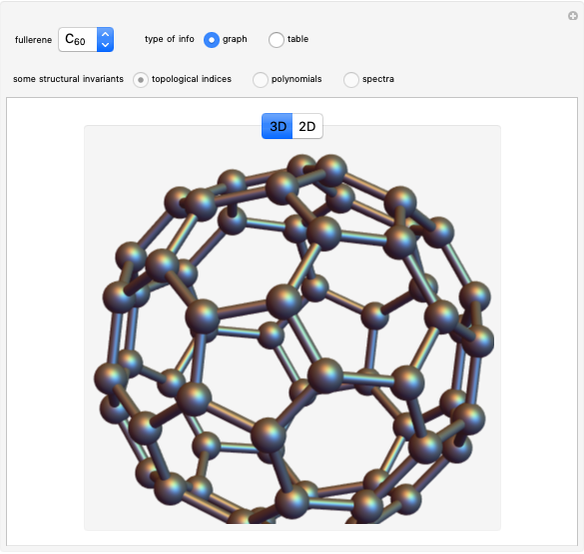
In the Demonstration, one can choose 18 fullerenes from  to
to  . The molecular graph of the fullerene can be presented as a ball-and-stick figure or a Schlegel diagram. The radio buttons can be used to switch between the results for three structural invariants in a tabular view.
. The molecular graph of the fullerene can be presented as a ball-and-stick figure or a Schlegel diagram. The radio buttons can be used to switch between the results for three structural invariants in a tabular view.
References
[1] P. W. Fowler and D. E. Manolopoulos, An Atlas of Fullerenes, Oxford: Clarendon Press, 1995.
[2] F. Cataldo, A. Graovac, and O. Ori, The Mathematics and Topology of Fullerenes, New York: Springer, 2011.
[3] M. V. Diudea, I. Gutman, and J. Lorentz, Molecular Topology, Huntington, NY: Nova Science Publishers, 2001.
[4] T. Puzyn, J. Leszczynski, and M. Cronin, Recent Advances in QSAR Studies, New York: Springer, 2010.
[5] J. Gasteiger, Handbook of Chemoinformatics, Weinheim: Wiley-VCH, 2003.
[6] O. Ivanciuc, T. Ivanciuc, and M. Diudea, "Polynomials and Spectra of Molecular Graphs," Roumanian Chemical Quarterly Reviews, 7(1), 1999 pp. 41–67. www.ivanciuc.org/Files/Reprints/p0055_rcqr_ 1999_ 7_ 41.pdf.
Permanent Citation