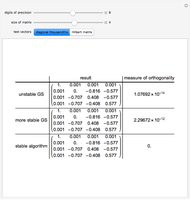
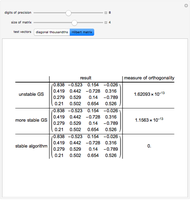
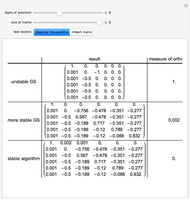
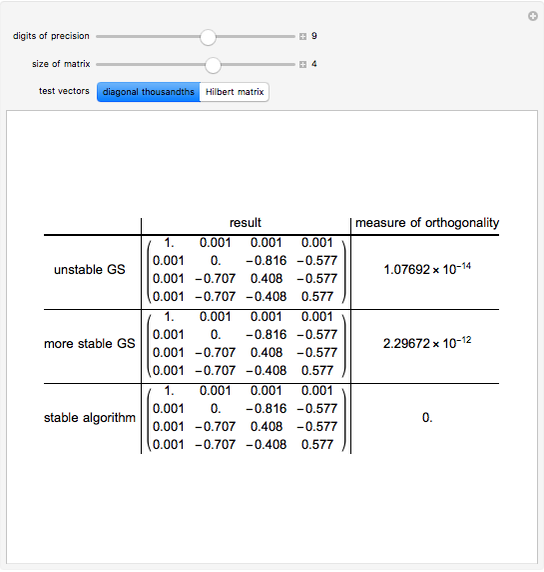
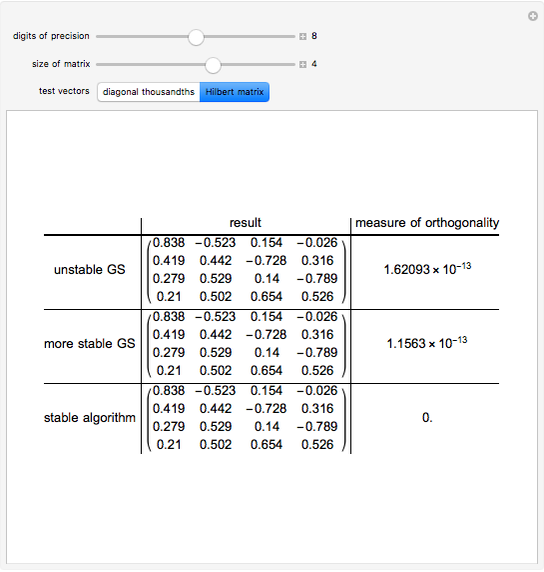
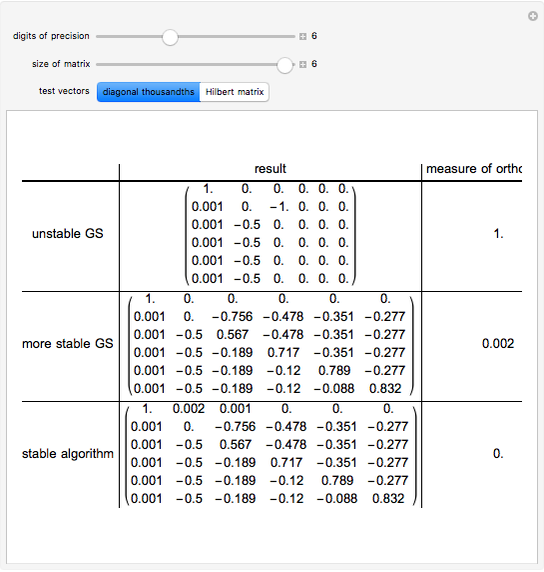
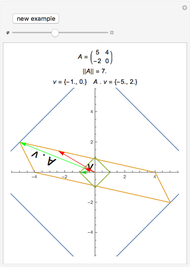
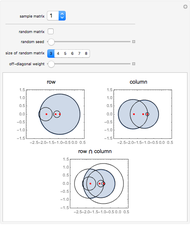
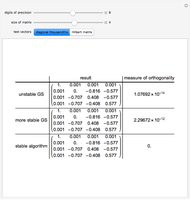
The Gram–Schmidt process is an algorithm for converting a set of linearly independent vectors into a set of orthonormal vectors with the same span. The classical Gram–Schmidt algorithm is numerically unstable, which means that when implemented on a computer, round-off errors can cause the output vectors to be significantly non-orthogonal. This instability can be improved with a small adjustment to the algorithm. This Demonstration tests the two algorithms on two families of linearly independent vectors. Selecting "diagonal thousandths" tests the algorithm on the vectors that form the columns of the matrix:
.
Selecting "Hilbert matrix" tests the algorithm on the vectors that form the columns of the
Hilbert matrix:
.
Both of these families of matrices have small but nonzero determinants, an indicator of sorts that their columns are linearly independent, but barely so. Let
be the matrix whose columns are the vectors produced by the algorithm. This Demonstration measures the orthogonality of the resulting vectors via the number
. The closer this number is to zero, the closer the columns of
are to being orthogonal.
The
Mathematica function
QRDecomposition produces an orthogonal collection of vectors from a linearly independent one in a highly stable way. The result of this function is shown at the bottom of the pane.
Browse all topics
