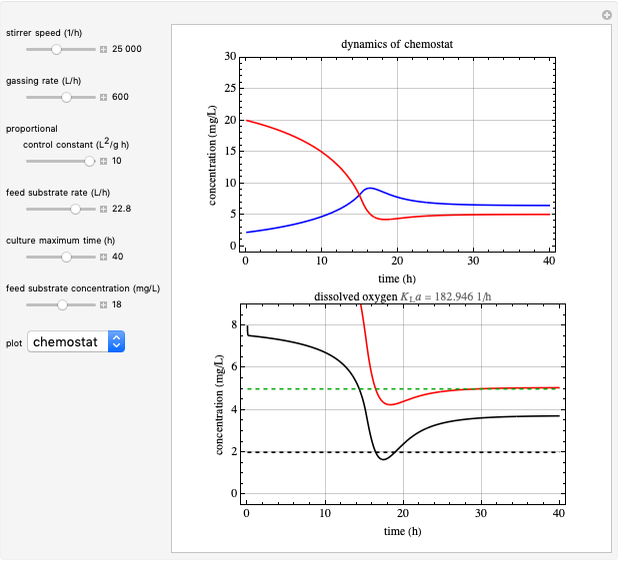
Oxygen Dynamics in a Chemostat with Substrate Inhibition

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
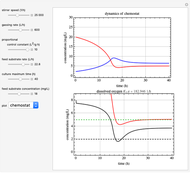
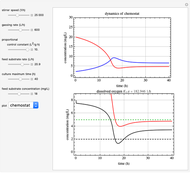
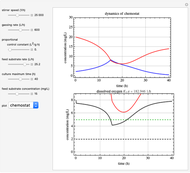
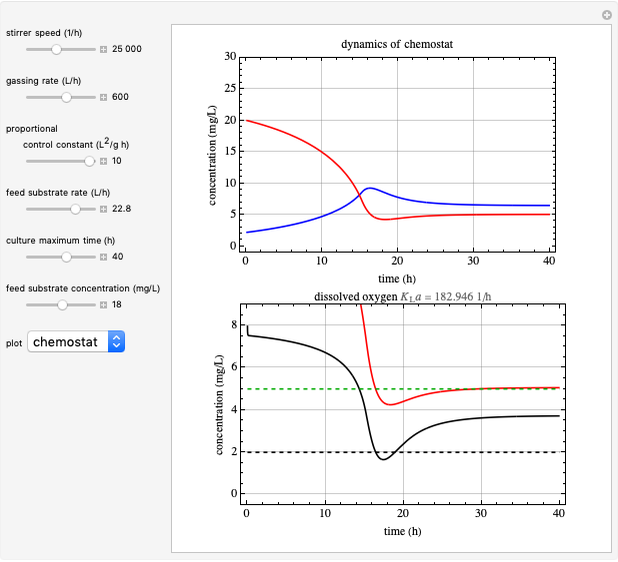
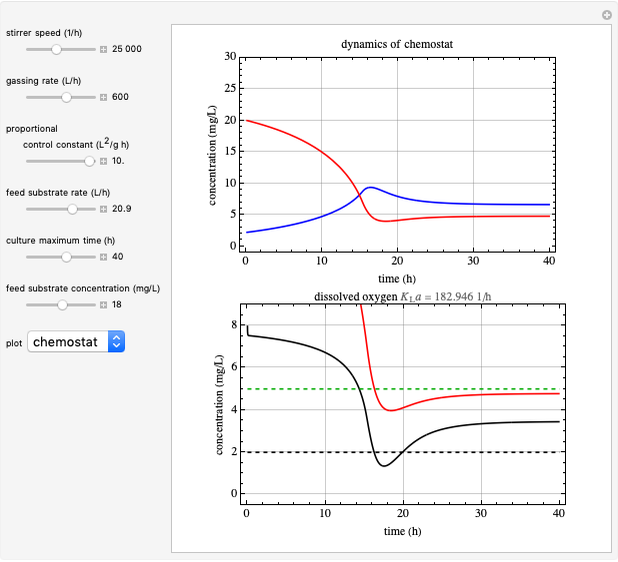
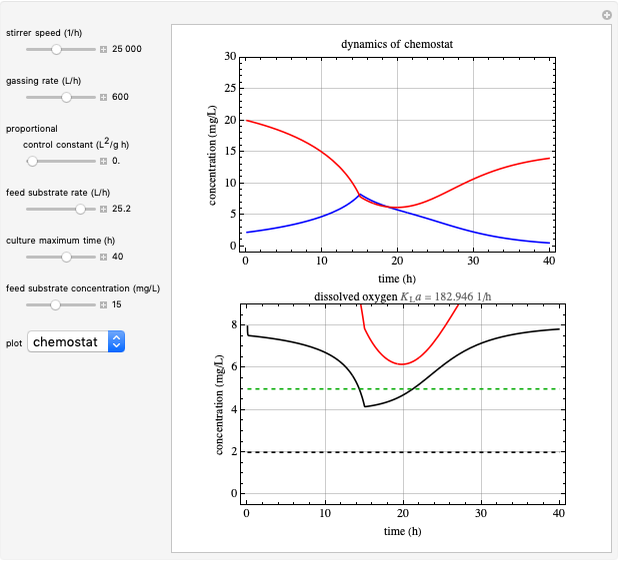
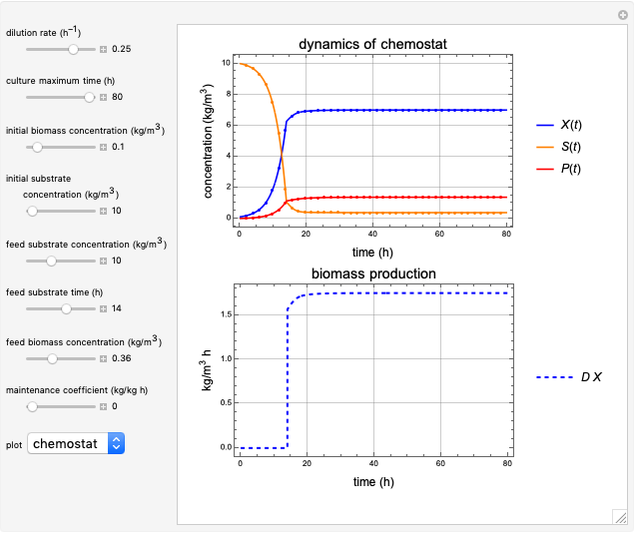
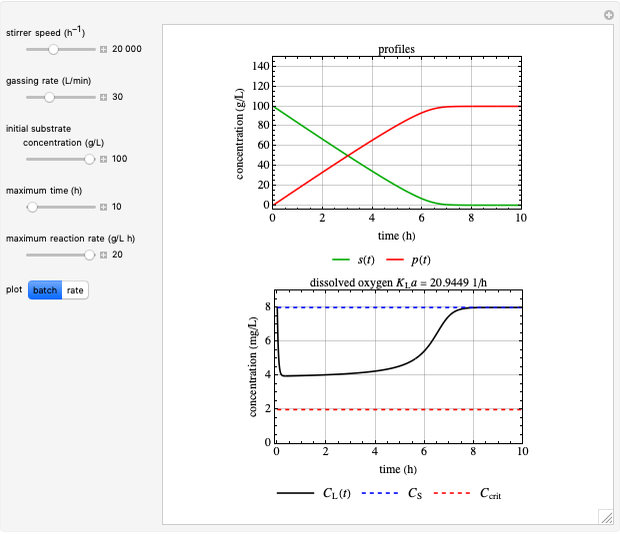
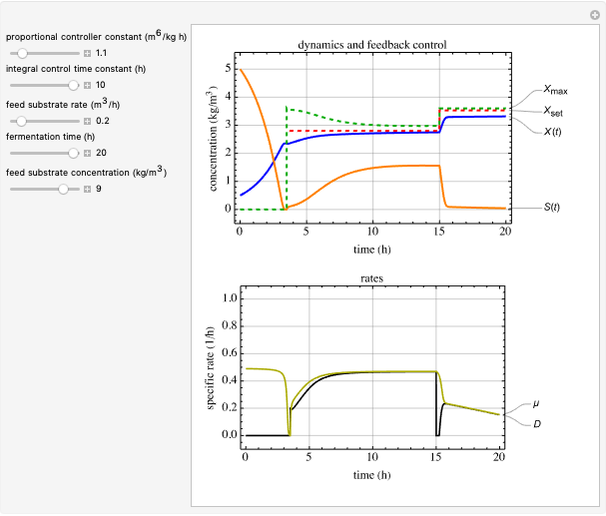
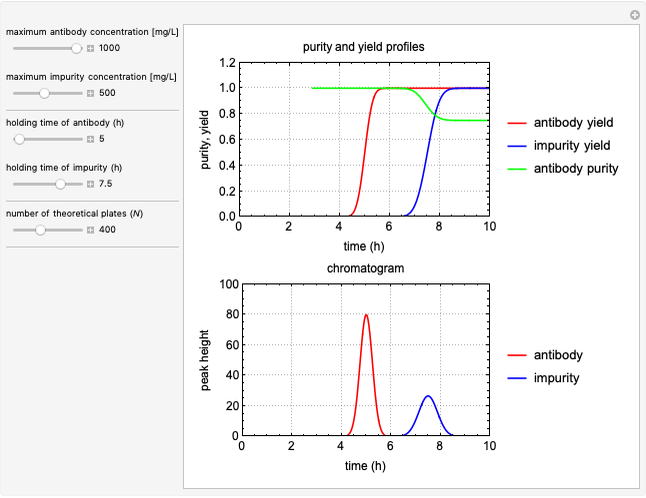
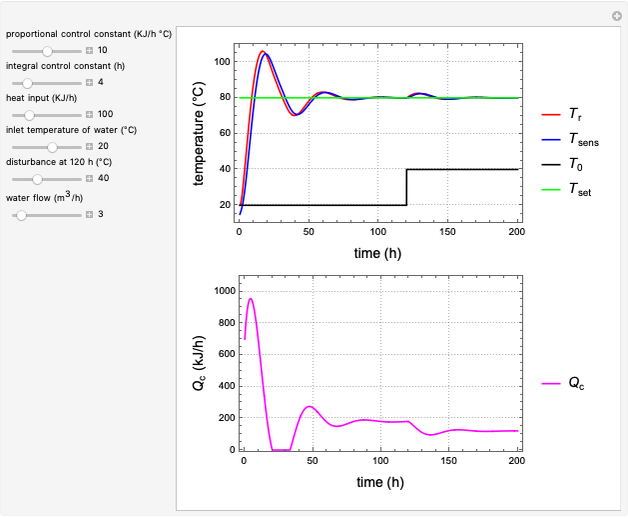
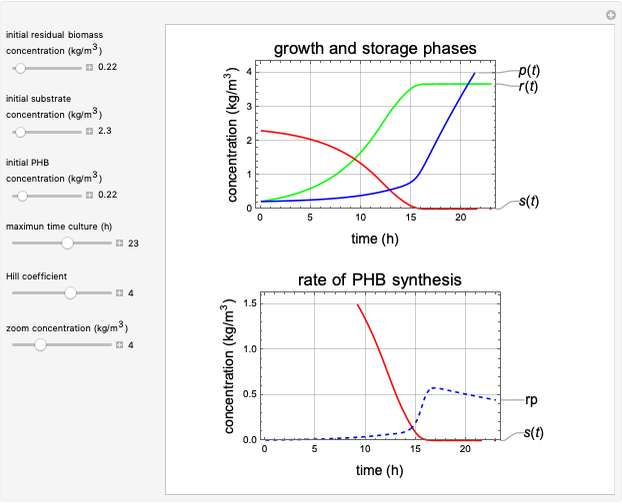
This Demonstration simulates cell growth in a chemostat limited by the oxygen mass transfer rate, which depends on the dissolved oxygen concentration. Cell growth is also inhibited by substrate concentration in the reactor; therefore a proportional control 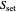 is used to maintain the substrate concentration below a critical value.
is used to maintain the substrate concentration below a critical value.
Contributed by: R. Ricardo Sánchez (August 2022)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Notation:
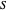 : substrate concentration (mg/L)
: substrate concentration (mg/L)
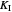 : inhibition constant (mg/L)
: inhibition constant (mg/L)
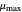 : maximum specific growth rate
: maximum specific growth rate 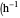 )
)
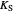 : saturation constant (mg/L)
: saturation constant (mg/L)
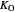 : oxygen saturation constant (mg/L)
: oxygen saturation constant (mg/L)
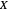 : biomass concentration (mg/L)
: biomass concentration (mg/L)
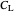 : dissolved oxygen concentration (mg/L)
: dissolved oxygen concentration (mg/L)
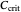 : critical dissolved oxygen concentration (mg/L)
: critical dissolved oxygen concentration (mg/L)
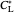 : dissolved oxygen saturation concentration or solubility of oxygen in the broth (mg/L)
: dissolved oxygen saturation concentration or solubility of oxygen in the broth (mg/L)
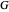 : gassing rate (L/min)
: gassing rate (L/min)
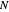 : stirrer speed
: stirrer speed 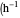 )
)
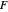 : feed substrate rate (L/h)
: feed substrate rate (L/h)
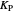 : proportional control constant
: proportional control constant 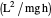
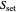 : inhibitory substrate concentration (mg/L)
: inhibitory substrate concentration (mg/L)
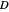 : dilution rate
: dilution rate 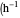 )
)
Kinetics
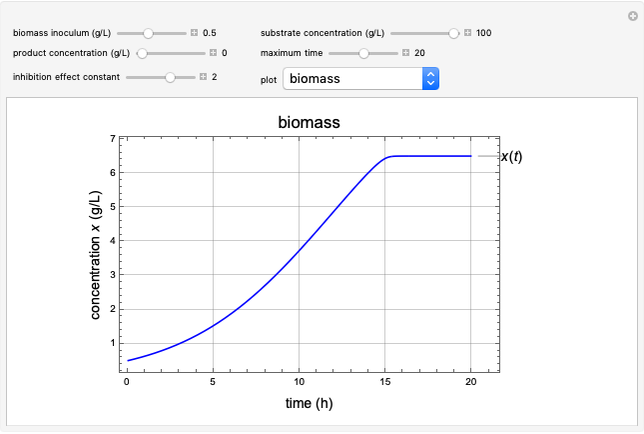
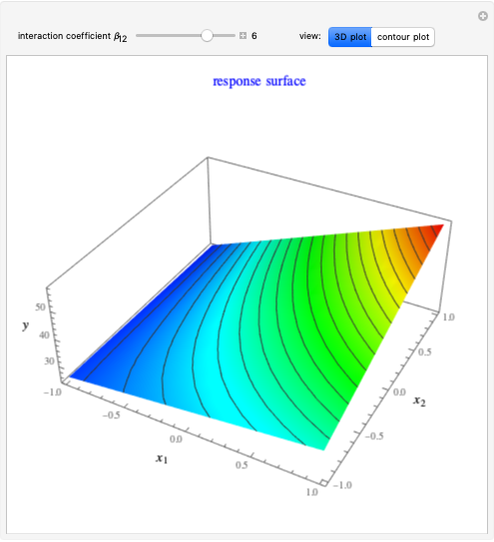
Inhibitory substrates at high concentrations reduce  , the specific growth rate, below that predicted by the Monod equation. The empirical inhibition function can be written:
, the specific growth rate, below that predicted by the Monod equation. The empirical inhibition function can be written:
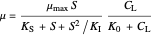 .
.
If substrate concentrations are low, the term 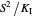 is smaller than
is smaller than 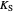 and
and 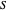 , and the inhibition function is represented by coupled Monod equations [2]. The plots show inhibition of the oxygen uptake and specific growth rates.
, and the inhibition function is represented by coupled Monod equations [2]. The plots show inhibition of the oxygen uptake and specific growth rates.
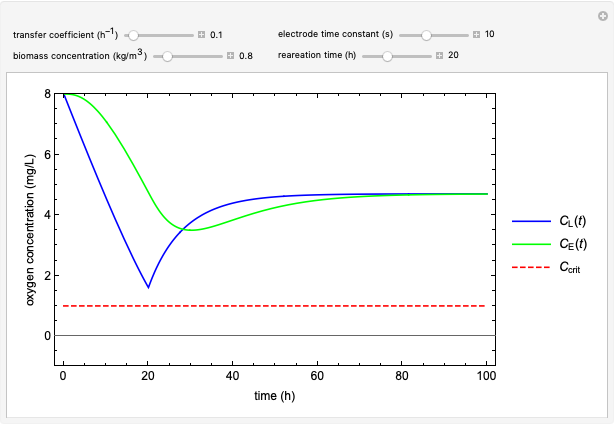
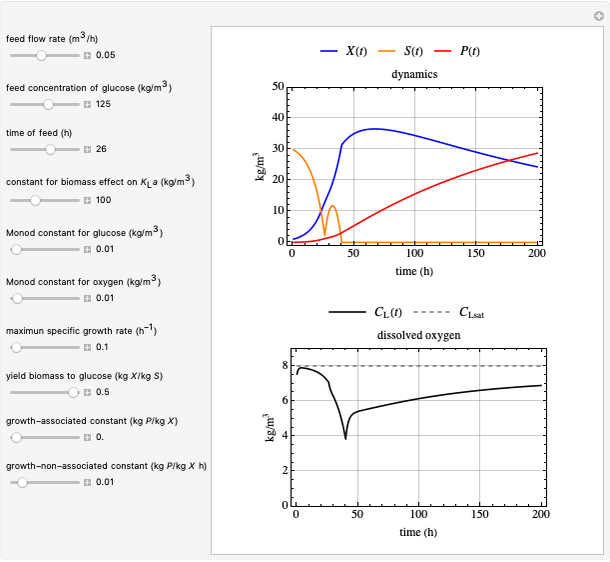
Oxygen Transfer
The oxygen mass transfer rate 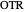 , could be represented by [1]:
, could be represented by [1]:
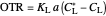 .
.
The transfer coefficient 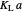 , varies with
, varies with 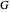 and
and 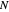 according to [2]:
according to [2]:
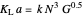 with
with 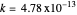 .
.
Control Process
Proportional control of the feed rate is based on the exit concentration using:
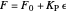
where  is the error, represented by
is the error, represented by 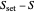 . If
. If 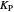 is zero, then
is zero, then 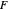 takes the value
takes the value 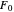 and the process runs out of control [2].
and the process runs out of control [2].
References
[1] P. M. Doran, Bioprocess Engineering Principles, Boston: Elsevier, 1995.
[2] I. J. Dunn, E. Heinzle, J. Ingham and J. E. Prvenosil, Biological Reaction Engineering, 2nd ed., Weinheim, Germany: VCH Verlagsgesellschaft mbH, 2003.
Permanent Citation