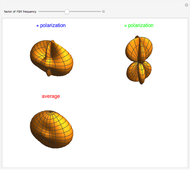
Spin-Weighted Spherical Harmonics

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
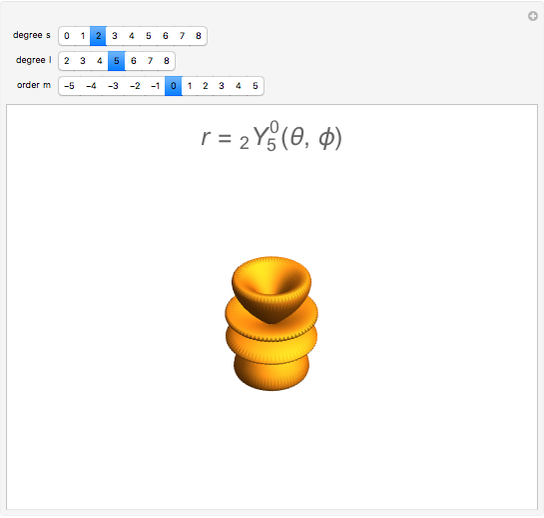
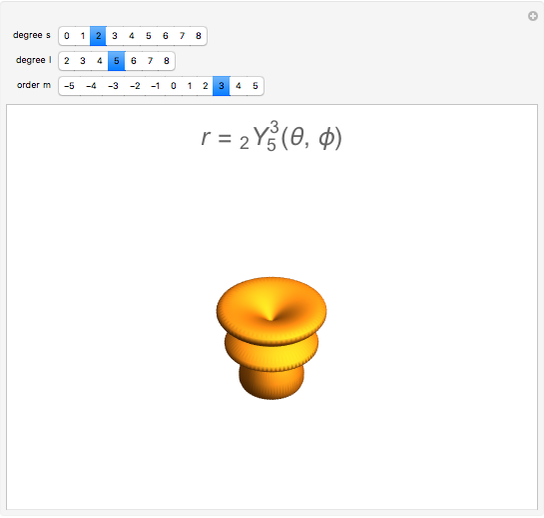
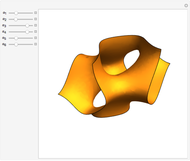
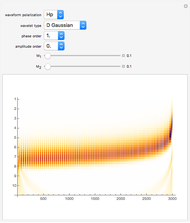
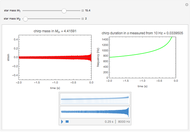
Spin-weighted spherical harmonics are generalizations of standard spherical harmonics and like them are complex functions on the sphere. The multipole expansions of an electromagnetic field can be expressed in terms of spin-weighted spherical harmonics. They are particularly important in the theory of gravitational waves.
Contributed by: Satya Mohapatra (March 2011)
Based on a program by: Stephen Wolfram
Open content licensed under CC BY-NC-SA
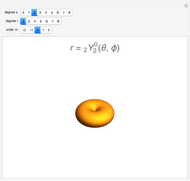
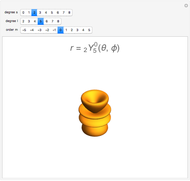
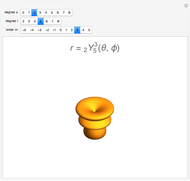
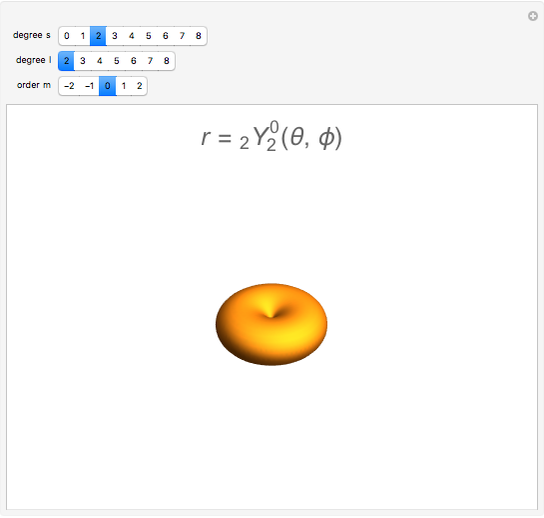
Snapshots
Details
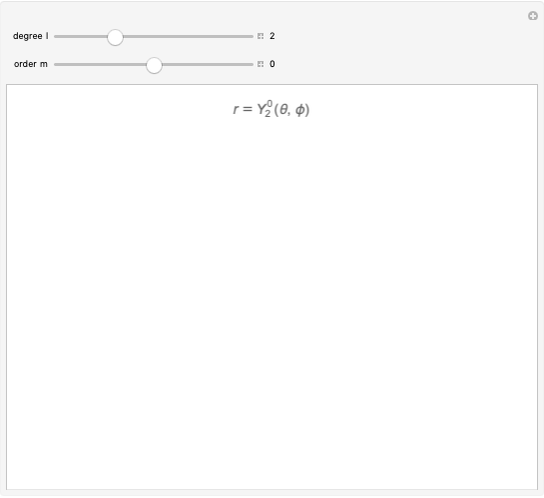
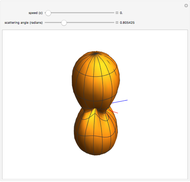
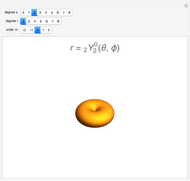
For spin weight  , the spin-weighted spherical harmonics become identical to the spherical harmonics. The case of spin weight
, the spin-weighted spherical harmonics become identical to the spherical harmonics. The case of spin weight  is important for describing gravitational waves. In this Demonstration you can choose different values of the spin weight
is important for describing gravitational waves. In this Demonstration you can choose different values of the spin weight  to see the angular distribution in space for different
to see the angular distribution in space for different  and
and  modes.
modes.
1) Introductory level reference:
http://en.wikipedia.org/wiki/Spin-weighted_spherical _harmonics
2) Intermediate level reference:
J. J. G. Scanio, "Spin-Weighted Spherical Harmonics and Electromagnetic Multipole Expansions," Am. J. Phys., 45(2), 1977 pp. 173–178.
3) Advanced level reference:
J. N. Goldberg, A. J. Macfarlane, E. T. Newman, F. Rohrlich, and E. C. G. Sudarshan, "Spin- Spherical Harmonics and
Spherical Harmonics and  ," J. Math. Phys., 8(11), 1967 pp. 2155–2161.
," J. Math. Phys., 8(11), 1967 pp. 2155–2161.
Permanent Citation