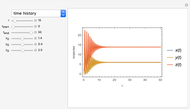
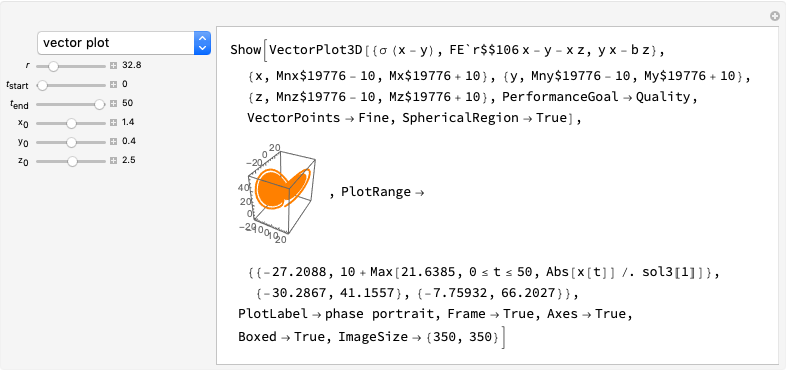
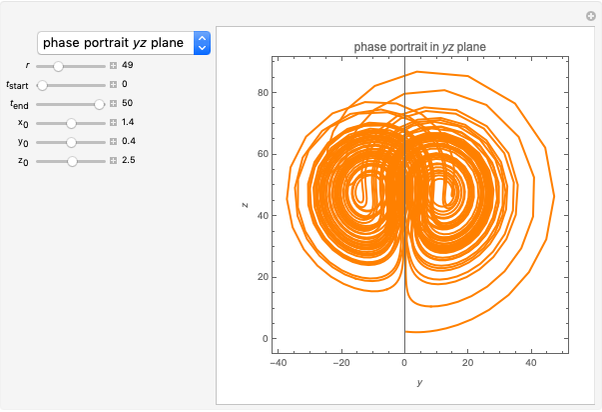
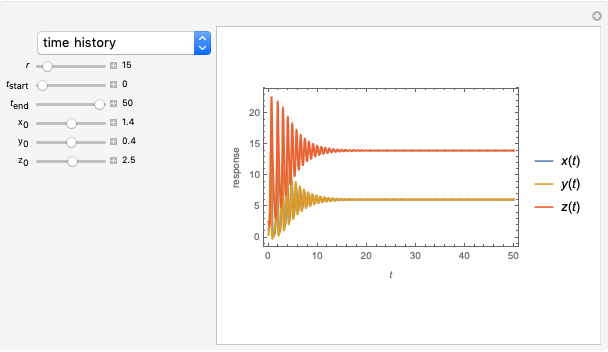
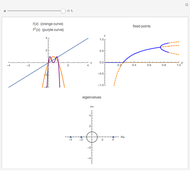
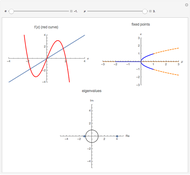
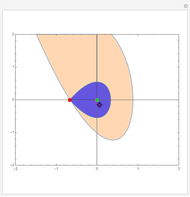
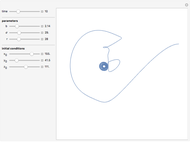
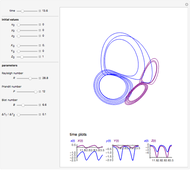
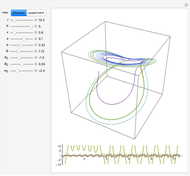
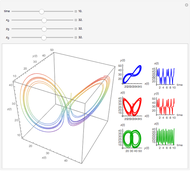
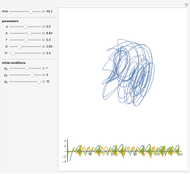
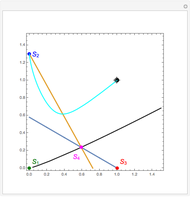
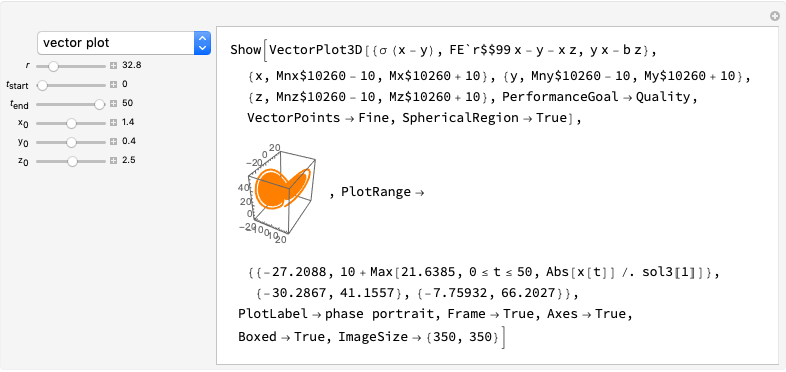
Stability of the Lorenz System
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
The Lorenz system is represented by three first-order differential equations (see [1, Section 9.0 and Exercise 9.3]):
[more]
Contributed by: Md Raf E Ul Shougat and Edmon Perkins (June 2020)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Reference
[1] S. H. Strogatz, Nonlinear Dynamics and Chaos: With Applications to Physics, Biology, Chemistry, and Engineering, 2nd ed., Boulder, CO: Westview Press, 2015.
Permanent Citation