Geometry of Two-Variable Associated Legendre Polynomials
Initializing live version

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
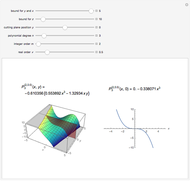
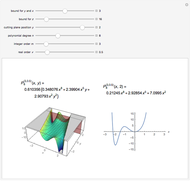
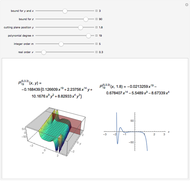
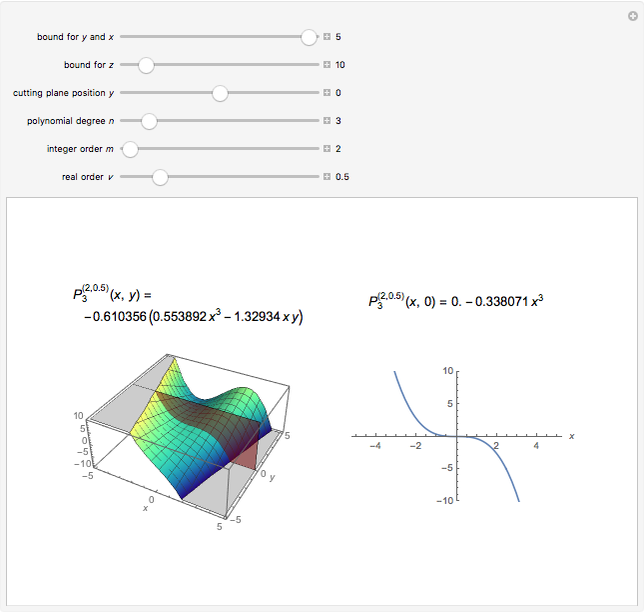
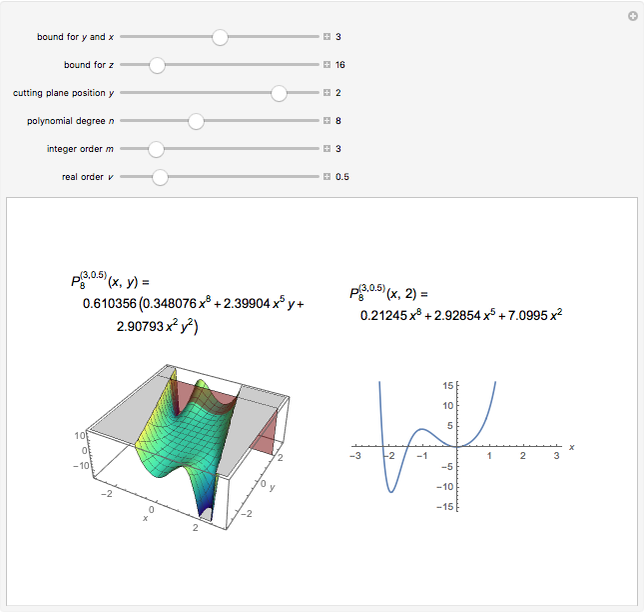
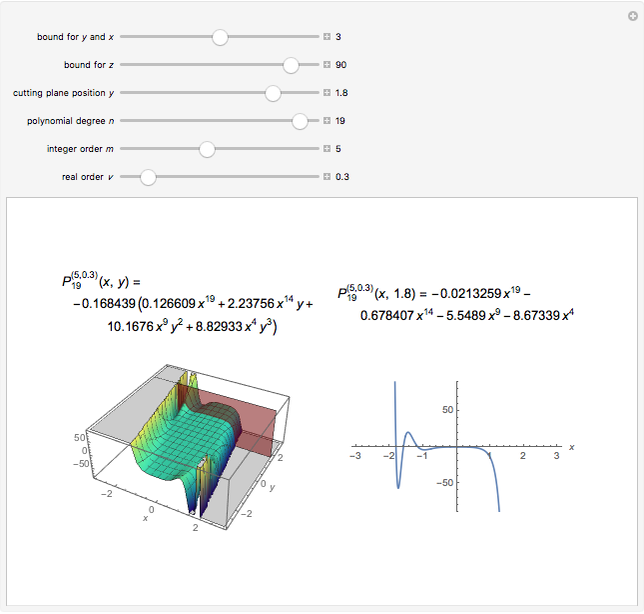
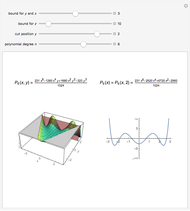
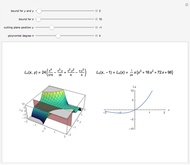
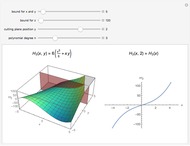
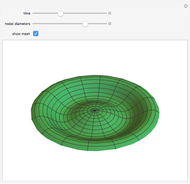
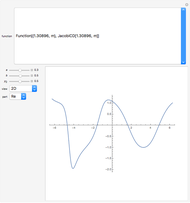
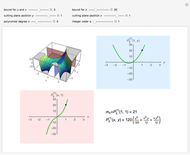
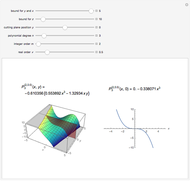
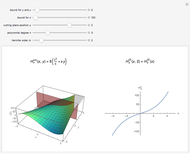
On the left is a three-dimensional plot of a Legendre polynomial in two variables 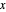 and
and 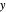 ; on the right is a two-dimensional plot of the surface cut by a plane perpendicular to the
; on the right is a two-dimensional plot of the surface cut by a plane perpendicular to the 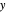 axis. The exponential operator transform is defined in the Details.
axis. The exponential operator transform is defined in the Details.
Contributed by: Marcello Artioli and Giuseppe Dattoli (February 2016)
Open content licensed under CC BY-NC-SA
Snapshots
Details
$FailedPermanent Citation