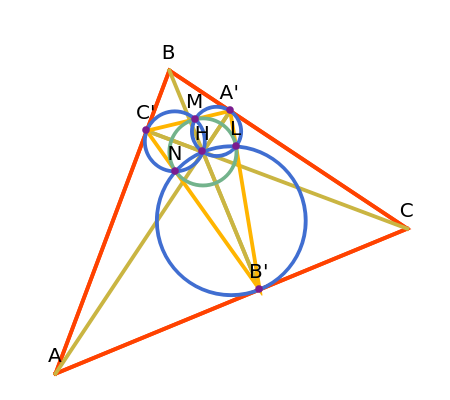
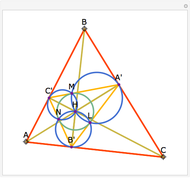
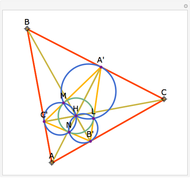
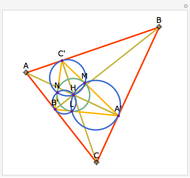
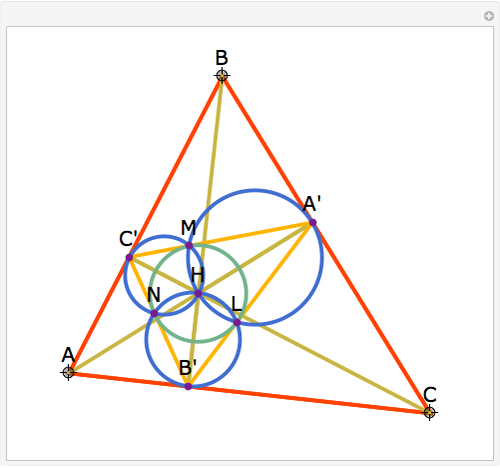
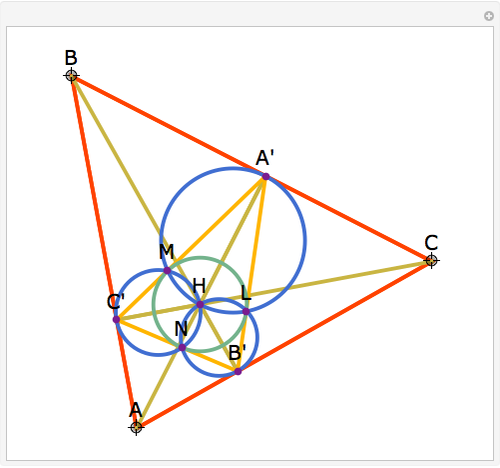
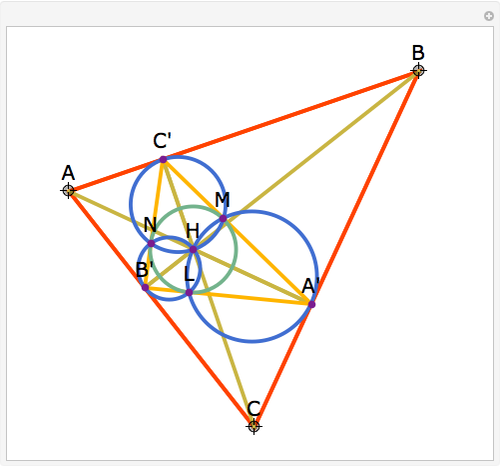
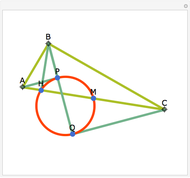
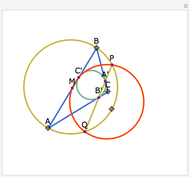
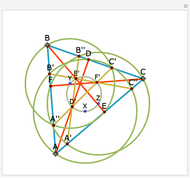
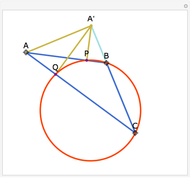
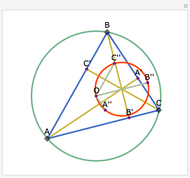
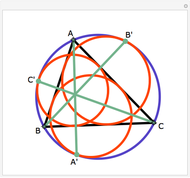
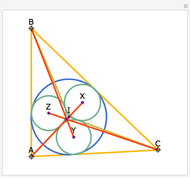
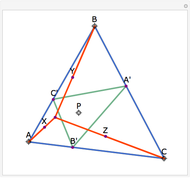
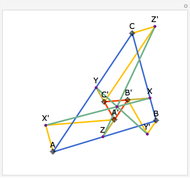
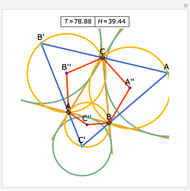
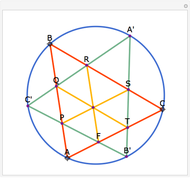
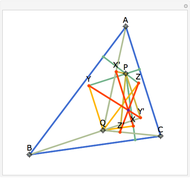
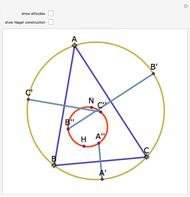
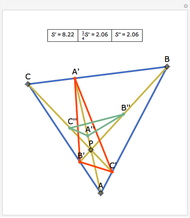
Let ABC be an acute triangle and H its orthocenter. Let A', B', and C' be the feet of the altitudes from A, B, and C. Let L, M, and N be the contact points of the orthic triangle with its incircle with L on A'B', M on A'C', and N on B'C'. Then A', M, H, L are concyclic as are B', L, H, N and C', M, H, N.