Visual Means Test

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
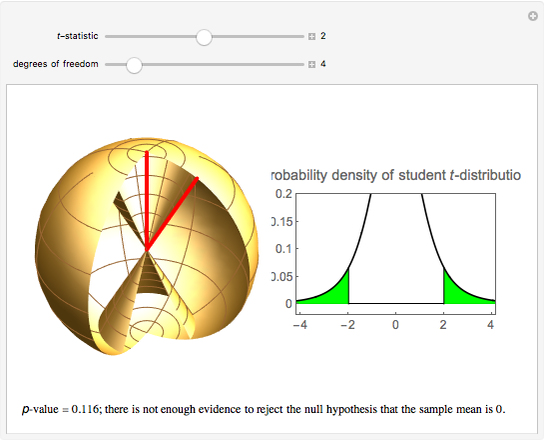
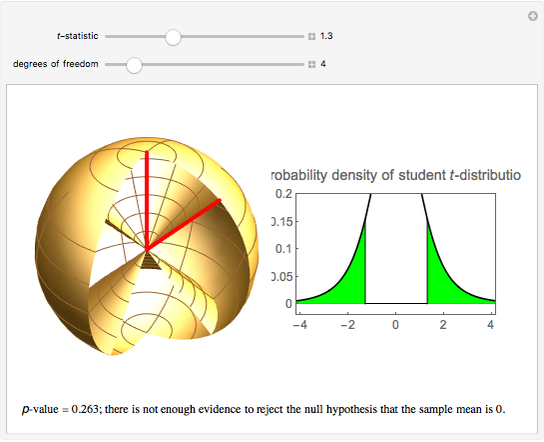
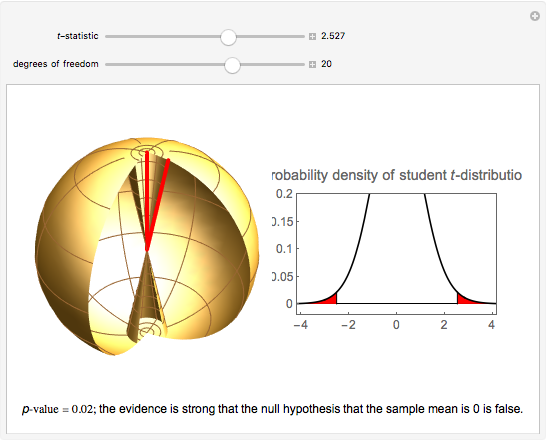
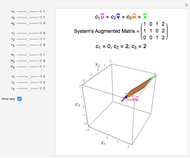
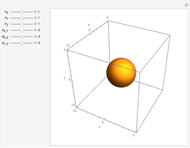
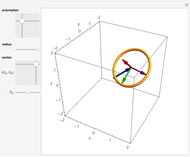
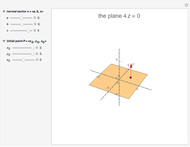
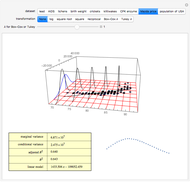
The set of vectors  in
in  -space of norm 1 forms a sphere. Any particular vector
-space of norm 1 forms a sphere. Any particular vector  , when rotated about the vector of means
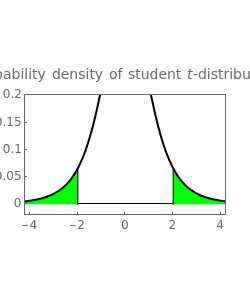
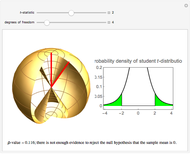
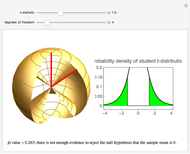
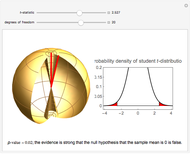
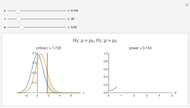
, when rotated about the vector of means  , defines a cone that intersects the sphere to form two spherical caps. The surface area of the caps divided by the surface area of the sphere equals the significance probability of the
, defines a cone that intersects the sphere to form two spherical caps. The surface area of the caps divided by the surface area of the sphere equals the significance probability of the  -test. Choose
-test. Choose  , the degrees of freedom;
, the degrees of freedom;  is then a vector in (
is then a vector in ( )-space that has the
)-space that has the  -value corresponding to the other control. Although the sample vector lives in
-value corresponding to the other control. Although the sample vector lives in  dimensions, a three-dimensional graphic is used to illustrate the cap-area phenomenon, with the cone angle determined by
dimensions, a three-dimensional graphic is used to illustrate the cap-area phenomenon, with the cone angle determined by  .
.
Contributed by: Karl W. Heiner and Stan Wagon (August 2011)
(SUNY New Paltz and Macalester College)
Open content licensed under CC BY-NC-SA
Snapshots
Details
Reference
[1] G. E. P. Box, W. G. Hunter, and J. S. Hunter, Statistics for Experimenters: An Introduction to Design, Data Analysis, and Model Building, 2nd ed., New York: Wiley–Interscience, 2005.
Permanent Citation