Zeno's Arrow Paradox and an Infinite Series

Requires a Wolfram Notebook System
Interact on desktop, mobile and cloud with the free Wolfram Player or other Wolfram Language products.
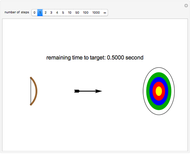
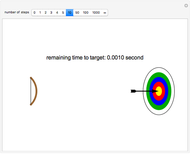
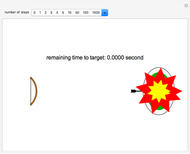
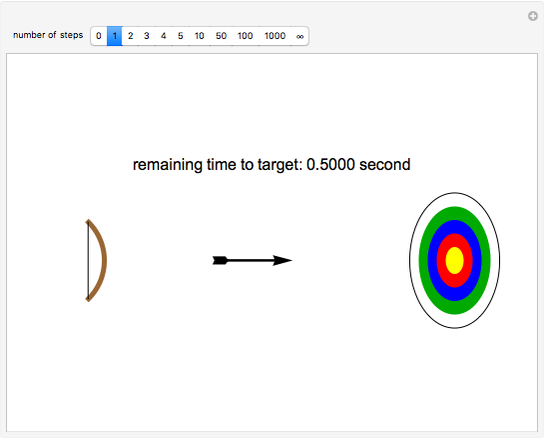
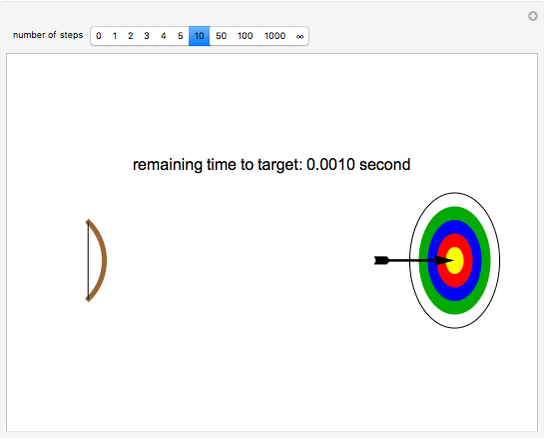
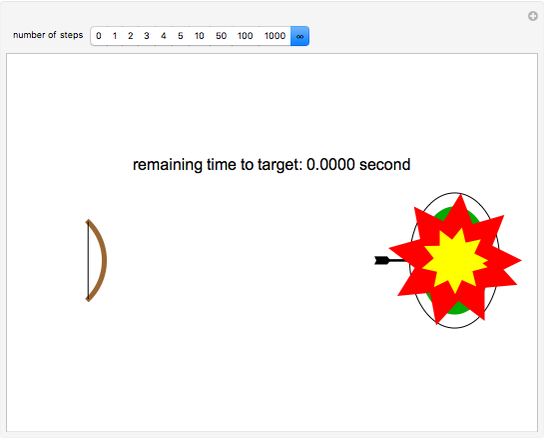
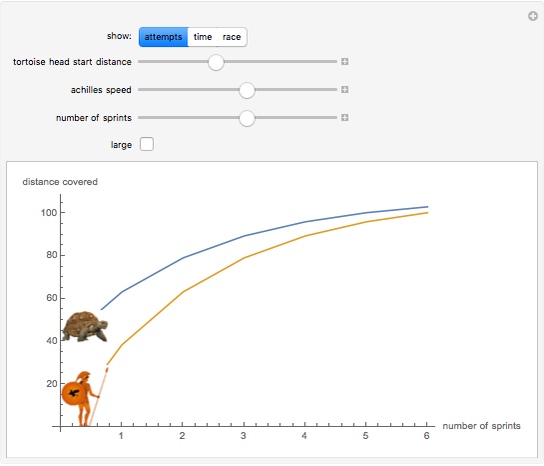
The Greek philosopher Zeno of Elea (ca. 490–430 BCE) is generally believed to have devised a group of paradoxes pertaining to motion over a finite distance during a finite interval of time. By Zeno's argument, an arrow shot at a target must first cover half the distance to the target, then half of the remaining distance, and so on. But this requires an infinite number of steps, so the arrow will never reach the target!
[more]
Contributed by: S. M. Blinder (December 2017)
Open content licensed under CC BY-NC-SA
Snapshots
Details
It is tempting to conclude that Zeno’s paradoxes were resolved after the introduction of the infinitesimal calculus and the understanding of convergent infinite series. In fact, the early twentieth-century philosopher C. S. Peirce claimed that "this ridiculous little catch presents no difficulty at all to a mind adequately trained in mathematics and logic."
However, some philosophical fine points are still under discussion [1], pertaining mainly to whether space and time are infinitely divisible, and to the exact meanings of a point in space and an instant in time. Recall that matter turned out not to be infinitely divisible.
References
[1] N. Huggett. "Zeno's Paradoxes." The Stanford Encyclopedia of Philosophy. (Dec 11, 2017) plato.stanford.edu/entries/paradox-zeno.
[2] Wikipedia. "Zeno's Paradoxes." (Dec 11, 2017) en.wikipedia.org/wiki/Zeno%27 s_paradoxes.
Permanent Citation